(Department of Mathematics,Inner Mongolia Normal University,Huhhote 010022,China)

§1. Introduction and main results

Letbe the weighted Orlicz spaces,the weight function isForits Orlicz norm is de fined as[1]

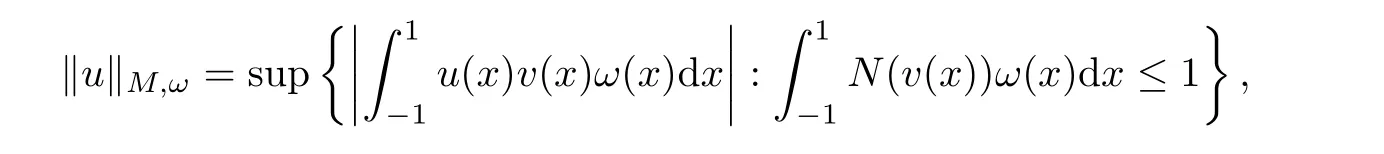
or

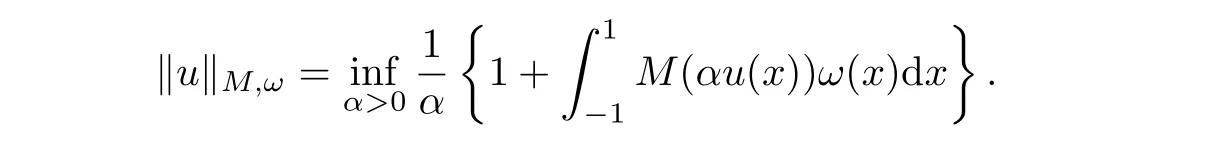
The Luxemburg norm

is equivalent to the Orlicz norm asholds true.

denotes the modulus of continuity of f in Orlicz spaces,that is

Sun Zhiling[2]discussed the approximation of Nevai-Kantorovich rational interpolation operator in weighted Orlicz spaces.In 2009,Gu Chunhe[3]studied the approximation theorem of the first kind of modi fied Vertesi-Kantorovich operator in Orlicz spaces which is formed by the Lagrange interpolation basis function of the first kind of Chebyshev polynomial.In 2010,Feng Yue[4]studied the approximation theorem of shepard-Kantorovich operator in Orlicz space.In the second chapter of[5],not only the de finition of Shepard-Durrmeyer,Vertesi-Durrmeyer and Nevai-Durrmeyer rational interpolation operators are given by Cheng Wentao,but also the approximation theorems of three kinds of operators in Lp(1≤p<∞)spaces were proved in 2012.Therefore,on the basis of the literature[5],we study the approximation problems of two kinds of Durrmeyer rational interpolation operators inspaces.

Let η(x)be a generalized smooth Jacobi weight function de fined by

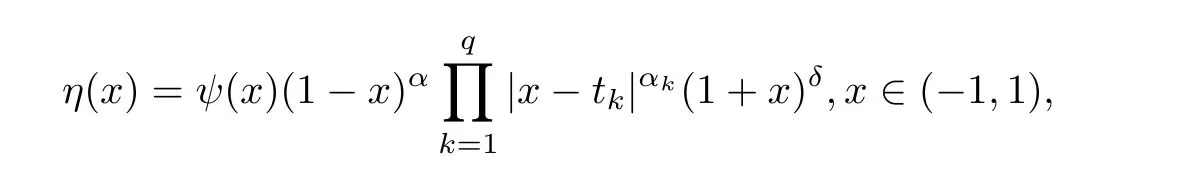
where ?1 ?1,k=1,2,···,q and 0< ψ ∈ LipMλ.Denote bythe corresponding system of orthogonal polynomials associated with the weight function η(x),andthe zero of Pn(x).

For anythe modi fied Nevai-Durrmeyer operator is de fined as

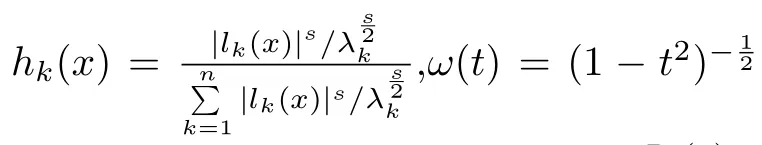
For anyde fine the modi fied Shepard-Durrmeyer operators as

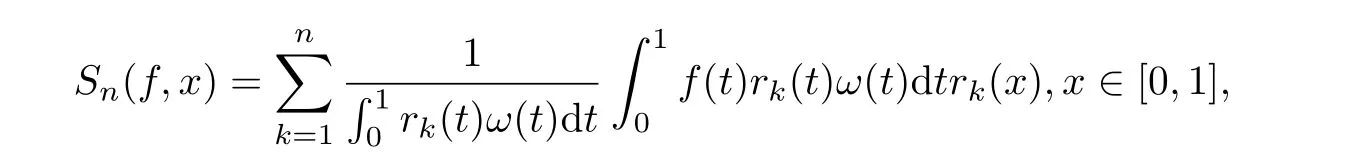
Theorem 1.1Let,then

Write

Here and throughout the whole paper,we use Csto indicate a positive constant depending only on s,Cλ,to indicate a positive constant depending only on λ,and C is an absolute positive constant,their values may vary in de ff erent occurences even in the same line.

§2. Preliminaries

In order to proof theorem,we need the following lemmas.

Let x0=1,xn+1= ?1,also xk,k=1,2,···,n,be the zeros of pn(u).Set xk=cosθk,then θk∈ [0,π],k=0,1,···,n+1 and

Lemma 2.1[5,6]For any x∈[xj+1,xj],0≤j≤n,0≤k≤n,we have

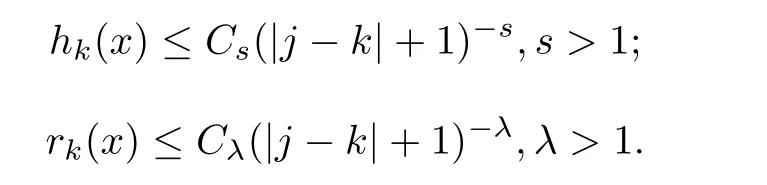
Lemma 2.2[5,6]Let x=cosθ,θ∈ [0,π],we have

where

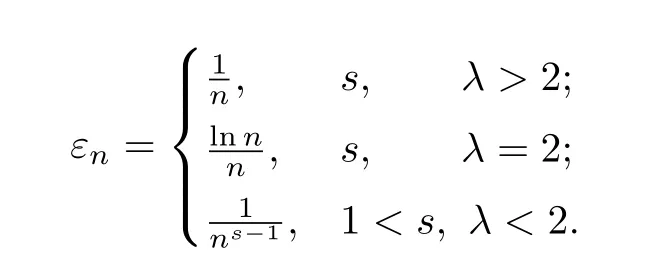
Lemma 2.3For s,λ >1,Dn(f,x),Sn(f,x)are positive bounded linear operators in,that is,for anywe have

Let g(θ)=f(cosθ),by using Jackson operators,we can easily obtain the following lemma.

Lemma 2.4[3]Letbe an even function,then there exists an even trigonometric polynomial TN(θ)with degree ≤ N,such that

Lemma 2.5Let PN(x)be a polynomial whose order is less than or equal to N,and TN(θ)=PN(cosθ),then

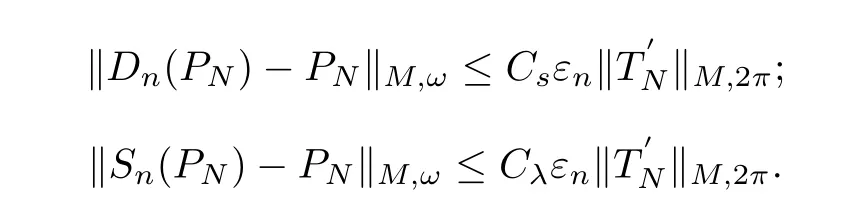
ProofLet x=cosθ,t=cosυ,θ,υ ∈ [0,π],because of Dn(f,x)=1,we have

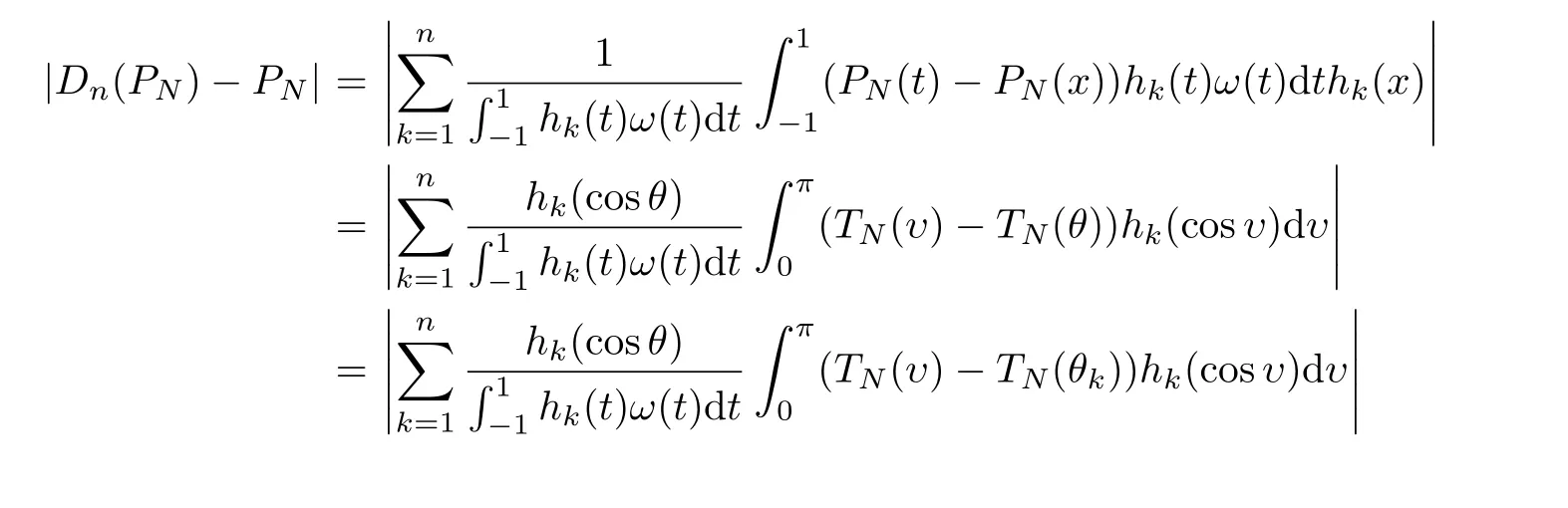
whereare the Hardy-Littlewood extremal maximum function of

By lemma 2.3,we obtain

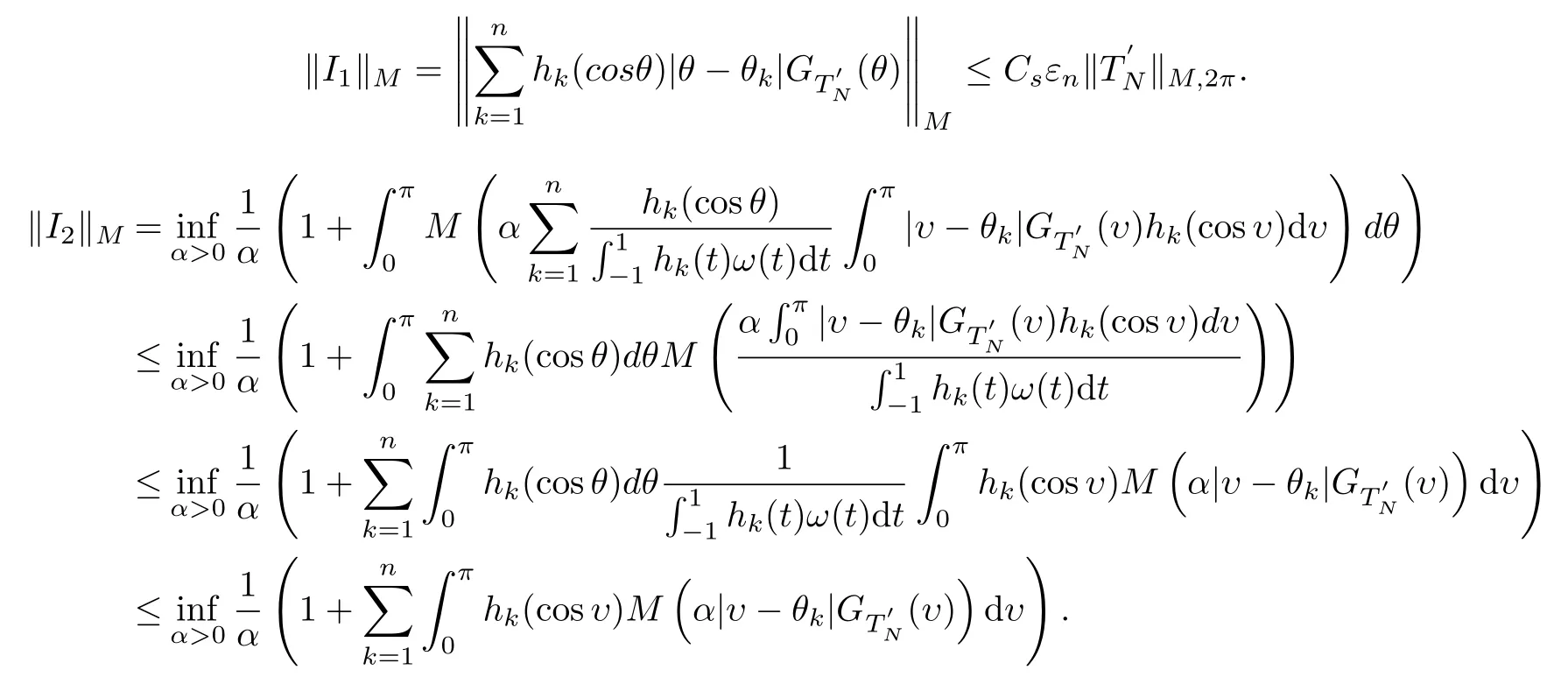
There is another constant C,satis fiesby lemma 2.3,we obtain

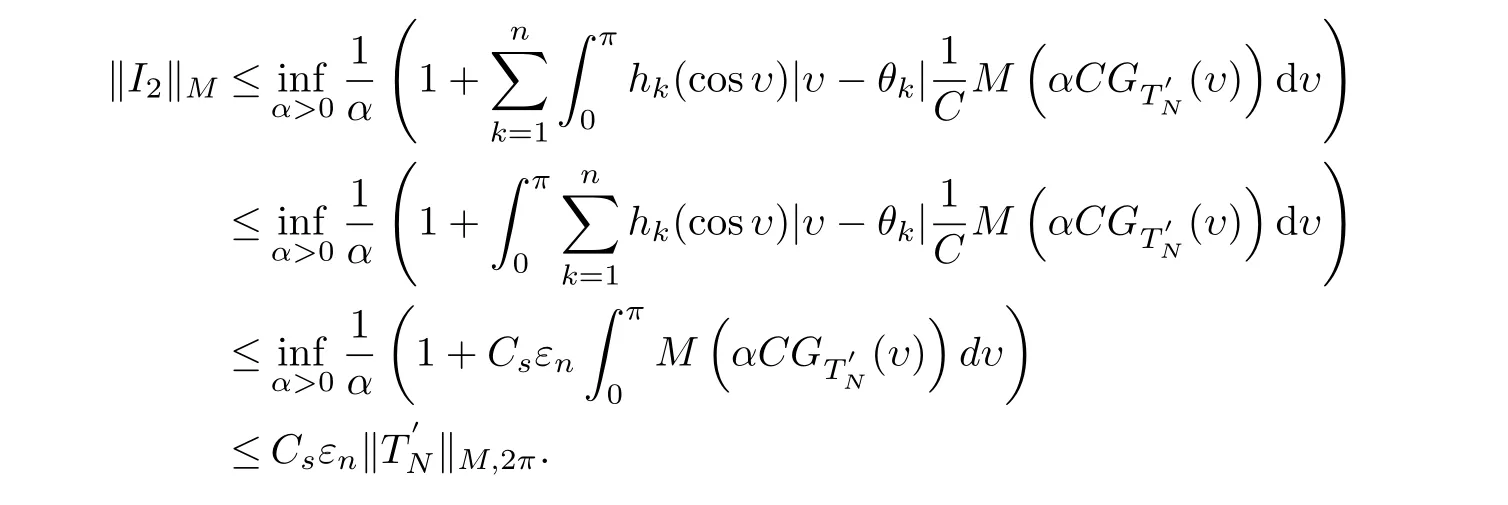
Similarly available

§3.Proof the Theorem

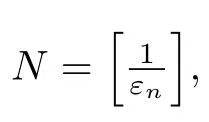
Similarly available

[1]WU Cong-xin,WANG Ting-fu.Orlicz space and its application[M].Harbin:Heilongjiang Science and Technology Press,1983.

[2]SUN Zhi-ling.Approximation theorems of modi fied Nevai operators in Orlicz spaces[J].Journal of Inner Mongolia University for the Nationalities(NATURAL SCIENCE EDITION),2007,22(2):121-124.

[3]GU Chun-he.Study on some approximation problems in Orlicz spaces[D].Hohhot:School of Mathematical Sciences,Inner Mongolia Normal University,2009.

[4]FENG Yue.Approximation equivalence theorem of Shepard operator in Orlicz spaces[J].journal of inner mongolia normal university2010,39(6):565-568.

[5]CHENG Wen-tao.Approximation theorems of modi fied rational operators[D].Zhejiang:School of mathematics,Zhejiang Sci-Tech University,2012.

[6]ZHOU Guan-zhen.Approximation of a kind of Nevai-Durrmeyer operators in spaces[J].Analysis in Theory and Applications2005,21(3):294-300.

[7]A.-R.K.RAMAZANOV.On approximation by polynomials and rational functions in Orlicz spaces[J].Analysis Mathematica,1984,10:117-132.