Tengteng Zheng · Liuxiao Zou · Yingcheng Hu

Abstract A wood-based X-type lattice sandwich structure was manufactured by insertion-glue method. The birch was used as core, and Oriented Strand Board was used as panel of the sandwich structure. The short beam shear properties and the failure modes of the wood-based X-type lattice sandwich structure with different core direction (vertical and parallel), unit specification (120 mm × 60 mm and 60 mm × 60 mm), core size (50 mm and 60 mm), and drilling depth (9 mm and 12 mm) were investigated by a short beam shear test and the establishment of a theoretical model to study the equivalent shear modulus and deflection response of the X-type lattice sandwich structure. Results from the short beam shear test and the theoretical model showed that the failure modes of the wood-based X-type lattice sandwich structure were mainly the wrinkling and crushing of the panels under three-point bending load. The experimental values of deflection response of various type specimens were higher than the theoretical values of them. For the core direction of parallel, the smaller the unit specification is, the shorter the core size is, and the deeper the drilling depth is, the greater the short beam shear properties of the wood-based X-type lattice sandwich structure is.

Keywords X-type · Lattice sandwich structure · Failure modes · Short beam shear properties · Theoretical model

Introduction

Wood is a high-quality material, compared with other building materials or metal, it has outstanding thermal insulation, high strength-weight ratio, sound absorption and vibration isolation, comfortable texture, and offers environmental protection. These characteristics make wood and wood products more and more widely used in the field of wood construction (Elias et al. 2018; Marius et al. 2018). However, owing to the ongoing substantial changes in the global forest resource structure and heightened concerns regarding anthropogenic climate change. In particular, the high-quality wood resources in Asia are rapidly decreasing, while the demand for wood products and the environmental consciousness are constantly growing. Low-quality timber such as fast-growing and high-yield plantation has become the main source of commercial timber in Asia (Katsigris et al. 2004). Therefore, the emphasis of processing and utilization in the future is the in-depth development and high value-added utilization of advanced technologies of artificial fast-growing forests, lowquality wood, processing residues and biomass materials (Min and Zhi 2004; Wang et al. 2019; Nguyen et al. 2019; Zhang et al. 2018; Yuan et al. 2013). With the increasing awareness ofenvironmental issues, the research of biomass composites based on renewable resources has become more and more popular (Li and Hu 2019; Hao et al. 2017; Qin et al. 2018; Li et al. 2018, 2019a, b; Lu et al. 2014).

The lattice sandwich structure is a kind of structure with periodic cell arrangement and cell bar system satisfying tensile dominant configuration, which was originally proposed by Evans and co-workers (Evans et al. 2001; Deshpande and Fleck 2001). At present, most common lattice sandwich structures are prepared from metal and carbon fiber materials (Lim et al. 2009; Lee et al. 2014), which are used in the fields of transportation and aerospace. Fan et al. ( 2006, 2007) studied the mechanical properties of Kagome lattices reinforced by carbon fibers, Results show that the carbon fiber reinforced lattice grids are much stiffer and stronger than foams and honeycombs. Wang et al. ( 2014, 2018) studied the mechanical properties of the 3-D lattice sandwich structure. Results show that the mechanical properties of the X-type lattice sandwich structure are better than the other sandwich structures. However, the lattice sandwich structure made of metal has high material density and low safety at the junction of core and panel. In addition, due to the high quality of lattice sandwich structure made of metal and poor shock and energy absorption performance, it is diffi cult to apply in the field of architecture. For high-rise buildings, the reduction of the upper mass can reduce the pressure of the bottom structure and materials, and improve the service life, which is well reflected in the wood-structured houses. Because of its small mass, wood-structured buildings absorb relatively small seismic forces during earthquakes. Wood structure with superior toughness has strong resistance to instantaneous impact load and periodic fatigue damage, and can absorb and dissipate energy. Filling some functional materials in the layer of wood-based lattice sandwich structure can realize the integration of structure and function (Fam and Sharaf 2010).

However, the study of the lattice sandwich structure mainly composed of wood or wood composite materials is rare, and its application as a wood engineering material in wood construction is worth exploring. Li et al. ( 2018, 2019a, b) studied the compressive behavior of the bio-based 2-D lattice structure. Results show that the bio-based 2-D lattice structure has superior behavior than the other bio materials. Qin et al. ( 2019) investigated the effects of the core configuration on the out-of-plane compressive behavior of a woodbased 2-D straight column lattice truss sandwich structure. The compressive modulus of the structure reinforced with birch sheeting is higher than that without reinforcement. Jin et al. ( 2015) studied the mechanical properties of 2-D lattice sandwich structure with different panel materials. The compression property of wood-based lattice sandwich structure demonstrate affects the safety of wood structure and bending property of lattice sandwich structure. Zheng et al. ( 2020a, b) studied the mechanical properties and failure modes of the wood-based X-type lattice sandwich structure. Their results showed that the wood-based lattice sandwich structure has the characteristics of light weight and high strength, and the mechanical properties of double X-type lattice sandwich structure are better than that of X-type lattice sandwich structure. In a sandwich structure, the strong and stiff skins carry most of the in-plane and bending loads while the core mainly bears the transverse shear and normal loads (He and Hu 2008). In particular, the shear strength of the core is a critical parameter and is an important consideration when designing the wood-based lattice sandwich structures (Manalo et al. 2010; Manalo 2013; Sideridis and Papadopoulus 2004 ). These important aspects have to be addressed in order to advance the use of the wood-based lattice sandwich structures in the field of wood construction.

From the above, it can be seen that the light lattice sandwich structure has the characteristics of high weight height ratio and high rigidity. Therefore, based on the design concept of high porosity and large space, the use of wood materials to prepare the lattice sandwich structure provides reference for the production of wooden material shear walls, and it may have certain practical significance for improving the effi cient use of wood resources.

Materials and methods

Materials

The raw materials required for the wood-based X-type lattice sandwich structure specimens were panel materials, core materials, and adhesive. The Oriented Strand Board (OSB, GERMANY, E0, Oriental Port International Wood Co. LTD, China) was used as the panel material, its thickness is 15 mm, and density is 0.61 g cm?3. The Oriented Strand Board was made of the European pine wood, and the shavings were connected by isocyanate with non-formaldehyde. The birch (wooden dowel pins, Tengzhan Wood Industry Co. LTD, Harbin, China) was used as the core material, it was made of board of the Asian white birch. The birch used in this experiment has a diameter of 8 mm and a density of 6.26 g cm?3, and it is produced in Harbin, Heilongjiang Province, China. The adhesive was a mixture ofepoxy resin (WSR6101 E-44, Star Synthetic Materials Co. LTD, Nantong, China) and polyamide resin (Low molecular-650-, Danbao Resin Co. LTD, Chuzhou, China), and the quality ratio ofepoxy resin and polyamide resin was 1:1.

Unit cell design

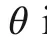
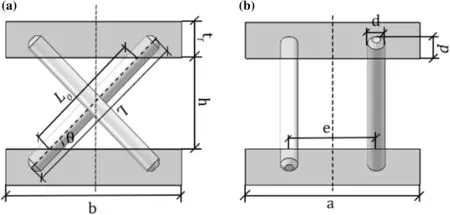
Fig.1 The unit cell schematic of the X-type lattice sandwich structure a main view and b side view

The relative density of the wood-based X-type lattice sandwich structure (the ratio of the density of the lattice sandwich cores to the solid material density of the constituent lattice cells) can be expressed in Eq. 1:

We explored the short beam shear properties of the wood-based X-type lattice sandwich structure by designing eight types of X-type lattice sandwich structures. The effects of core direction (vertical and parallel), unit specification (120 mm × 60 mm and 60 mm × 60 mm), core size (50 mm and 60 mm), and drilling depth (9 mm and 12 mm) were determined. The parameters of the X-type lattice sandwich structures are shown in Table 1.

BS1 and BS4 are specimens of the same type. BS3, BS6 and BS8 are specimens of the same type; In order to compare the influences of different variables on the short beam shear properties of the sandwich structure.

Fabrication

The process of manufacturing the X-type lattice sandwich structure as follows: (a) The width and length of the panels of the wood-based lattice sandwich structure were cut according to the relevant standards of sandwich structure (GB/T 1456-2005) and the cores were optimized according to quality of them. The panels were drilled with a drilling device capable of automatic positioning. The Angle between the drill bit and the panel is 45°. (b) The appropriate amount of mixture ofepoxy resin and curing agent was applied in the hole of the drilled panels. (c) The core with the same diameter as the borehole diameter was inserted into the hole of the lower panel, and the other end of the core is inserted into the hole of the upper panel. At this time, the specimen has been assembly completed. (d) The appropriate pressure was applied on the X-type lattice sandwich structure to make sure the upper and lower panels were parallel. The specimen is ready for testing after standing for 72 h. There were 8 specimens ofeach type, resulting in a total of 64 specimens.

Mechanical properties of core materials

The mechanical property tests of birch round rod were carried out based on the recommendations of ISO640-02. The results are shown in Table 2.

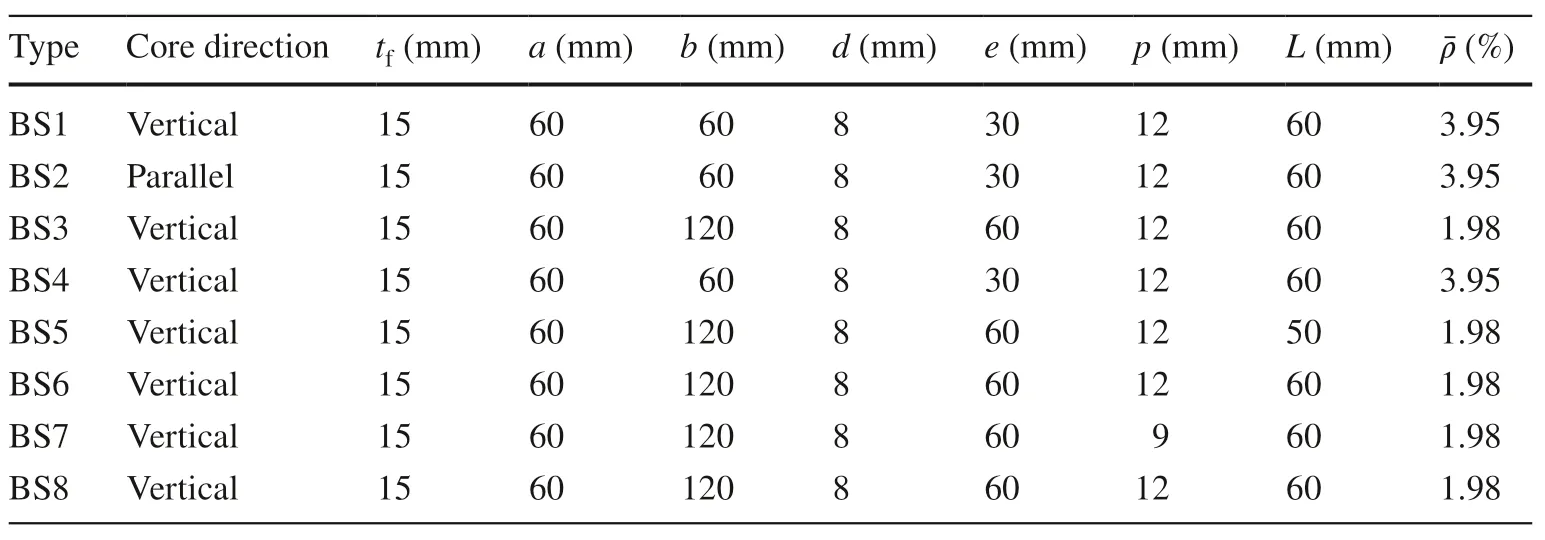
Table 1 Cell size design of the X-type lattice sandwich structure

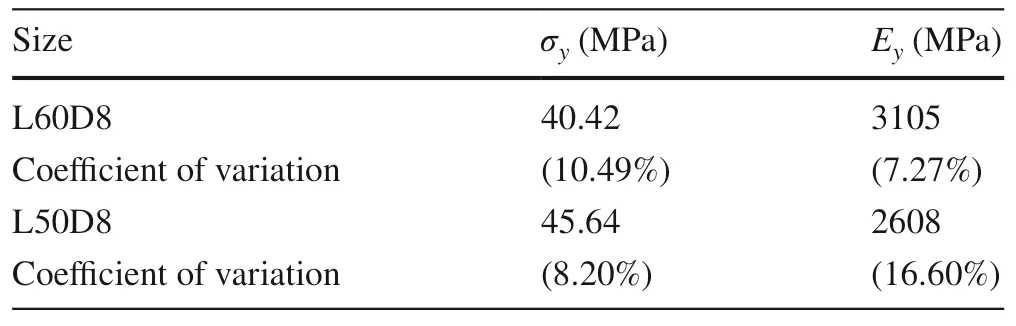
Table 2 The mechanical property of the core material

Short beam shear test

Short beam shear experiments of the wood-based X-type lattice sandwich structure were performed at a loading rate of 1 mm/min in accordance with GB/T 1456-2005. The experimental temperature was 20 ± 2 °C and the humidity was 65% ± 5%. The diameter of cylindrical loading roll with pressure head was 30 mm. The length of the specimen of short beam shear is 240 mm, and the span of the specimen is 120 mm.

The following equation was used to calculate the shear stress of the core:

where P is the midspan load, K is the dimensionless number, H is the width, and b is the thickness of the short beam shear test specimen. The thickness of the panel is tf.

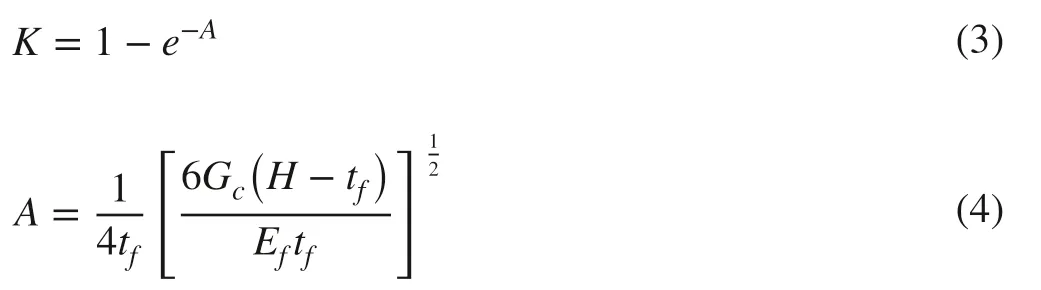
where Efis the elastic modulus of panel, A is the dimensionless number, Gcis the shear modulus of core, and e is the base of natural logs.

The formula for calculating the stress of the panel was as follows:

The following equation was used to calculate the bending stiff ness of the sandwich structure:

where a is the length ofextended arm,ΔP is the load increment value of load–deflection curve in initial elastic stage, and f1is the incremental value of the deflection of the extended part corresponding toΔP in the elastic stage.

The formula for calculating the elastic modulus of panel was as follows:

where J is the inertial moment of the sandwich structure.

The following equation was used to calculate the shear stiff ness of sandwich structure:

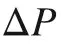
The following equation was used to calculate the shear modulus of core:

Analytical model

The homogenization method not only simplifies the calculation, but also has better accuracy. Therefore, it is appropriate to study the stiff ness of the X-type lattice sandwich structure by means of homogenization method. In this paper, the short beam shear properties of the core of the wood-based X-type lattice sandwich structure were studied by homogenization method, and the deflection response of the structure in the elastic stage was calculated and compared with the experimental results. Under three-point bending load, the upper and lower panels of the X-type lattice sandwich structure mainly bear bending load, while the cores mainly bear shear load, in which the lower panel is under tension load and the upper panel is under compression load. The X-type lattice sandwich structure diagram under three-point bending load was shown in Fig.2.

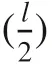
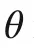
Fig.2 The X-type lattice sandwich structure diagram under threepoint bending load

The equivalent parameter of short beam shear

Because the wood-based X-type lattice sandwich structure is a typical periodic structure, the unit cell of the structure can be taken out for stress analysis to derive its transverse shear modulus. The transverse shear modulus of core of the X-type lattice sandwich structure can be expressed by Eq. 11:

The cores of the X-type lattice sandwich structure mainly bear axial compression load. The transverse shear force and transverse shear strain of core can be expressed by Eqs. 12 and 13:

The transverse shear modulus of the core can be expressed by Eq. 15:

Deflection response

where Deqis the equivalent bending stiff ness of the X-type lattice sandwich structure, and ( AG)eqis the equivalent shear stiff ness of the X-type lattice sandwich structure.

The equivalent bending stiff ness of the X-type lattice sandwich structure can be expressed by Eq. 17:

where D f is the bending stiff ness of panel relative to its neutral axis, D 0 is the bending stiff ness caused by shifting axle of panel, and D c is the bending stiff ness of core.

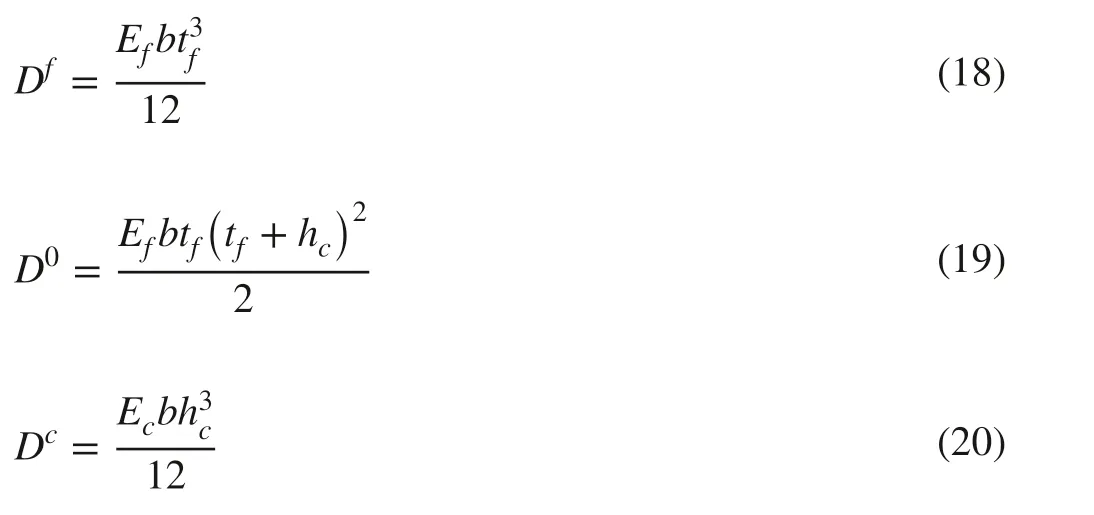
The shear deformation of the X-type lattice sandwich structure was shown in Fig.3. The relationship between shear deformation of the sandwich structure and transverse shear strain of the core can be expressed by Eq. 21:

The transverse shear force of the X-type lattice sandwich structure can be expressed as follows:

The equivalent shear stiff ness of the X-type lattice sandwich structure is:

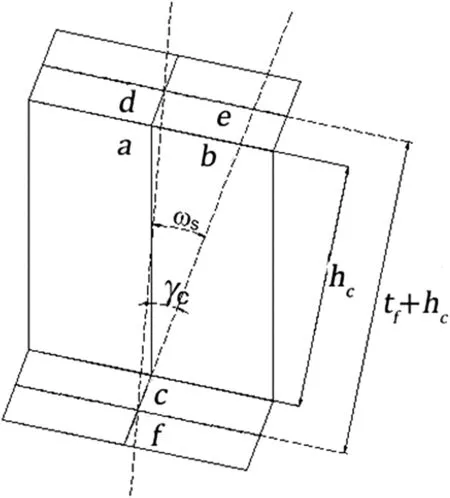
Fig.3 The shear deformation of the X-type lattice sandwich structure

The deflection response of the X-type lattice sandwich structure is:

Results and discussion

Failure modes and short beam shear properties

The short beam shear properties and the failure modes of the wood-based X-type lattice sandwich structure with different core direction (BS1 and BS2), unit specification (BS3 and BS4), core size (BS5 and BS6), and drilling depth (BS7 and BS8) were investigated. The load–displacement curves of the X-type lattice sandwich structure are shown in Fig.4.

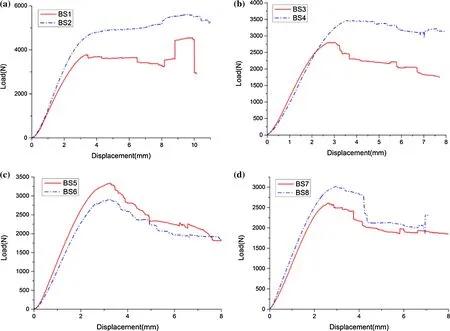
Fig.4 The load–displacement curves of the X-type lattice sandwich structure with different a different core direction, b different unit specification, c different core size, and d different drilling depth

The failure modes of the sandwich structure are shown in Fig.5, and the shear stress and modulus of core of the sandwich structure are shown in Fig.6.

Different core direction

The load–displacement curves of type BS1 and BS2 specimens are shown in Fig.4 a. The curves are divided into two stages: the linear elastic stage and the slow decreasing curve growth rate stage. The curve shows a good linear relationship in the linear elastic stage. With the increase of displacement, the rate of load increase decreases until the peak load is reached. After crossing the peak load, the curve does not decline rapidly, but slowly, and then there is a long platform area. The shear stress and modulus of core of type BS2 specimen are 30.19 and 29.03% higher than those of type BS1 specimen, respectively (Fig.6). This indicates that the core direction has an effect on the bending performance and shear performance of the wood-based X-type lattice sandwich structure. The binding force of the panel on the core of type BS2 specimen is larger than that of type BS2 specimen. The failure modes of the type BS1 and BS2 specimens are mainly the failure of the upper and lower panels (Fig.5 a, b), which indicates that the upper and lower panels cannot resist the increasing vertical load.

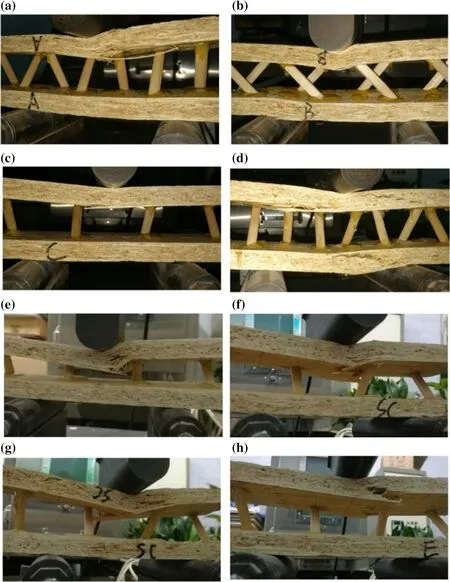
Fig.5 The failure modes of the X-type lattice sandwich structure with a type BS1, b type BS2, c type BS3, d type BS4, e type BS5, f type BS6, g type BS7, h type BS8 specimens

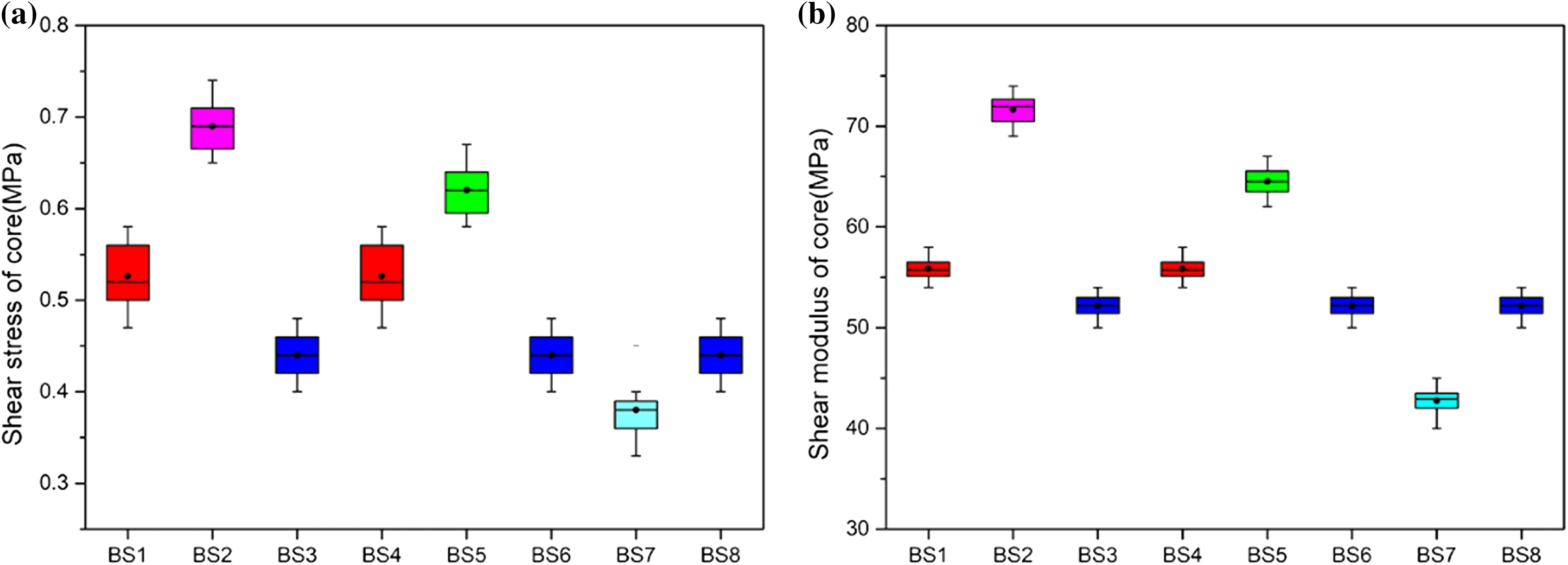
Fig.6 The shear stress ( a) and the shear modulus ( b) of core of the sandwich structure

Different unit specification

The load–displacement curves of type BS1 and BS2 specimens are shown in Fig.4 b. The curves can be divided into two stages: the linear elastic stage and the platform stage after the peak load. In the platform stage, the curve of type BS4 specimen is higher than that of type BS3 specimen. The shear stress and modulus of core of type BS4 specimen are 20.45 and 7.46% higher than those of type BS3 specimen, respectively (Fig.6). The shear stress of core of type BS3 and BS4 specimens are significantly different, but the shear modulus of core of two types of specimens are very close, which indicates that different unit specifications will have a certain impact on the shear stress of core, but a small impact on the shear modulus of core. The smaller the unit specification is, the higher the relative density of the core with the same size, the greater the shear stress, and the stronger the bearing capacity under the three-point bending load. Type BS4 specimen has better bearing capacity than Type BS3 specimen. The main failure mode of type BS3 specimen is that the upper panel crumples or collapses first (Fig.5 c), while the main failure mode of type BS4 specimen is that the upper panel crumples or collapses at the same time as the lower panel (Fig.5 d).

Different core size

The load–displacement curves of type BS5 and BS6 specimens are shown in Fig.4 c. The curve is divided into two stages: the linear elastic stage and the slow descending stage after the peak load. The failure process of the structure is as follows: under the action of load, the sandwich structure enters the linear elastic stage, and the deflection increases rapidly and uniformly with the load at this stage, but no obvious signs of failure are found in all parts of the structure. With the increase of the load, the sandwich structure enters the plastic failure stage. Compared with the load whose growth rate slowly declines, the growth rate of deflection is accelerated. The crack defect on the lower surface in the middle of the upper panel of the sandwich structure begins to expand rapidly and gradually. At this time, the load of sandwich structure reaches the maximum peak value, and the sandwich structure enters the failure stage. Then the crack expanded rapidly, the load decreased slowly, but the deflection increased rapidly, and the load–displacement curve appeared a long platform area. When the fiber of particleboard on the lower surface of the upper panel of sandwich structure is obviously separated from the original plane, the load begins to decline. All inclined bars are under compression load and transverse shear force, and the load decreases slowly until the upper and lower end of the panel shows obvious fracture, and the whole structure loses its bearing capacity. The shear stress and modulus of core of type BS5 specimen are 40.91% and 25.49% higher than those of type BS6 specimen, respectively (Fig.6). The core size (length) has an effect on the shear performance of the core of wood-based x-lattice sandwich structure. The shorter the core, the stronger the bearing capacity of the structure, the smaller the modulus of flexural section of the specimen, the greater the strength of static bending, and the better the shear performance. The failure modes of the type BS5 and BS6 specimens are mainly the failure of the crushing of the upper panels (Fig.5 e, f).

Different drilling depth

The load–displacement curves of type BS7 and BS8 specimens are shown in Fig.4 d. The two curves are different. The curve of type BS7 specimen can be divided into two stages: In the first stage, namely, the linear elastic stage, it rises in a straight line. After the linear elastic stage, the curve reaches the peak load, and cracks appear in the upper panel of the sandwich structure. After passing the load peak, it enters the second stage, that is, the slow decline stage after the load peak. The curve first drops rapidly, and then enters a long platform area, where the core shear modulus is 42.83 mpa. Finally, the upper panel of the specimen collapsed in the middle of the span. The curve of type BS8 specimen can be divided into three stages: The curve shows a good linearity in the first stage, that is, the linear elastic stage. With the increase of load, it enters the second stage, namely the plastic stage, and the rise rate of curve slows down. Then the load drops rapidly and enters the third stage, which is the platform stage after the peak load, accompanied by a long platform area. Finally, the upper panel of the specimen collapsed in the middle of the span. The shear stress and modulus of core of type BS8 specimen are 15.79% and 21.08% higher than those of type BS7 specimen, respectively (Fig.6). The drilling depth has an effect on the shear performance of cores of the wood-based X-type lattice sandwich structure. The greater the drilling depth is, the better the shear performance of core is. This is because the greater the drilling depth is, the greater the bonding area between the core and the panel is, and the greater the corresponding bonding force is. The failure modes of the type BS5 and BS6 specimens are mainly the failure of the crushing of the upper panels (Fig.5 g, h), which indicates that the adhesive relay of the core is suffi cient to withstand the transverse shear force, but the pressure bearing performance of the panel is not enough.

Comparison and analysis of experimental values and theoretical predicted values

The deflection response is the key point of shear performance of core analysis of the wood-based X-type lattice sandwich structure. In general, the deflection is the linear displacement of the centroid of the specimen’s cross section along the vertical direction of the axial direction under the bending load of three points. Manalo ( 2013) studied the behavior of structural fiber composite sandwich beams made up of glass fiber composite skins and phenolic core material under three-point short beam and asymmetrical beam shear tests. The deflection response of structural fiber composite sandwich beams is 1.72 mm, which is smaller than that of the 8 types of lattice structures in this paper under the same span thickness ratio. The performance of the sandwich structure can be comprehensively understood by comparing the theoretical and experimental values of deflection response under the action of three-point bending. The theoretical and experimental values of deflection response of the X-type lattice sandwich structure are shown in Table 3.

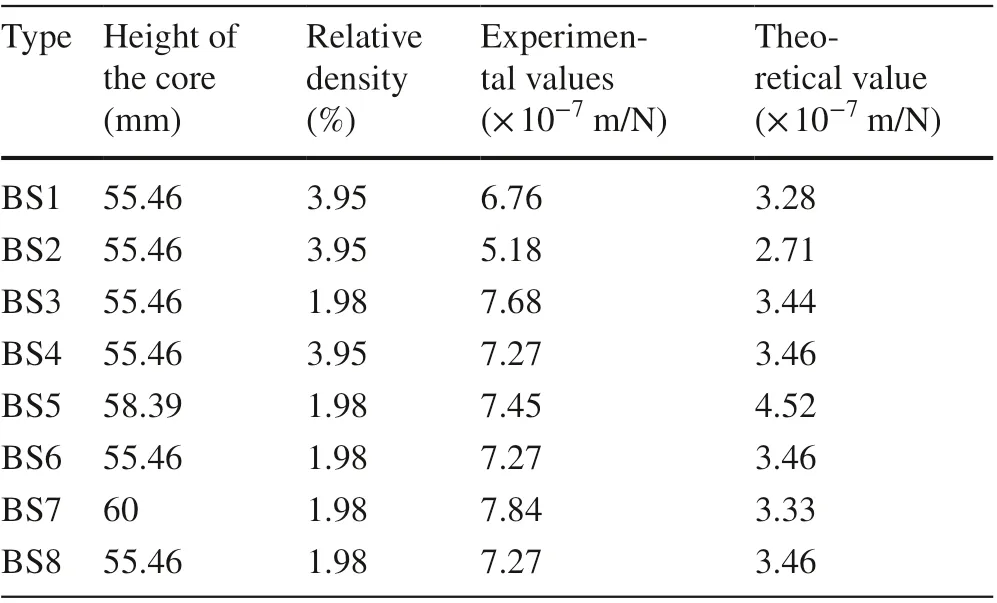
Table 3 The theoretical and experimental values of deflection response of the X-type lattice sandwich structure

As shown in Table 3, the experimental values of deflection response of various types’ specimens are higher than the theoretical values of them. The reason for the above situation may be that the contact of the testing machine and the specimen panel fully contact and produce friction, which is easy to cause the loss ofenergy. In addition, the applicable condition of this theory is that the thickness of the panel is smaller than that of the whole sandwich structure specimen, while the thickness of the wood-based X-type lattice sandwich structure designed in this study accounts for more than the thickness of the whole specimen. Finally, due to the limitation of manual process, the panel and round bar tenon are manually connected by adhesive, so it is impossible to ensure the complete parallelism of the upper and lower panels, so it is easy to make errors in theoretical calculation. The deflection caused by transverse shear force is much larger than that caused by bending action. When calculating the wood-based X-type lattice sandwich structure, the bending stiff ness of the core and the bending stiff ness caused by the displacement of the panel axis cannot be ignored compared with the general bending theory of the panel.

Conclusion

The wood-based X-type lattice sandwich structure was prepared by an insertion-glue method with Oriented Strand Boards serving as panel and birch serving as core. According to the short beam shear test and theoretical model, the following conclusions on the short beam shear properties and the failure modes of the wood-based X-type lattice sandwich structure were drawn:

1. The failure modes of the structure were mainly the wrinkling and crushing of the panel under three-point bending load, and the failure position occurred at the local loading position, which was also the place where the specimen suffered the greatest bending strength.

2. The shear performance of the core direction perpendicular to the directional particleboard shavings direction was better than that of the core direction parallel to the directional particleboard shavings direction. The smaller the unit size, the greater the relative density of the core, the greater the shear stress, and the stronger the bearing capacity. The shorter the core, the stronger the bearing capacity and the better the short beam shear properties of the sandwich structure. The greater the drilling depth, the greater the bonding area between the core and the panel, and the greater the corresponding bonding force, the better the short beam shear properties of the sandwich structure.

3. The experimental values of deflection response of the sandwich structure were higher than the theoretical value, which was due to manual manufacturing. The deflection caused by transverse shear forces is much greater than that caused by bending forces. In the calculation of wood-based X-type lattice sandwich structure, compared with the general bending theory of the panel, the bending stiff ness of the core material and the bending stiff ness caused by the displacement of the panel axis cannot be ignored.

Open AccessThis article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creat iveco mmons.org/licen ses/by/4.0/.

References

Deshpande VS, Fleck NA (2001) Collapse of truss core sandwich beams in 3-point bending. Int J Solids Struct 38:6275–6305

Elias H, Ragnar J, Jaana K, Janne J, Marko M, Pekka L, Lauri H (2018) Diversification of the forest industries: role of new wood-based products. Can J For Res 48(12):1417–1432

Evans AG, Hutchinson JW, Fleck NA, Ashby MF, Wadley HNG (2001) The topological design of multifunctional cellular metals. Prog Mater Sci 46:309–327

Fam A, Sharaf T (2010) Flexural performance of sandwich panels comprising polyurethane core and GFRP skins and ribs of various configurations. Compos Struct 92:2929–2935

Fan HL, Meng FH, Yang W (2006) Mechanical behaviors and bending effects of carbon fiber reinforced lattice materials. Arch Appl Mech 10–12:635–647

Fan HL, Meng FH, Yang W (2007) Sandwich panels with Kagome lattice cores reinforced by carbon fibers. Compos Struct 4:533–539

Hao MR, Hu YC, Wang B (2017) Mechanical behavior of natural fiberbased isogrid lattice cylinder. Compos Struct 176:117–123

He M, Hu W (2008) A study on composite honeycomb sandwich panel structure. Mater Des 29:709–713

Jin MM, Hu YC, Wang B (2015) Compressive and bending behaviours of wood-based two-dimensional lattice truss core sandwich structures. Compos Struct 124:337–344

Katsigris E, Bull GQ, White A, Barr C, Barney K, Bun Y, Kahrl F, King T, Lankin A, Lebedev A, Shearman P, Sheingauz A, Yufang SU, Weyerhaeuser H (2004) The China forest products trade: overview of Asia-Pacific supplying countries, impacts and implications. Int For Rev 3–4:237–253

Lee MG, Yoon JW, Han SM, Suh YS, Kang KJ (2014) Bending response of sandwich panels with discontinuous wire-woven metal cores. Mater Des 55:707–717

Li XW, Hu YC (2019) Luminescent films functionalized with cellulose nanofibrils/Cd Te quantum dots for anti-counterfeiting applications. Carbohydr Polym 203:167–175

Li S, Qin JK, Li CC, Feng YX, Zhao X, Hu YC (2018) Optimization and compressive behavior of composite 2-D lattice structure. Mech Adv Mater Struct 1:1–10

Li S, Qin JK, Wang B, Zheng TT, Hu YC (2019a) Design and compressive behavior of a photosensitive resin-based 2-D lattice structure with variable cross-section core. Polymers 11:186

Li XH, Liu YZ, Yu YY, Chen WS, Liu YX, Yu HP (2019b) Nanoformulations of quercetin and cellulose nanofibers as healthcare supplements with sustained antioxidant activity. Carbohydr Polym 207:160–168

Lim CH, Jeon I, Kang KJ (2009) A new type of sandwich panel with periodic cellular metal cores and its mechanical performances. Mater Des 30:3082–3093

Lou J, Ma L, Wu LZ (2011) Free vibration analysis of composite tetrahedral lattice sandwich beam. J Solid Mech 4(2):339–345 (in China)

Lu TJ, Liu SM, Jing M, Xu XL, Wang Y, Wang ZY, Gou J, Hui D, Zhou ZW (2014) Effects of modifications of bamboo cellulose fibers on the improved mechanical properties of cellulose reinforced poly (lactic acid) composites. Compos Pt B Eng 62:191–197

Manalo AC (2013) Behaviour of fibre composite sandwich structures under short and asymmetrical beam shear tests. Compos Struct 99:339–349

Manalo AC, Aravinthan T, Karunasena W (2010) In-plane shear behaviour of fibre composite sandwich beams using asymmetrical beam shear test. Constr Build Mater 24(10):1952–1960

Marius C, Semiyou P, Edmond CA, Emmanuel O, Valerie D (2018) Study of the implementation of waste wood, plastics and polystyrenes for various applications in the building industry. Constr Build Mater 167:936–941

Min X, Zhi C (2004) Effects of different modifiers on the properties of wood-polymer composites. J For Res 15(1):77–79

Nguyen VD, Nguyen TT, Zhang AH, Hao JX, Wang WH (2019) Effect of three tree species on UV weathering of wood flour-HDPE composites. J For Res 53:1–9

Qin JK, Li XW, Shao YL, Shi KX, Zhao X, Feng TS, Hu YC (2018) Optimization of delignification process for effi cient preparation of transparent wood with high strength and high transmittance. Vacuum 158:158–165

Qin JK, Zheng TT, Li S, Cheng YP, Xu QY, Ye GY, Hu YC (2019) Core configuration and panel reinforcement affect compression properties of wood-based 2-D straight column lattice truss sandwich structure. Eur J Wood Wood Prod 4:1–8

Sideridis E, Papadopoulus GA (2004) Short-beam and three-pointbending tests for the study of shear and flexural properties in unidirectional-fibre-reinforced epoxy composites. J Appl Polym Sci 93:63–74

Wang B, Zhang GQ, He QL, Ma L, Wu LZ, Feng JC (2014) Mechanical behavior of carbon fiber reinforced polymer composite sandwich panels with 2-D lattice truss cores. Mater Des 55:591–596

Wang B, Hu J, Li Y, Yao Y, Wang SX, Ma L (2018) Mechanical properties and failure behavior of the sandwich structures with carbon fiber-reinforced X-type lattice truss core. Compos Struct 185:619–633

Wang HG, Zhang JF, Fu HT, Wang WH, Wang QW (2019) Effect of an antioxidant on the life cycle of wood flour/polypropylene composites. J For Res 55:1–9

Yuan FP, Ou RX, Xie YJ, Wang QW (2013) Reinforcing effects of modified Kevlar ? fiber on the mechanical properties of woodflour/polypropylene composites. J For Res 24(1):149–153

Zhang JF, Wang HG, Ou RX, Wang QW (2018) The properties of flax fiber reinforced wood flour/high density polyethylene composites. J For Res 29(2):533–540

Zheng TT, Cheng YP, Li S, Zhang Y, Hu YC (2020a) Mechanical properties of the wood-based X-type lattice sandwich structure. BioResources 15:1927–1944

Zheng TT, Yan HZ, Li S, Cheng YP, Zou LX, Hu YC (2020b) Compressive behavior and failure modes of the wood-based double X-type lattice sandwich structure. J Buil Eng 30:101176