Abstract: The formula for the analytical calculations of the shaped charge jetting parameters exhibit an explicit dependence upon the Gurney velocity of an explosive material. Many attempts have been done to approximate the characteristic constant and to make its use more efficient. It is shown herein that the characteristic Gurney velocity parameter depends on the ratio of the Chapman Jouguet-pressure to explosive impulse ratio by the where PCJ is the Chapman Jouguet pressure (Pa), ISP is the specific impulse of the explosive used as a monopropellant (N·s/kg) and ρo is the explosive density(kg/m3). The proposed empirical formula was found to be reasonable and quite accurate approximation that can be implemented over a wide range of explosives, where the maximum deviation between the measured and the calculated Gurney velocities was nearly 6%. Besides, the proposed approach has been used analytically to calculate the jet tip velocity of a small caliber shaped charge, which is measured experimentally. The difference between the measured and the calculated jet tip velocity was only 1.6%.

Keywords: Gurney velocity; metal acceleration; explosives; jet tip velocity

Introduction

The model of an explosively-driven metal is used to predict the fragmentation velocities, the flyer plate motions and the collapse velocities of the shaped charge liners. Many authors have attempted to deduce simple relations governing the driven metal velocity under the effect of detonation gaseous products. Gurney[1]tried to identify the chemical energy liberated from the detonation of a high explosive that could be imparted to the metal in contact with the explosive, causing its acceleration and attaining terminal velocity[2]. This energy is called Gurney energy (E) and is considered as an intrinsic property for each explosive. It was defined as the part of the total chemical energy of explosive that is released during detonation and converted to the kinetic energy of the metal. The final kinetic energy from the detonation of explosive was partitioned between the kinetic energy of the driven metal and the gaseous product by an estimated linear velocity profile. To simplify the calculations of the terminal velocity of the metal, following assumptions were normally taken:

The detonation products are assumed to expand uniformly with constant density;Rarefaction and shock wave effect within the solid metals due to shock waves are neglected;The total Gurney energy is divided into the kinetic energy of the gas expansion and the kinetic energy of the driven elements in contact with the explosive.

The Gurney model was essentially based on the principles of momentum and energy conservations. It could be applied to any one-dimensional explosive-metal interaction system. The Gurney approximation exhibits high accuracy for the prediction of the final metal velocity over the range of mass ratio between metal (M) and explosive (C) from 0.1 to 10[2]. Many investigators introduced their analytical formulae used to determine the Gurney velocity and discuss the effect of metals and explosives formulations[3-4]. But for the common shaped charges, the liner collapse velocity was determined using the formulae derived by different researchers[5]. Gui-Xi[6]extended the Gurney model to small shaped charges, whereas he also deduced another formula considering the effect of the confinement of the charge on the collapse velocity. The detonation characteristics of several highly pressed plastic bonded explosives (PBXs) and different plastic explosives have been studied[7-10]where these parameters could be used to determine the explosive Gurney energy. Also the effect of the geometry of different configurations was also studied by several researchers[11-13].

These studies show that the terminal velocity of the metal depends on the configurations of the metal-explosive interactions, the explosive Gurney energy and the mass ratioM/C.

1 Theories and fundamentals

Kennedy[14]provided an easy approximation to determine the Gurney energy for some explosives. This approximation is:

E=0.7Qv

(1)

WhereQvis the heat of explosion of the explosive. However, for most commonly used explosives, the Gurney energy;Eis the energy from the detonation that is responsible for metal acceleration.Evaries between 0.61Qvand 0.7Qv.

In 2002, Koch et al.[15]used the low of energy conservation to get a relation between the explosive detonation velocity and its Gurney velocity. They applied the energy conservation law to different explosive metal configuration, symmetrical plates, cylinder filled with explosive core and hollow metallic sphere filled with a solid explosive. For common explosives, it was found that the Gurney velocity =UD/3.08, whereUDis the detonation velocity of the explosive, which generally agrees with existing estimations[15]. Furthermore, Gurney velocity has been experimentally determined for certain explosives, which are listed in Table 1. The average value calculated for these explosives ofUD/(2E)1/2is 3.19 with a standard deviation of 0.2, which means that this formula presents a reliable tool to estimate the Gurney velocity for certain explosives based on their detonation velocity. In 2006, Keshavarz and Abolfazl[16]extended this definition to include the detonation gaseous and solid products and their heat of formation. The proposed new relationship betweenEandQvis similar to those calculated by the existing approximations for a range of different explosives.

Hurley[18]proposed a new function to predict the Gurney Velocity of ammonium nitrate based explosives based on their detonation velocity. This proposed relationship is applied to a weld-ability window. In 2012, Ludwig and Wibke discussed various expressions for the analytical and empirical Gurney formula[19]. In 2015, Frem suggested an approximation to the Gurney constant depending on the empirical thermochemical BKW equation of state of various high explosives[20].

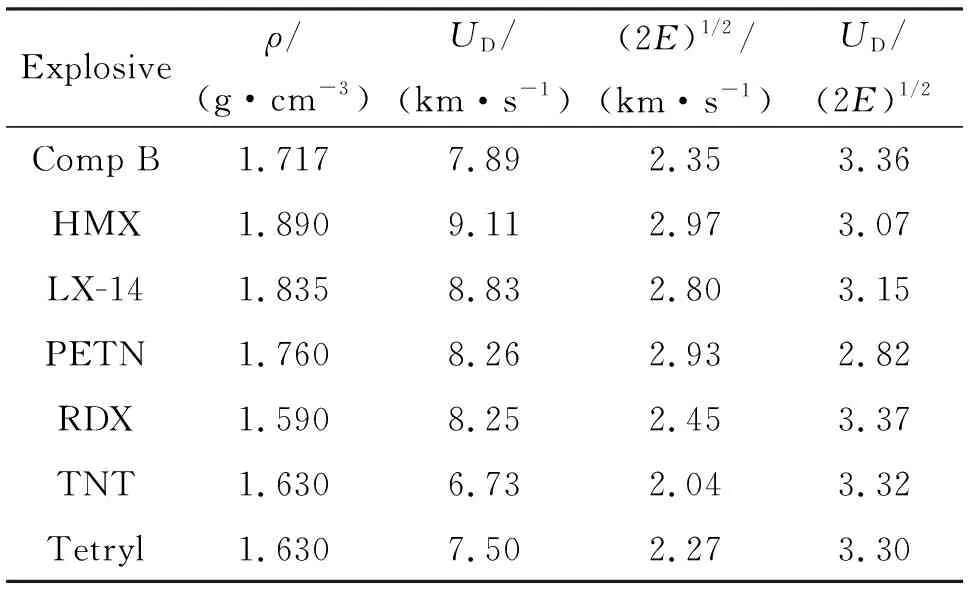
Table 1 Explosive characteristics and Gurney velocity for some common explosives [17]

The introduced approximations have quite accurate estimation of the characteristic of Gurney velocity, but it does not include the specific impulse of an energetic material that represents the change in momentum of this energetic material, it has unit of velocity. The next section thus discusses the relation between the characteristic Gurney velocity and its dependence on the specific impulse of an explosive regardless the value of detonation velocity[15, 18]or the heat of formation of this energetic material[16].

2 Results and discussion

The Gurney velocities of various explosive types are depicted in Figure 1 against the characteristic explosive Chapman Jouget pressure to its total impulse ratio. The following relation was obtained from the fitting of the previously calculated Gurney velocity against thePCJ/ISP.ρ0ratio.

(2)

wherePCJis the Chapman Jouguet pressure (Pa),ISPis the specific impulse of the explosive used as a monopropellant (N·s/kg) and ρ0is the explosive density (kg/m3). If the impulse of the explosive is not known, it can be calculated using the empirical-impulse relation for CaHbNcOdexplosives[17]:

ISP=2.4205-0.074a-0.0036b+0.00237c+
0.04a-0.1001nNHx+0.1466(nAr-1)

(3)

whereISPin N·s/kg, is the number of NH2and NH groups and is the number of aromatic rings in aromatic explosives. The presented equation exhibited quite accurate approximation for various pure and composite explosives as proved in Ref.[17]. Various explosives (21 types) were studied and their pressure, impulse and densities are listed in Table 2.PCJvalues have been determined using Explo 5 thermodynamic code, where BKWN equation of state was applied[7,10]. The calculated and the measured Gurney velocities as well as the deviation between them are also presented in Table 2. The greatest deviation between the measured and the calculated Gurney velocities based on Eq. (2) is 4.8%, which means that this approximation can be used accurately over a wide range of explosives.

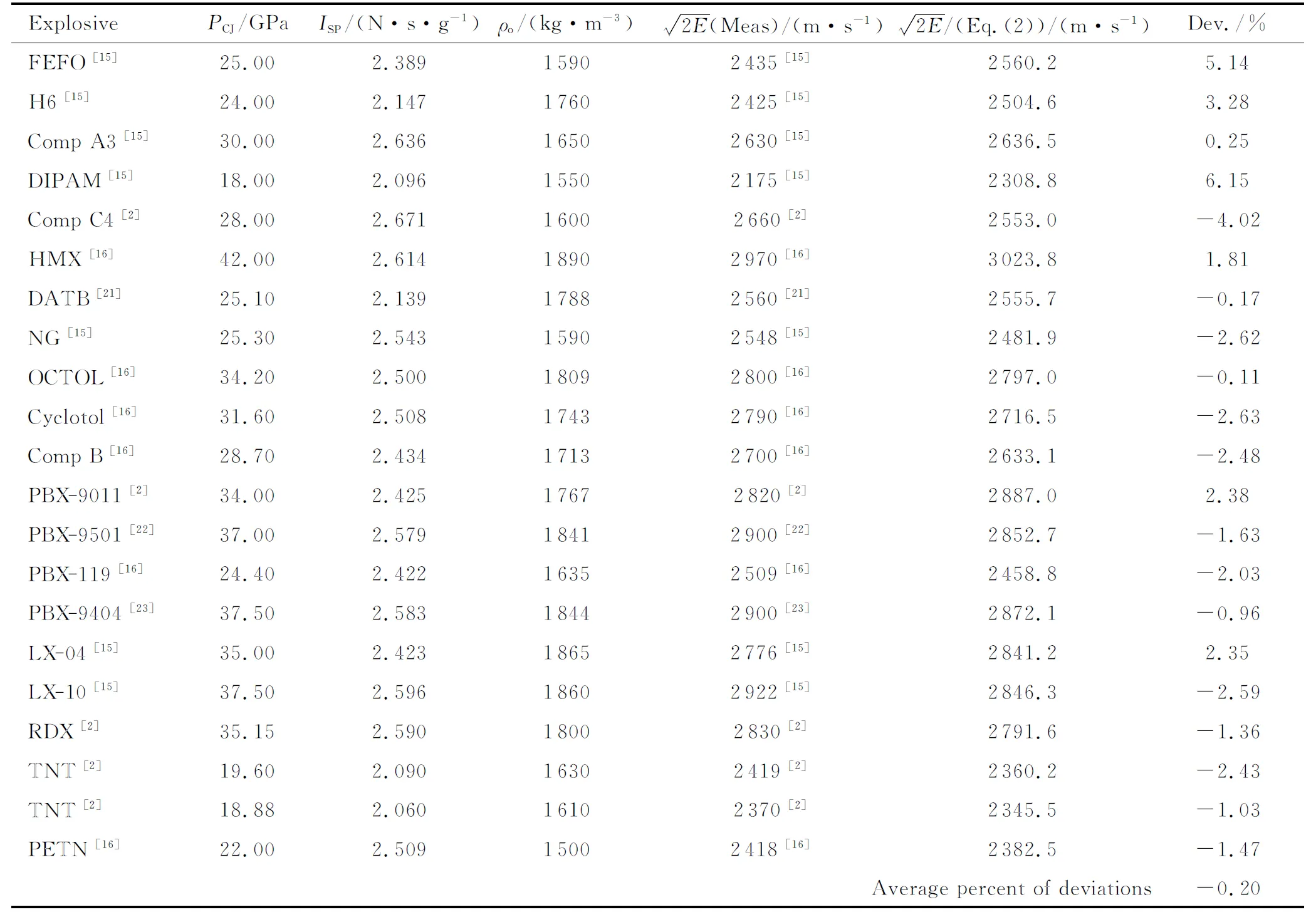
ExplosivePCJ/GPaISP/(N·s·g-1)ρo/(kg·m-3)2E(Meas)/(m·s-1)2E/(Eq.(2))/(m·s-1)Dev./%FEFO [15]25.002.38915902435 [15]2560.25.14H6 [15]24.002.14717602425 [15]2504.63.28Comp A3 [15]30.002.63616502630 [15]2636.50.25DIPAM [15]18.002.09615502175 [15]2308.86.15Comp C4 [2]28.002.67116002660 [2]2553.0-4.02HMX [16]42.002.61418902970 [16]3023.81.81DATB [21]25.102.13917882560 [21]2555.7-0.17NG [15]25.302.54315902548 [15]2481.9-2.62OCTOL [16]34.202.50018092800 [16]2797.0-0.11Cyclotol [16]31.602.50817432790 [16]2716.5-2.63Comp B [16]28.702.43417132700 [16]2633.1-2.48PBX-9011 [2]34.002.42517672820 [2]2887.02.38PBX-9501 [22]37.002.57918412900 [22]2852.7-1.63PBX-119 [16]24.402.42216352509 [16] 2458.8-2.03PBX-9404 [23]37.502.58318442900 [23]2872.1-0.96LX-04 [15]35.002.42318652776 [15]2841.22.35LX-10 [15]37.502.59618602922 [15]2846.3-2.59RDX [2]35.152.59018002830 [2]2791.6-1.36TNT [2]19.602.09016302419 [2]2360.2-2.43TNT [2]18.882.06016102370 [2]2345.5-1.03PETN [16]22.002.50915002418 [16]2382.5-1.47Average percent of deviations-0.20

Note:Dev.= (Calculated-Measured)×100/Measured

3 Verification of the presented formulae

In the present research, the verification of the suggested Gurney formulae has been performed using flash X-ray radiograph and the multi-layer electric aluminium panels that have been used to measure the jet tip velocity and to depict the jet profile at different times from the point of initiation. The used shaped charge has a conical copper liner of angle 45 degree, a wall thickness of 1.3mm and a charge calibre of 36.6mm. The used explosive charge is composition C4[24]of loading density 1.61g/cm3and mass of 30g, while the charge casing is made up of mild steel as shown in Figure 2.

The shaped charge is attached to the top of the laminated electric foil layers separated by 25cm provided with electric circuits that are used to determine the jet tip velocity as it passes through them as shown in Figure 3.

The flash X-ray trial was performed in COTEC (Cranfield Ordnance Test and Evaluation Centre) field. Various heads were used to capture photos of the jet profile at different times. Figure 4 shows the jet shapes obtained from X-ray radiograph and the numerical jet formations using Autodyn hydrocode at 34μs and 122μs, respectively. A curved shape is observed for the real X-ray jet, which may be caused either by some asymmetries in the liner positioning during the manual filling of the charge or due to the non-uniform explosive mass distribution inside the charge cavity. Nevertheless, the general aspects of the jet shapes are similar.

The proposed approach has been used within the unsteady state PER theory[2]for the complete jetting calculations using the value of the Gurney velocity obtained from Eq. (2) for the Comp. C4 explosive that was found to be 2553m/s. Thus, the jet tip velocities were found to be 6100m/s and 6000 m/s from the real measurements and the analytical model based on unsteady state PER theory, respectively, as shown in Fig.5. The jet velocity was calculated via the direct explicit relation among the collapse velocityV0, the stagnation velocityV1, the flow velocityV2and the collapse and deflection angles as well. For shaped charges, an often-used formula is that of a single flat plate backed by a slab of explosive, the collapse velocityV0is calculated by references[25]:

(4)

whereμis the metal/explosive mass ratio andV0is the collapse velocity of the liner element.

The collapse velocity is then used to estimate both the flow velocity and the stagnation velocity as follow:

(5)

(6)

whereV1andV2are stagnation and flow velocities during jet formation respectively whereasαis the half cone apex angle andβis the collapse angle.

Thus the jet velocity is calculated from:

Vj=V1+V2

(7)

while the jet massmjis calculated fromEq.

(8)

wheremis the total liner mass.

Further details of the analytical shaped charge modelling can be obtained from Ref.[2, 6, 22]. Besides, the obtained jet velocities of few elements on its axis show the relevant accuracy of the analytical model depending on the approached Gurney velocity of the chosen C4 explosive. This result means that the error percent in the jet tip velocity is only 1.6%, which implies that the suggested Gurney formulae can be used effectively to calculate the shaped charge jet characteristics with reasonable accuracy.

4 Conclusion

The relation between the characteristics Gurney velocity of a range of explosive materials and the ratio between the Chapman Jouget pressures to the total impulse is studied. It was found that the presented empirical relation exhibits a relative accurate tool that can used to estimate the characteristic Gurney velocity, where the maximum deviation between the measured and the calculated Gurney velocities was nearly 6%. Thus, the calculated Gurney constant was implemented in the analytical method to calculate the jet tip velocity using small caliber shaped charge, where it showed quite accurate model based on the small difference between the measured and the calculated tip velocity using the suggested Gurney approach.