STRONG INSTABILITY OF STANDING WAVES FOR A SYSTEM NLS WITH QUADRATIC INTERACTION?
Laboratoire Paul Painlevé UMR 8524,Université deLille CNRS,59655 Villeneuve d’Ascq,Cedex,France
Department of Mathematics,HCMC University of Pedagogy,280 An Duong Vuong,Ho Chi Minh,Vietnam
E-mail:contact@duongdinh.com
Abstract We study the strong instability of standing waves for a system of nonlinear Schr?dinger equations with quadratic interaction under the mass resonance condition in dimension d=5.
Key words System NLS quadratic interaction;ground states;instability;blow-up
1 Introduction
We consider the system NLS equations

The system(1.1)is regarded as a non-relativistic limit of the system of nonlinear Klein-Gordon equations
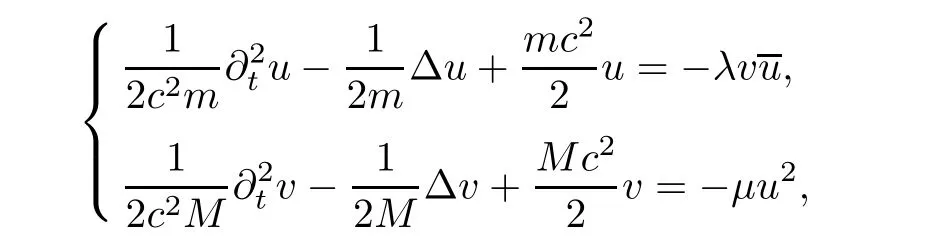
under the mass resonance condition

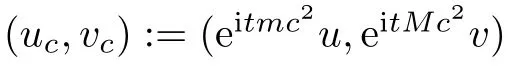
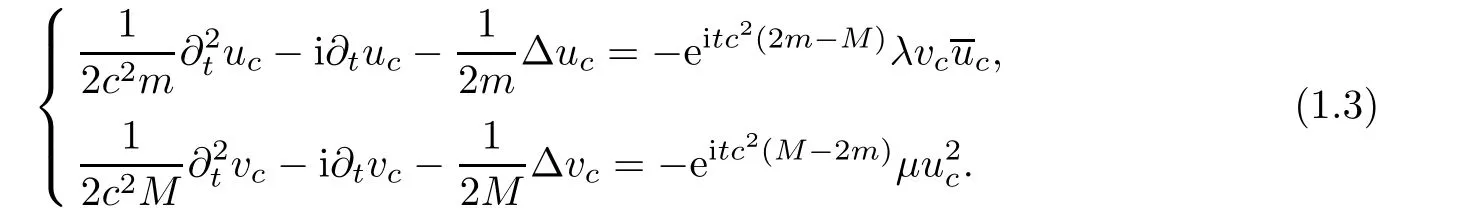
We see that the phase oscillations on the right hand sides vanish if and only if(1.2)holds,and system(1.3)formally yields(1.1)as the speed of light c tends to infinity.System(1.1)also appears in the interaction process for waves propagation in quadratic media(see e.g.[3]).
System(1.1)has attracted a lot of interest in past several years.The scattering theory and the asymptotic behavior of solutions were studied in[10–12,14].The Cauchy problem for(1.1)in L2,H1and in the weighted L2space hxi?1L2=F(H1)under mass resonance condition were studied in[13].The space-time analytic smoothing effect was studied in[7–9].The sharp threshold for scattering and blow-up for(1.1)under the mass resonance condition in dimension d=5 was studied in[6].The existence,stability of standing waves and the characterization of finite time blow-up solutions with minimal mass were studied recently in[4].
Let us recall the local well-posedness in H1for(1.1)due to[13].To ensure the conservation law of total charge,it is natural to consider the following condition:

Proposition 1.1(LWP in H1[13]) Let d≤ 6 and let λ and μ satisfy(1.4).Then,for any(u0,v0)∈H1×H1,there exists a unique paire of local solutions(u,v)∈Y(I)×Y(I)of(1.1)with initial data(u(0),v(0))=(u0,v0),where
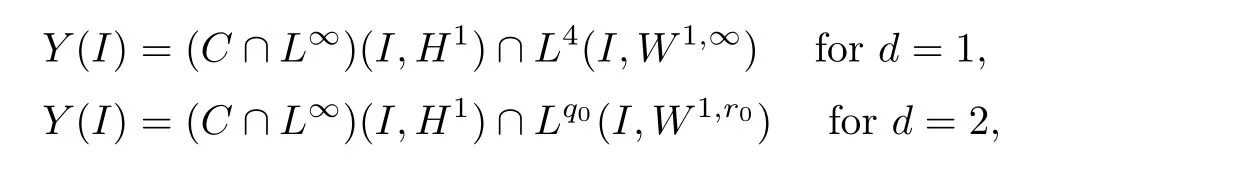
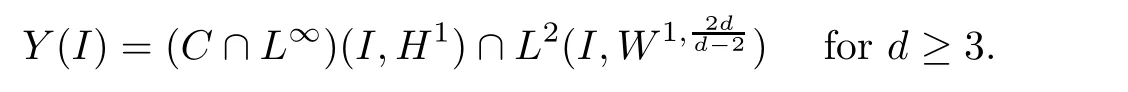
Moreover,the solution satisfies the conservation of mass and energy:for all t∈I,
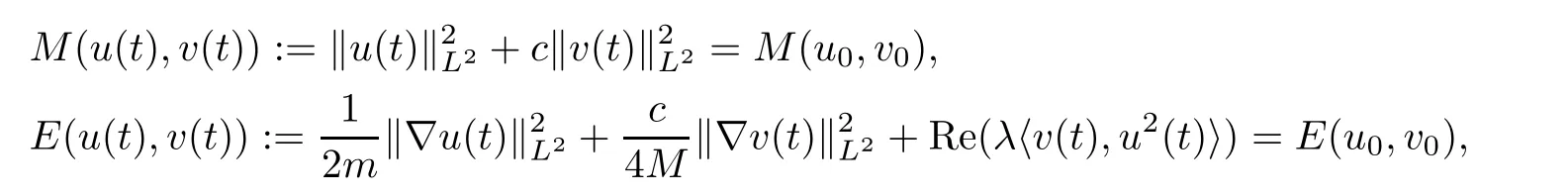
We now assume that λ and μ satisfy(1.4)with c>0 andBy change of variables
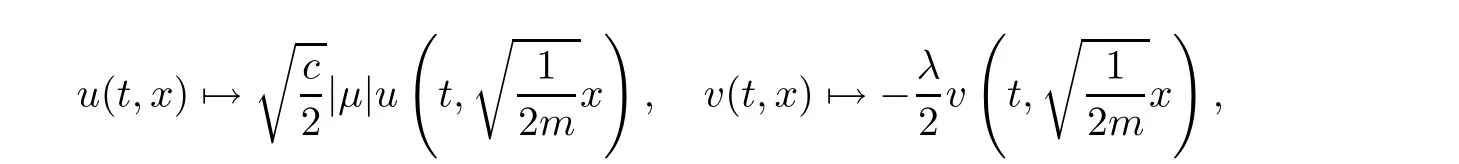
system(1.1)becomes

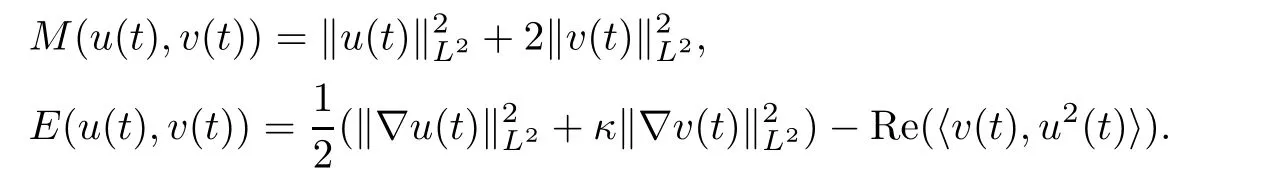
The local well-posedness in H1for(1.5)reads as follows.
Proposition 1.2(LWP in H1) Let d≤6.Then for any(u0,v0)∈H1×H1,there exists a unique pair of local solutions(u,v)∈Y(I)×Y(I)of(1.5)with initial data(u(0),v(0))=(u0,v0).Moreover,the solution satisfies the conservation of mass and energy:for all t∈I,
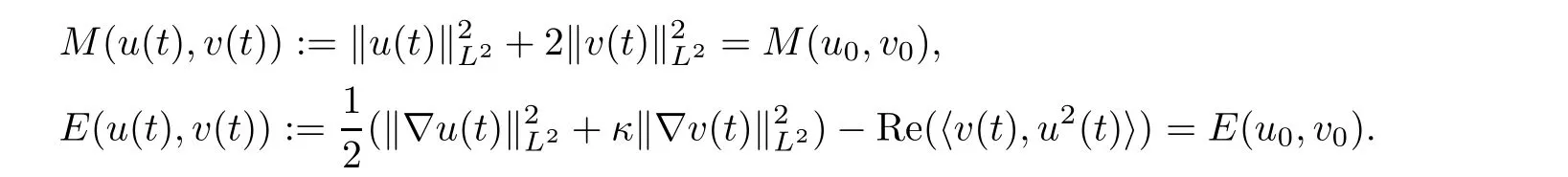
The main purpose of this article is to study the strong instability of standing waves for system(1.5)under the mass resonance conditionin dimension d=5.Let d=5 and consider
Throughout the sequel,a standing wave is a solution to(1.6)of the formwhere ω ∈ R is a frequency and(φω,ψω) ∈ H1× H1is a non-trivial solution to the elliptic system

We are interested in showing the strong instability of ground state standing waves for(1.6).Let us first introduce the notion of ground states related to(1.6).Denote
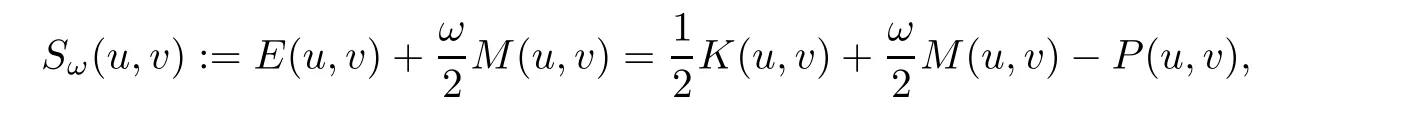
where
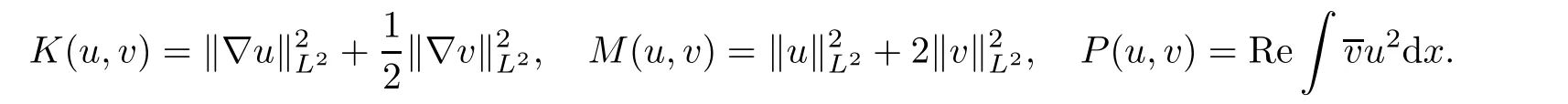
We also denote the set of non-trivial solutions of(1.7)by
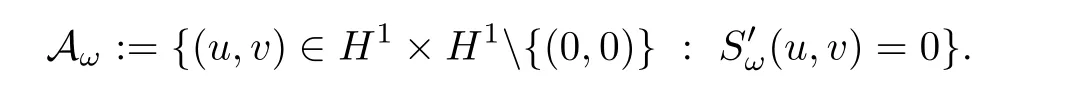
Definition 1.3A pair of functions(φ,ψ)∈ H1×H1is called ground state for(1.7)if it is a minimizer of Sωover the set Aω.The set of ground states is denoted by Gω.In particular,
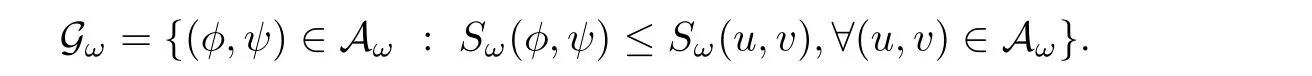
We have the following result on the existence of ground states for(1.7).
Proposition 1.4Let d=5,and ω >0.Then,the set Gωis not empty,and it is characterized by
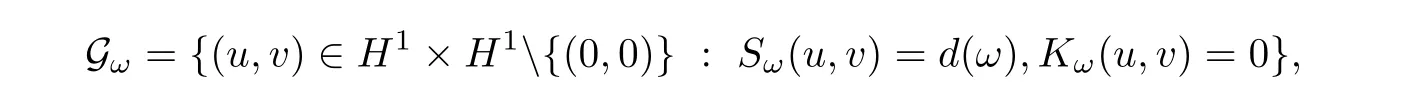
where
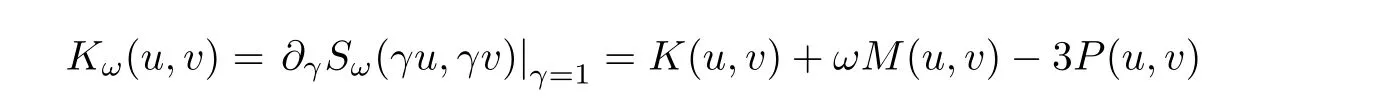
is the Nehari functional and
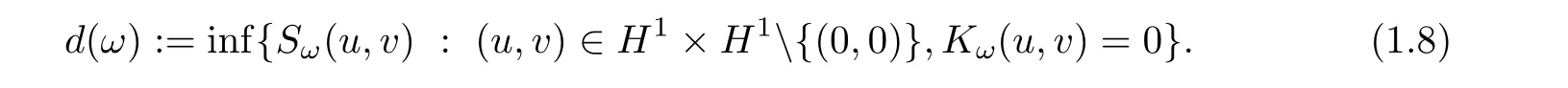
The existence of real-valued ground states for(1.7)was proved in[13](actually for d≤5 and κ>0).Here,we proved the existence of ground states(not necessary real-valued)and proved its characterization.This characterization plays an important role in the study of strong instability of ground states standing waves for(1.6).We only state and prove Proposition 1.4 for d=5 andHowever,it is still available for d ≤ 5 and κ >0.
We also recall the definition of the strong instability of standing waves.
Definition 1.5We say that the standing waveis strongly unstable if for any ?>0,there exists(u0,v0)∈ H1× H1such thatand the corresponding solution(u(t),v(t))to(1.6)with initial data(u(0),v(0))=(u0,v0)blows up infinite time.
Our main result of this article is the following.
Theorem 1.6Let d=5,ω >0,and(φω,ψω) ∈ Gω.Then,the ground state standing wavesfor(1.6)is strongly unstable.
To our knowledge,this article is the first one that addresses the strong instability of standing waves for a system of nonlinear Schr?dinger equations with quadratic interaction.In[2],Colin-Colin-Ohta proved the instability of standing waves for a system of nonlinear Schr?dinger equations with three waves interaction.However,they only studied the orbital instability not strong instability by blow-up,and they only consider a special standing wave solution(0,0,e2iωt?),where ? is the unique positive radial solution to the elliptic equation

This article is organized as follows.In Section 2,we show the existence of ground states and its characterization given in Proposition 1.4.In Section 3,we give the proof of the strong instability of standing waves given in Theorem 1.6.
2 Exitence of Ground States
We first show the existence of ground states given in Proposition 1.4.To do so,we need the following pro file decomposition.
Proposition 2.1(Pro file decomposition) Let d=5 andLetbe a bounded sequence in H1×H1.Then,there exist a subsequence,still denoted bya familyof sequences in R5and a sequenceof H1×H1-functions such that
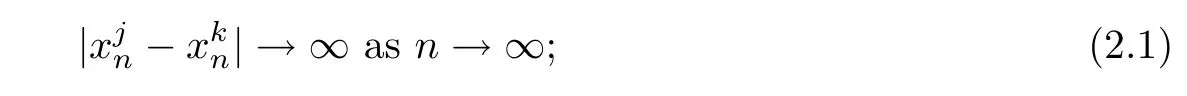
(2)for every l≥1 and every x∈R5,
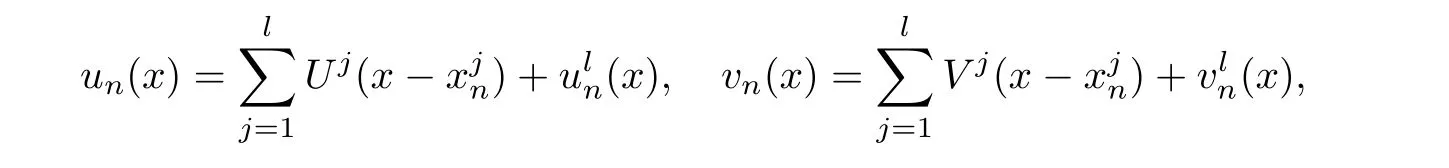
with
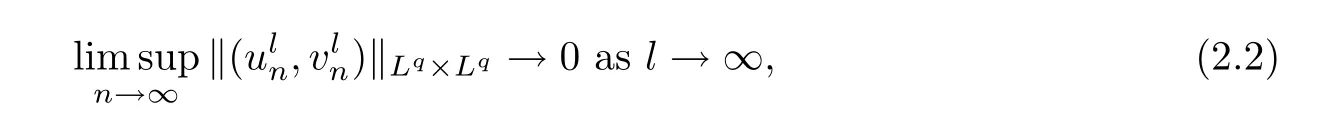
for every q∈(2,10/3).
Moreover,for every l≥1,
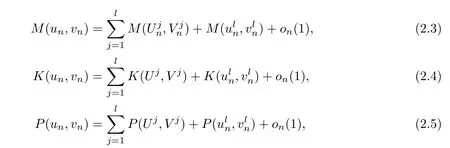
where on(1)→0 as n→∞.
We refer the reader to[4,Proposition 3.5]for the proof of this pro file decomposition.
The proof of Proposition 1.4 is done by several lemmas.To simplify the notation,we denote,for ω>0,
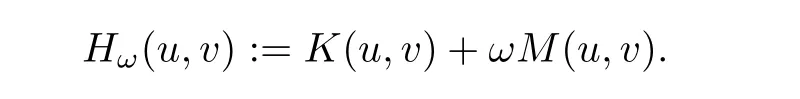
It is easy to see that for ω>0 fixed,we have

Note also that
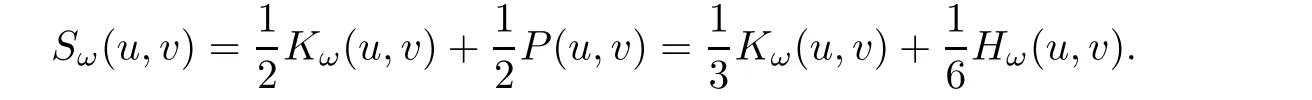
Lemma 2.2d(ω)>0.
ProofLet(u,v)∈ H1×H1{(0,0)}be such that Kω(u,v)=0 or H(u,v)=3P(u,v).From Sobolev embedding,we have
This implies that there exists C>0 such that
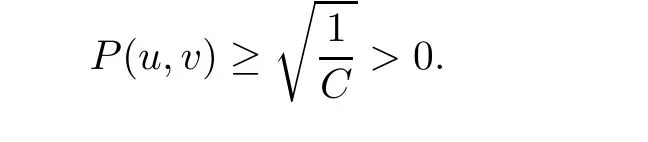
Thus,
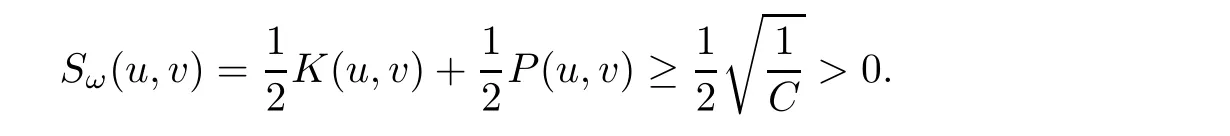
Taking the infimum over all(u,v)∈H1×H1{(0,0)}satisfying Kω(u,v)=0,we get the result.
We now denote the set of all minimizers for d(ω)by
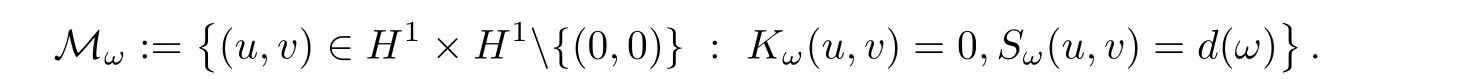
Lemma 2.3The set Mωis not empty.
ProofLet(un,vn)n≥1be a minimizing sequence for d(ω),that is,(un,vn) ∈ H1×H1{(0,0)},Kω(un,vn)=0 for any n≥1 andAs Kω(un,vn)=0,we have Hω(un,vn)=3P(un,vn)for any n≥1.We also have
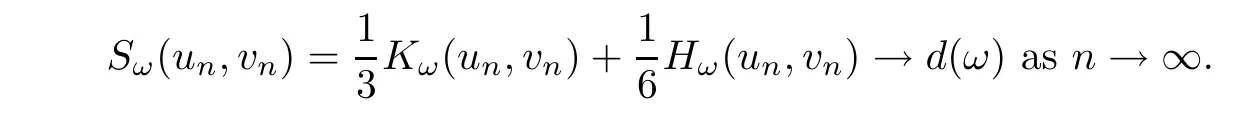
This yields that there exists C>0 such that
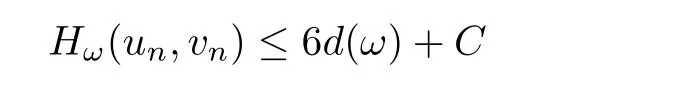
for all n ≥ 1.By(2.6),(un,vn)n≥1is a bounded sequence in H1×H1.We apply the pro file decomposition given in Proposition 2.1 to get(up to a subsequence)
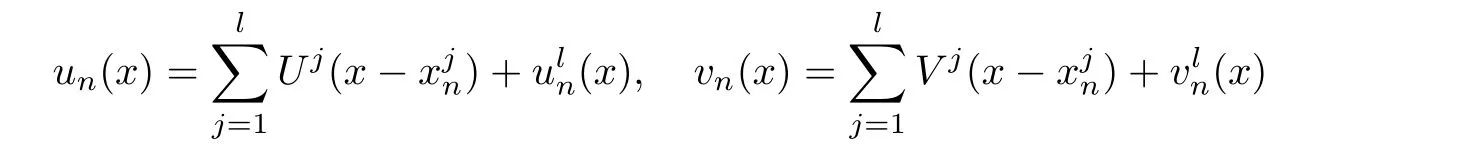
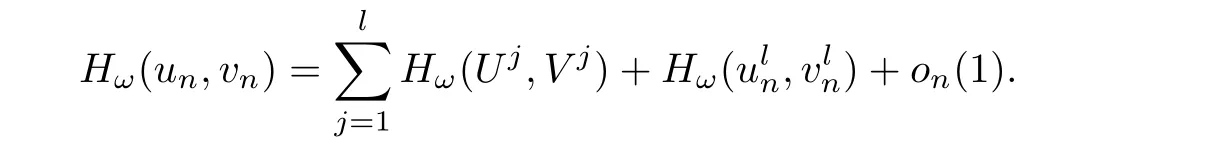
This implies that
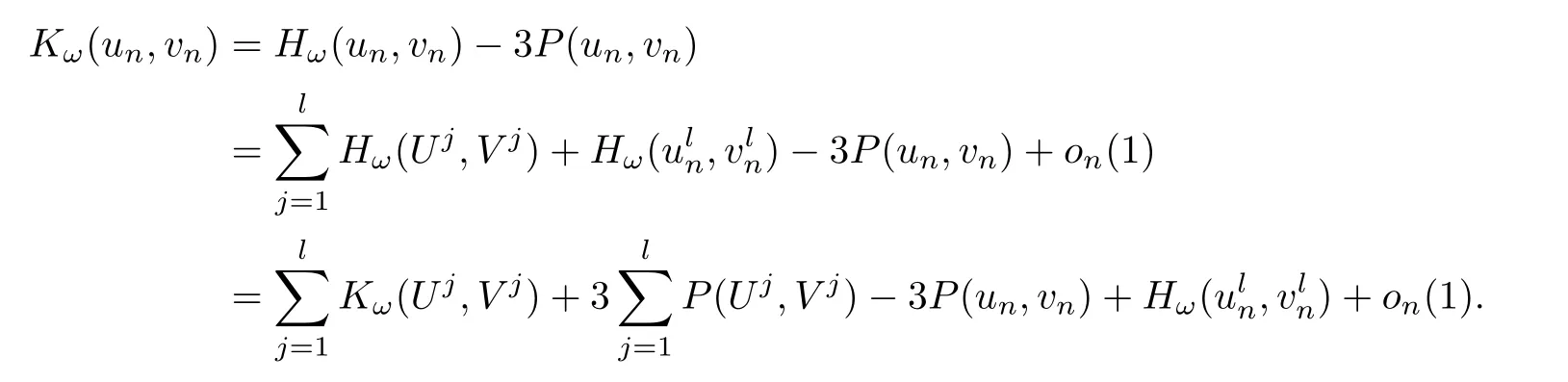
Because Kω(un,vn)=0 for any n≥ 1,P(un,vn)→2d(ω)as n→∞,andfor any n≥1,we infer that
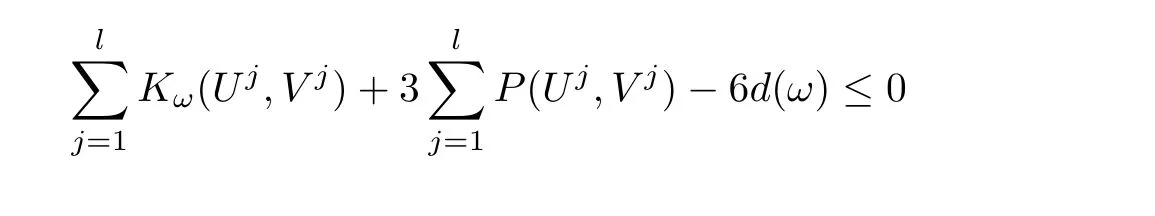
or
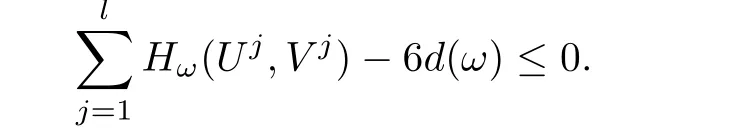
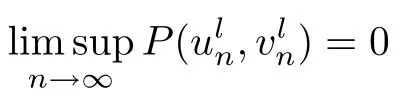
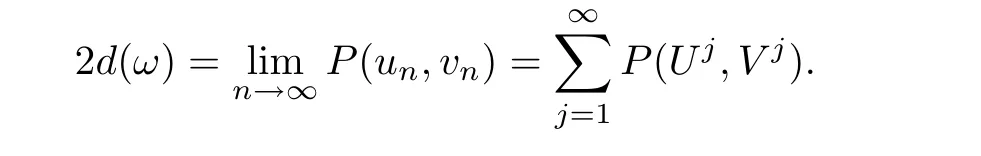
We thus obtain
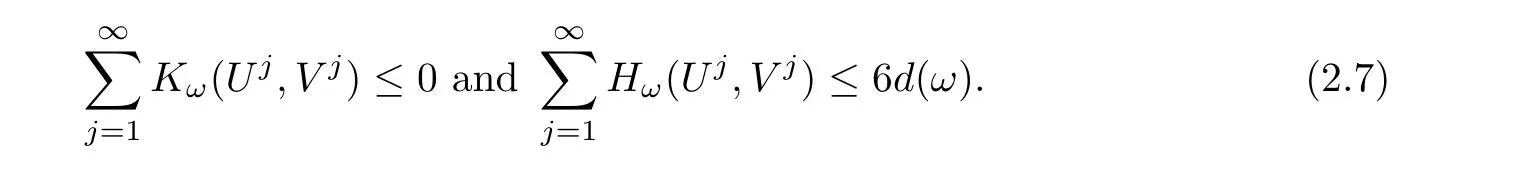
We now claim that Kω(Uj,Vj)=0 for all j≥ 1.Indeed,suppose that if there exists j0≥ 1 such thatthen we see that the equationadmits a unique non-zero solution
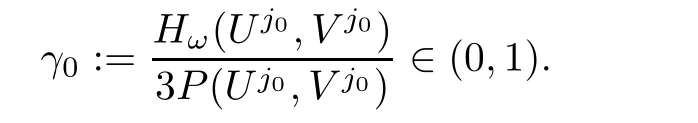
By the definition of d(ω),we have
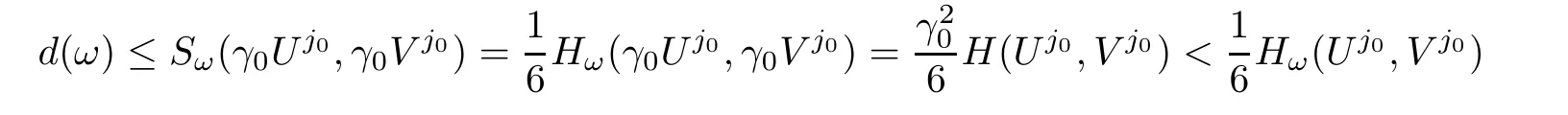
which contradicts to the second inequality in(2.7).We next claim that there exists only one j such that(Uj,Vj)is non-zero.Indeed,if there areandnon-zero,then by(2.7),bothare strictly smaller than 6d(ω).Moreover,as
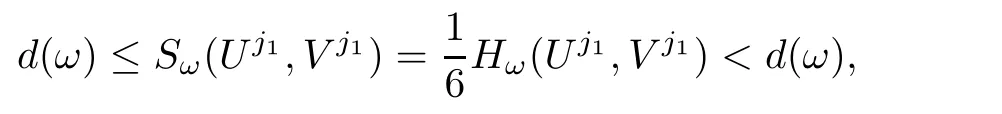
which is absurd.Therefore,without loss of generality,we may assume that the only one non-zero pro file is(U1,V1).We will show that(U1,V1)∈ Mω.Indeed,we have P(U1,V1)=2d(ω)>0,which impliesWe also have
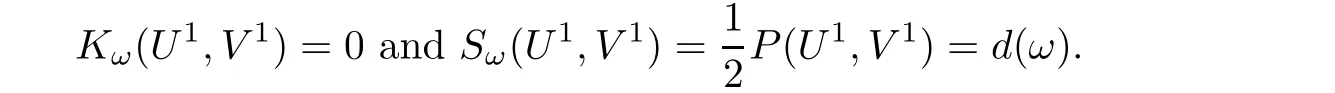
This shows that(U1,V1)is a minimizer for d(ω).The proof is complete.
Lemma 2.4Mω? Gω.
ProofLet(φ,ψ)∈ Mω.As Kω(φ,ψ)=0,we have Hω(φ,ψ)=3P(φ,ψ).On the other hand,as(φ,ψ)is a minimizer for d(ω),there exists a Lagrange multiplier γ ∈ R such that
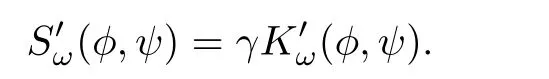
This implies that
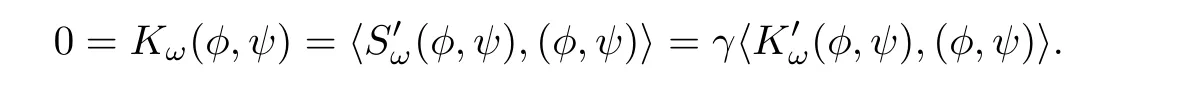
A direct computation shows that
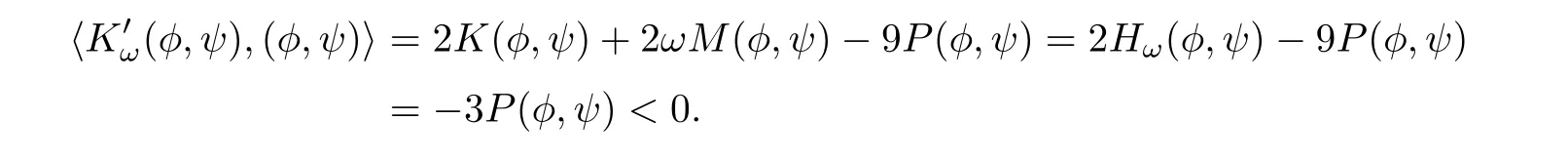
Lemma 2.5
ProofLet(φω,ψω) ∈ Gω.Because Mωis not empty,we take(φ,ψ) ∈ Mω.From Lemma 2.4,we have(φ,ψ) ∈ Gω.Thus,Sω(φω,ψω)=Sω(φ,ψ)=d(ω).It remains to show thatThis implies that
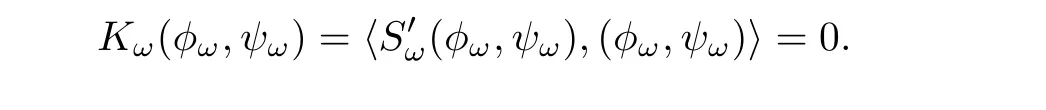
The proof is complete.
Proof of Proposition1.4 The proof of Proposition 1.4 follows immediately from Lemmas 2.3,2.4,and 2.5.
3 Strong Instability of Standing Waves
We are now able to study the strong instability of standing waves for(1.6).Note that the local well-posedness in H1×H1for(1.6)in 5D is given in Proposition 1.2.Let us start with the following so-called Pohozaev’s identities.
Lemma 3.1Let d=5,,and ω >0.Let(φω,ψω)∈ H1×H1be a solution to(1.7).Then,the following identities hold,
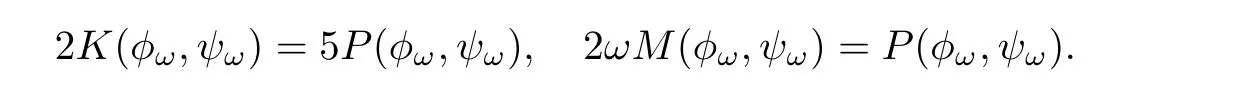
ProofWe only make a formal calculation.The rigorous proof follows from a standard approximation argument.Multiplying both sides of the first equation in(1.7)with,integrating over R5,and taking the real part,we have
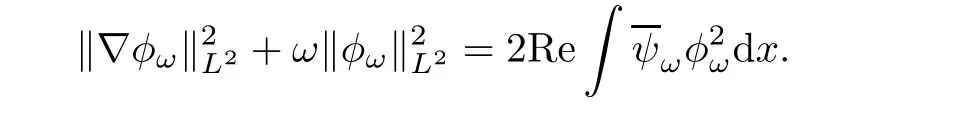
Multiplying both sides of the second equation in(1.7)withintegrating over R5,and taking the real part,we get
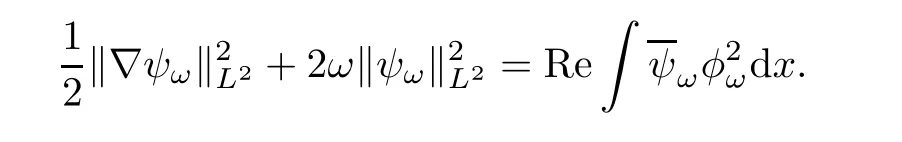
We thus obtain
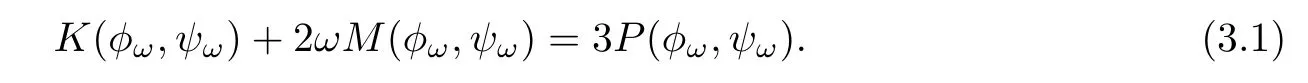
Multiplying both sides of the first equation in(1.7)withintegrating over R5,and taking the real part,we see that
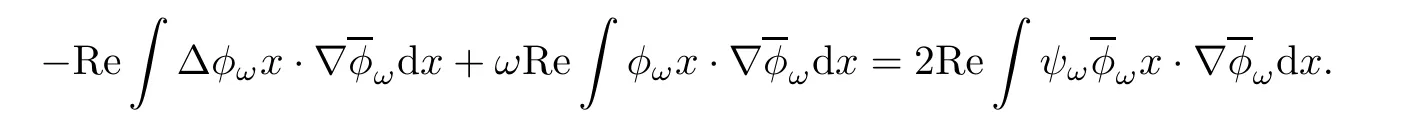
A direct computation shows that
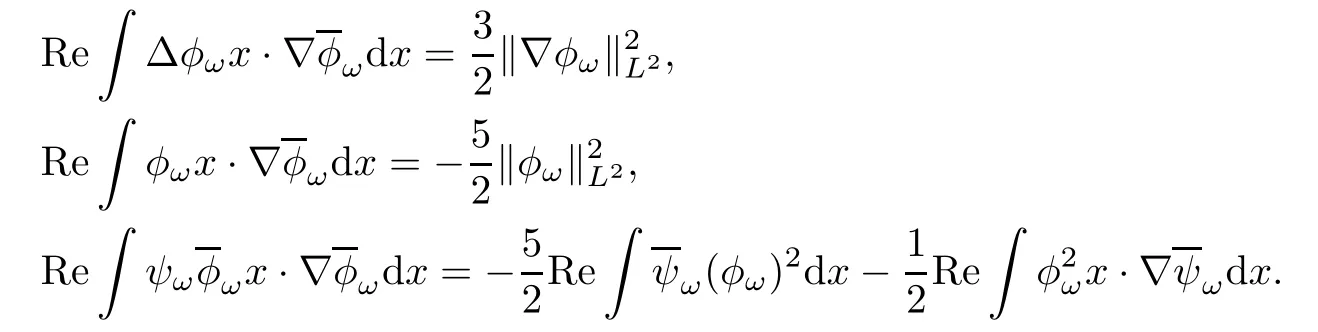
It follows that
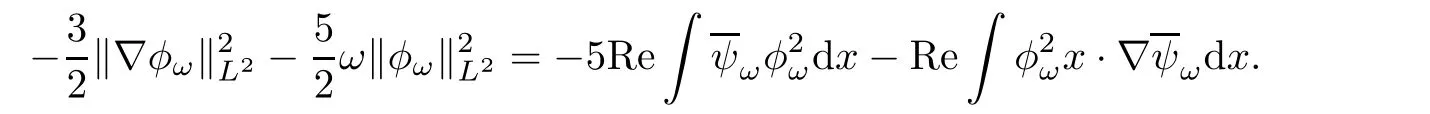
Similarly,multiplying both sides of the second equation in(1.7)withintegrating over R5,and taking the real part,we have
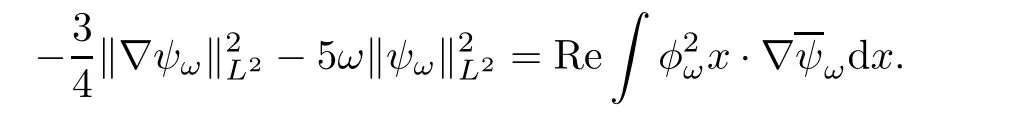
We thus get
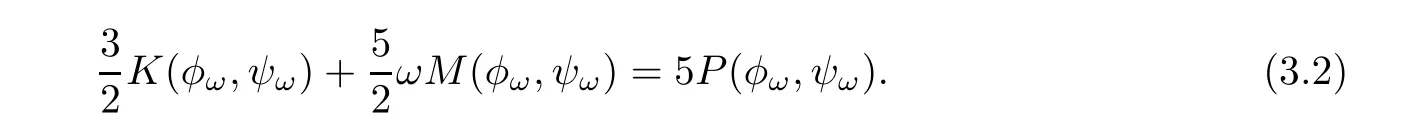
Combining(3.1)and(3.2),we prove the result.
We also have the following exponential decay of solutions to(1.7).
Lemma 3.2Let d=5,and ω >0.Let(φω,ψω)∈ H1×H1be a solution to(1.7).Then,the following properties hold:
? (φω,ψω)∈ W3,p× W3,pfor every 2 ≤ p< ∞.In particular,(φω,ψω)∈ C2× C2andas|x|→ ∞ for all|β|≤ 2;
?
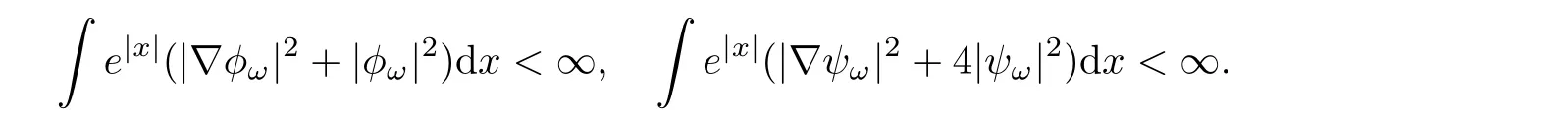
ProofWe follow the argument of[1,Theorem 8.1.1].Let us prove the first item.We note that if(φω,ψω) ∈ Lp× Lpfor some 2 ≤ p< ∞,thenIt follows thatBy Sobolev embedding,we see that
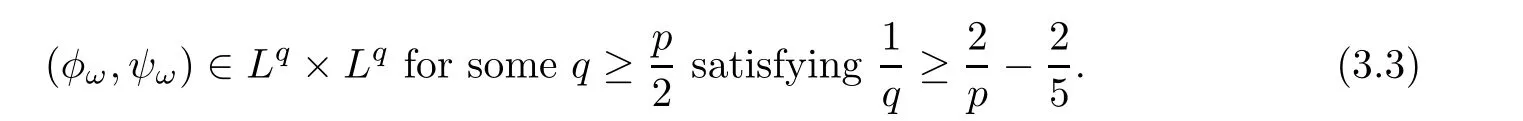
We claim that(φω,ψω)∈ Lp× Lpfor any 2 ≤ p< ∞.As(φω,ψω)∈ H1× H1,the Sobolev embedding implies that(φω,ψω)∈ Lp× Lpfor anyIt remains to show the claim for any p sufficiently large.To see it,we define the sequence
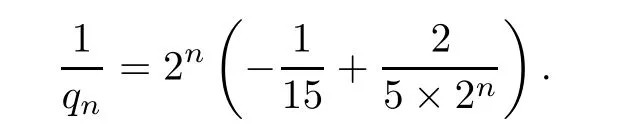
We have
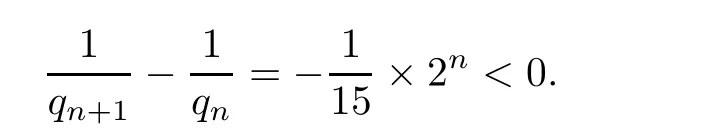
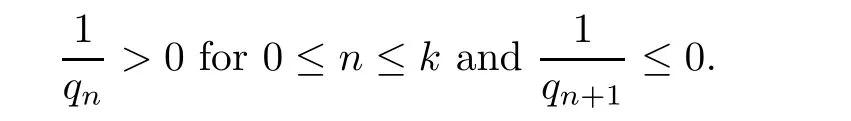
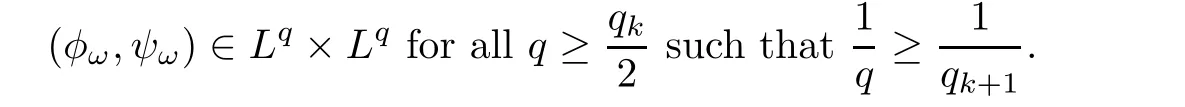
This shows that(φω,ψω)belongs to Lp× Lpfor any p sufficiently large.The claim follows.Using the claim,we have in particularfor any 2 ≤ p< ∞.Hence,(φω,ψω) ∈W2,p× W2,pfor any 2 ≤ p< ∞.By H?lder’s inequality,we see thatfor any 2 ≤ p< ∞ and any 1 ≤ j≤ 5.Thus,for any 2 ≤ p< ∞and any 1≤ j≤ 5,or(φω,ψω)∈ W3,p×W3,pfor any 2≤ p< ∞.By Sobolev embedding,(φω,ψω)∈ C2,δ×C2,δfor all 0< δ<1.In particular,for all|β|≤ 2.

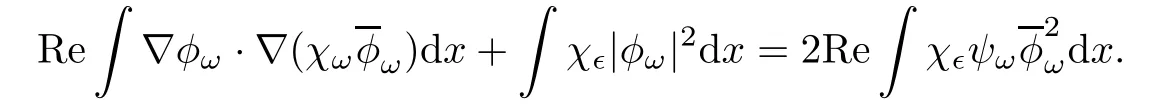
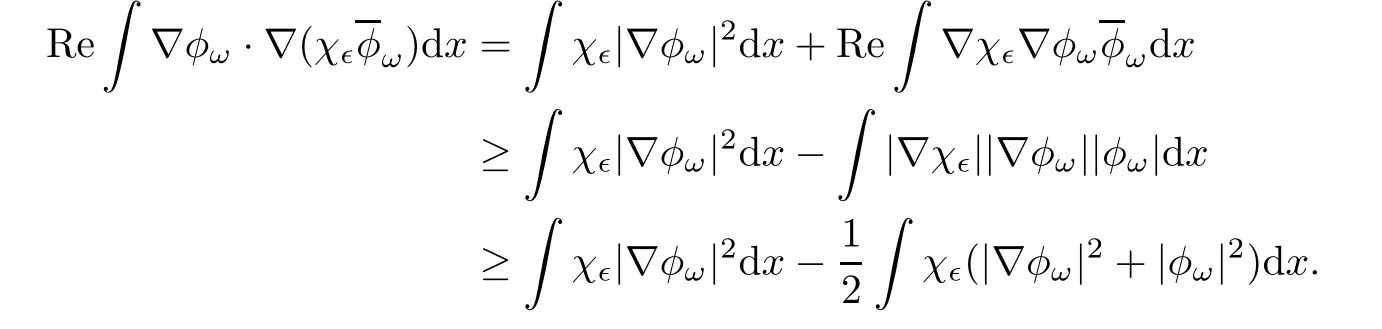
We thus get
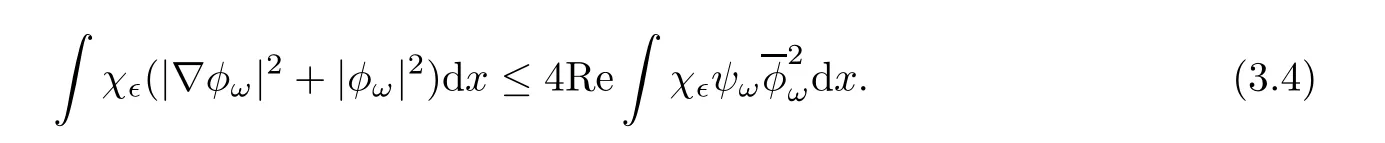
Similarly,multiplying both sides of the second equation in(1.7)withintegrating over R5,and taking the real part,we get
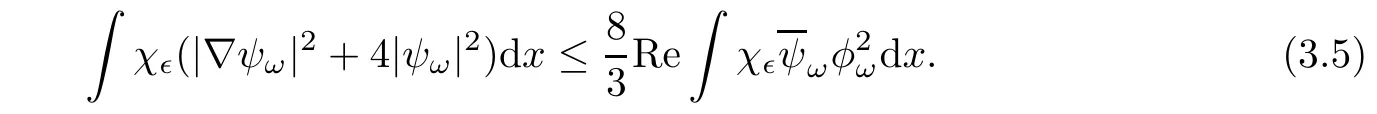
By the first item,there exists R>0 large enough such thatfor|x|≥ R.We have
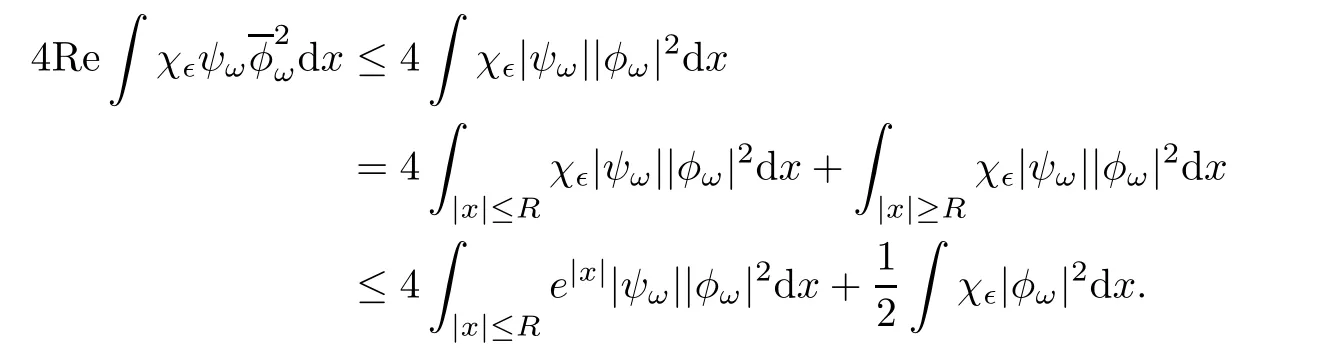
We thus get from(3.4)

Letting ?→ 0,we obtain
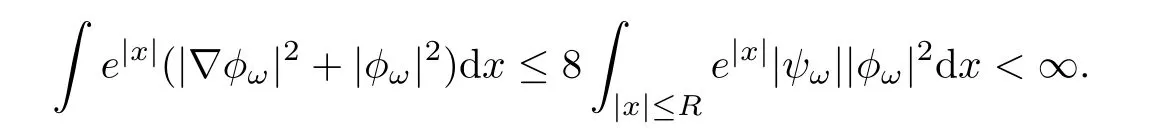
Similarly,by(3.5)and(3.6),
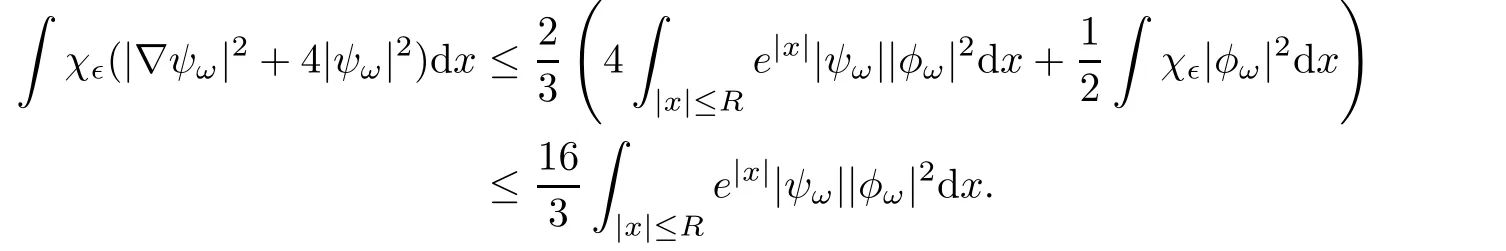
Letting ?→ 0,we get
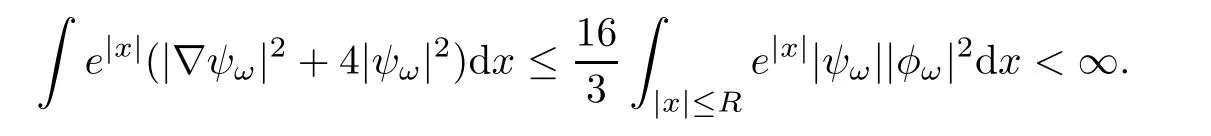
The proof is complete.
We also need the following virial identity related to(1.6).
Lemma 3.3Let d=5 andLet(u0,v0)∈H1×H1be such thatL2×L2.Then,the corresponding solution to(1.6)with initial data(u(0),v(0))=(u0,v0)satisfies
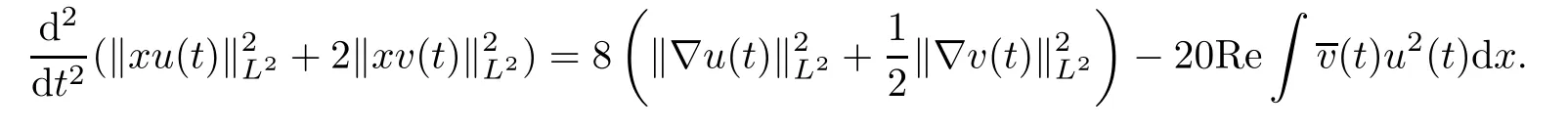
ProofThe above identity follows immediately from[4,Lemma 3.1]with
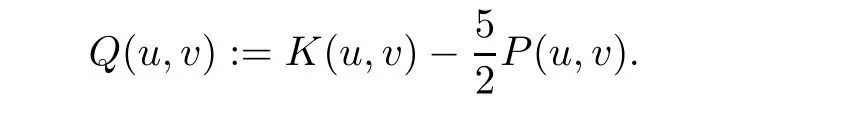
It is obvious that
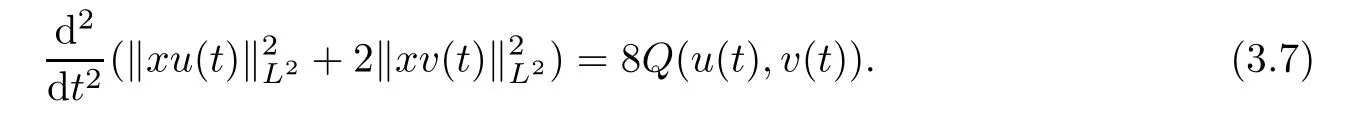
Note that if we take
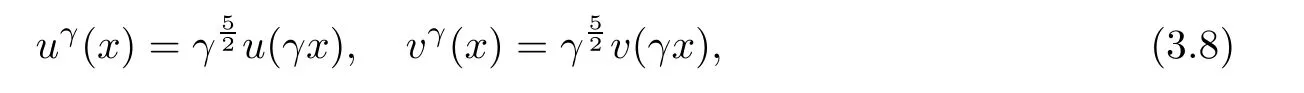
then
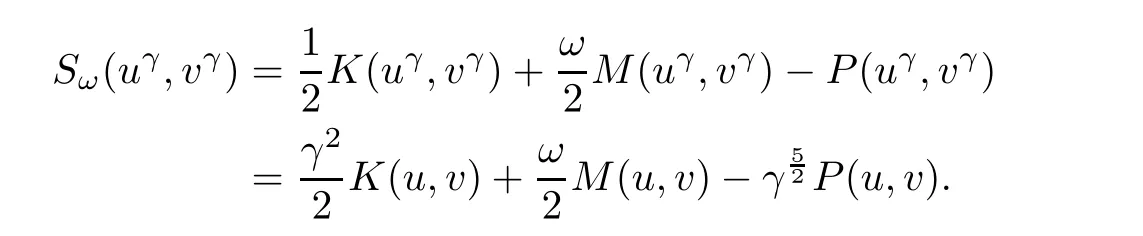
It is easy to see that
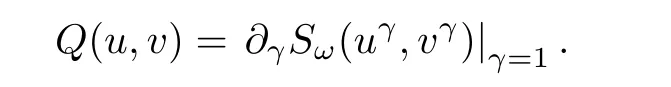
Lemma 3.4Let d=5,and ω >0.Let(φω,ψω)∈ Gω.Then,
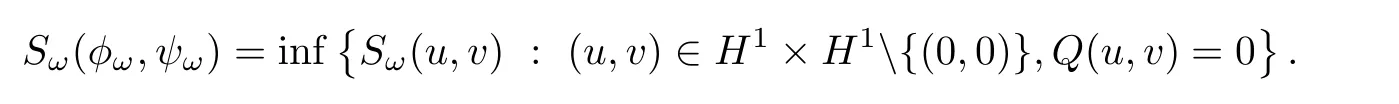
ProofDenote
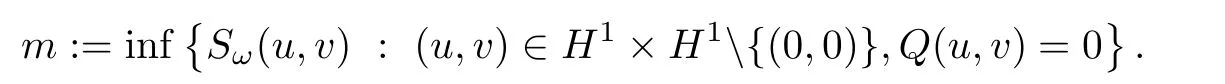
As(φω,ψω)is a solution of(1.7),it follows from Lemma 3.1 that Q(φω,ψω)=Kω(φω,ψω)=0.Thus,

Now,let(u,v)∈ H1× H1{(0,0)}be such that Q(u,v)=0.If Kω(u,v)=0,then by Proposition 1.4,Ifwe consider Kω(uγ,vγ)= γ2K(u,v)+where(uγ,vγ)is as in(3.8).Asandthere exists γ0>0 such that.It again follows from Proposition 1.4,On the other hand,
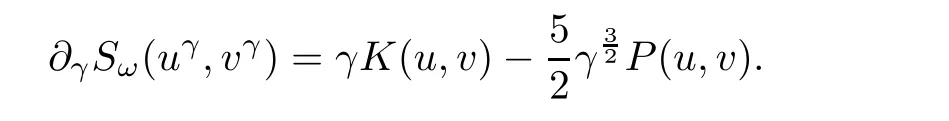
We see that the equation ?γSω(uγ,vγ)=0 admits a unique non-zero solution
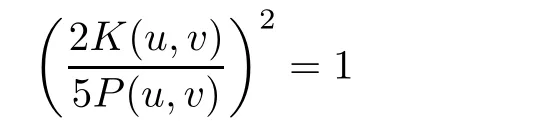
because Q(u,v)=0.This implies thatif γ ∈ (0,1)andif γ ∈ (1,∞).In particular,for all γ >0.Hence,We thus obtainfor any(u,v)∈ H1×H1{(0,0)}satisfying Q(u,v)=0.Therefore,

Combining(3.9)and(3.10),we prove the result.
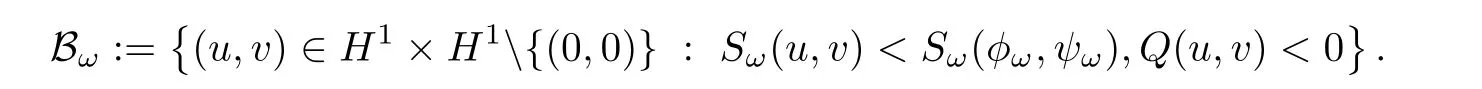
Lemma 3.5Let d=5,ω >0,and.The set Bωis invariant under the flow of(1.6).
ProofLet(u0,v0)∈ Bω.We will show that the corresponding solution(u(t),v(t))to(1.6)with initial data(u(0),v(0))=(u0,v0)satisfies(u(t),v(t))∈Bωfor any t in the existence time.Indeed,by the conservation of mass and energy,we have
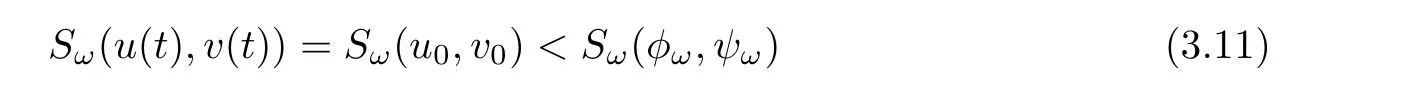
for any t in the existence time.It remains to show that Q(u(t),v(t))<0 for any t as long as the solution exists.Suppose that there exists t0>0 such that Q(u(t0),v(t0))≥0.By the continuity of the function t 7→ Q(u(t),v(t)),there exists t1∈ (0,t0]such that Q(u(t1),v(t1))=0.It follows from Lemma 3.4 that Sω(u(t1),v(t1))≥ Sω(φω,ψω),which contradicts to(3.11).The proof is complete.
Lemma 3.6Let d=5,ω >0,and(φω,ψω)∈ Gω.If(u,v)∈ Bω,then
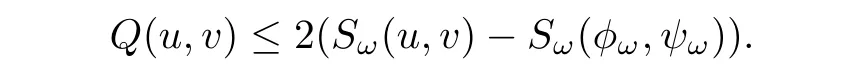
ProofLet(u,v)∈ Bω.Set
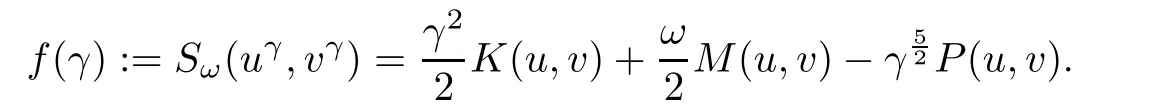
We have
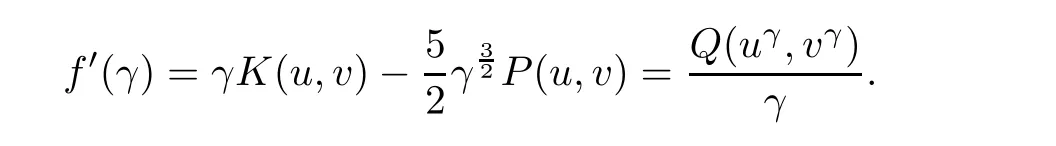
We see that
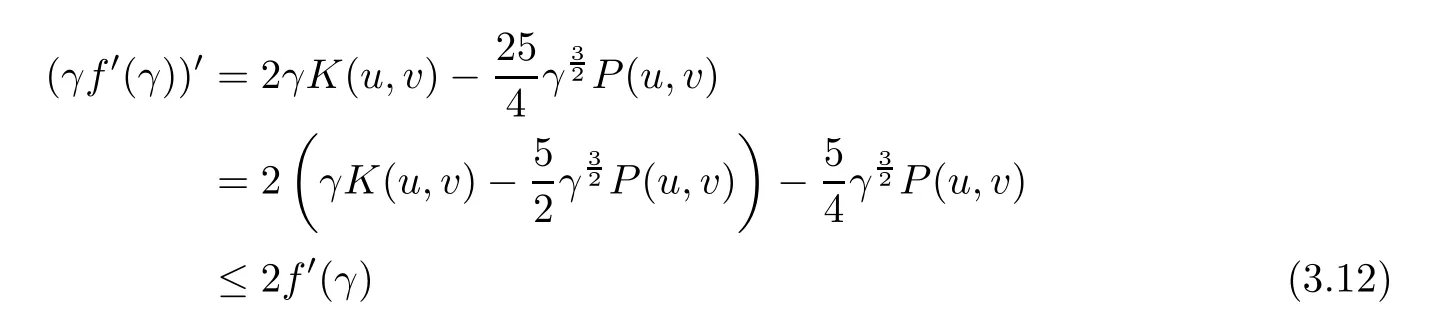
for all γ >0.Note that P(u,v)≥ 0,which follows from the fact Q(u,v)<0.We also note that as Q(u,v)<0,the equationadmits a unique non-zero solution
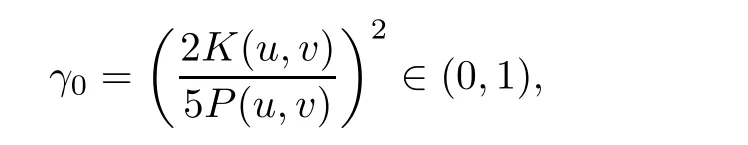
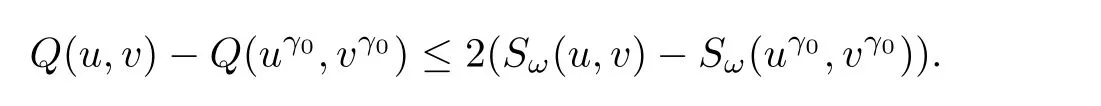
The result then follows from the fact thatas
We are now able to prove the strong instability of standing waves given in Theorem 1.6.
Proof of Theorem1.6Let ?>0.Becauseas γ →1,there exists γ0>1 such thatWe claim that.Indeed,we have

As Q(φω,ψω)=0,the equationadmits a unique non-zero solution
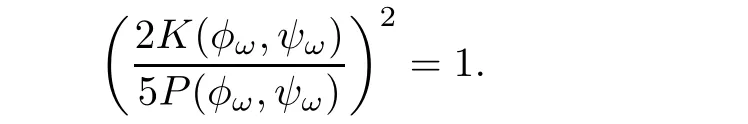
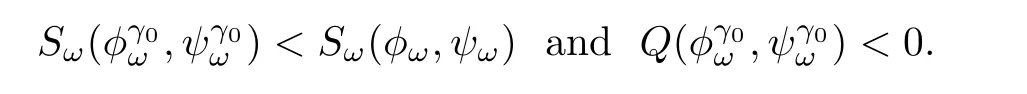
By the local well-posedness,there exists a unique solution(u(t),v(t))∈C([0,T),H1×H1)to(1.6)with initial data(u(0),v(0))=where T>0 is the maximal time of existence.By Lemma 3.5,we see that(u(t),v(t))∈Bωfor any t∈[0,T).Thus,applying Lemma 3.6,we get
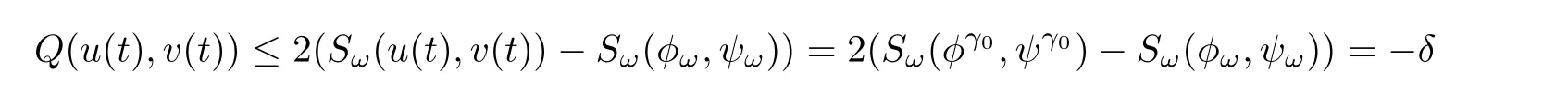
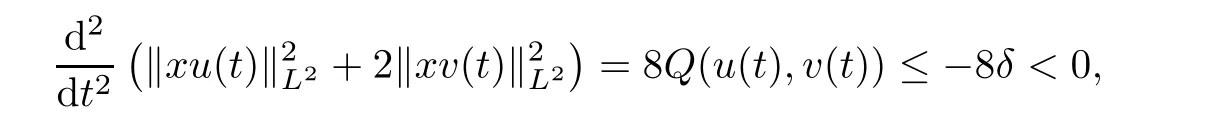
for any t∈[0,T).The classical argument of Glassey[5]implies that the solution blows up in if nite time.The proof is complete.
AcknowledgementsThis work was supported in part by the Labex CEMPI(ANR-11-LABX-0007-01).The author would like to express his deep gratitude to his wife-Uyen Cong for her encouragement and support.
 Acta Mathematica Scientia(English Series)2020年2期
Acta Mathematica Scientia(English Series)2020年2期
- Acta Mathematica Scientia(English Series)的其它文章
- ULAM-HYERS-RASSIAS STABILITY AND EXISTENCE OF SOLUTIONS TO NONLINEAR FRACTIONAL DIFFERENCE EQUATIONS WITH MULTIPOINT SUMMATION BOUNDARY CONDITION?
- EXPANDABLE PARALLEL FINITE ELEMENT METHODS FOR LINEAR ELLIPTIC PROBLEMS?
- PROPERTIES ON MEROMORPHIC SOLUTIONS OF COMPOSITE FUNCTIONAL-DIFFERENTIAL EQUATIONS?
- MAXIMUM TEST FOR A SEQUENCE OF QUADRATIC FORM STATISTICS ABOUT SCORE TEST IN LOGISTIC REGRESSION MODEL?
- INITIAL BOUNDARY VALUE PROBLEM FOR THE 3D MAGNETIC-CURVATURE-DRIVEN RAYLEIGH-TAYLOR MODEL?
- ON THE AREAS OF THE MINIMAL TRIANGLES IN VEECH SURFACES?
