Fano resonance in facile symmetric trimeric Babinet metasurface
, U , , ,
Guangxi Key Laboratory of Precision Navigation Technology and Application, Guilin University of Electronic Technology,Guilin 541004, Guangxi Zhuang Autonomous Region, P.R.China
Abstract: A facile symmetric trimeric Babinet metasurface is proposed for producing a Fano resonance in terahertz region. The Fano resonance is excited by an incident wave that interferes with the out-of-phase dark mode when a linearly polarized wave perpendicular to the Babinet slits illuminates the metasurface. By adjusting the structural parameters of the metasurface, the in-phase bright mode can be tuned independently, while the out-of-phase dark mode can be changed linearly, and the quality factor can also be tuned. Overall, a method for tuning the dark and bright modes for Fano resonance is revealed. The tuning mechanism has promise for applications in various fields such as sensors, filters, optical switches, photodetectors, and energy-harvesting devices with advanced performance.
Key words: terahertz device; Babinet metasurfaces; Fano resonance; tunable characteristic; bright mode; dark mode
Two-dimensional metamaterials, known as metasurfaces, have recently emerged as a novel research frontier, since they are significantly capable of tailoring electromagnetic wave with ultrathin thickness at will[1-6]. These remarkable surfaces are composed of two-dimensional arrays of polarizable particles, which can be arranged in a variety of topological structures to create various functionalities, such as negative refractive index[7-8], anomalous reflection[9-11], gradient-index[12-13], resonance-backed[14-15], and versatile holograms[16-18]. When it comes to resonance, the phenomenon of Fano resonance, which is due to interference between a narrow discrete resonance and a broad spectral line[19], has drawn much attention in various fields such as slow light[20-21], sensing[22- 23]and nano-lasing[24-25]. In order to activate the Fano resonance in a metasurface, one usually employs a symmetry-breaking structure such as asymmetric split-rings[26-27], detuned plasmonic and dielectric resonator-pairs[28-29]or dipole-quadrupole coupled structures[30-31]. Further tailoring the Fano resonance via plasmonic nanoclusters[32], nonlinear materials[33]and phase-change materials[34]not only brings about a host of intriguing physical phenomena[35-38], but also has multiple applications[20, 39]. Recently, researchers have found that Fano resonances exist widely in symmetric structures such as nanoscale plasmonic clusters and nano-shells, but their configurational requirements are complicated.
In this paper, we propose a facile symmetric trimeric metasurface array made up of multiple unit cells, each of them consisting of three rectangular Babinet slits, which act as dipolar resonators under the influence of a perpendicular incident plane wave. Finite element method simulations were carried out to obtain a series of parametric results, which indicate that disciplinary redshift and blueshift occur in the transmission spectrum with the altering of certain structural parameters. These results provide an ideal pathway to fabricate a compact, efficient terahertz device based on Fano resonance.
1 Schematic and physical model
Fig.1(a) shows the schematic diagram of the trimeric Babinet metasurface array model. Each unit cell of metasurface structure settled in vacuum is made up of perfect electric conductor (PEC) with periodpx=py=50 μm. Each of the three rectangular slits of the unit cell has lengthhand widthw. The spacing between adjacent slits isd. The incident electromagnetic wave is transmitted along the wave vectorkwhich is perpendicular to the surface. The incident plane waveExwith electric field parallel to thexaxis propagates along thekdirection as indicated in
Fig.1(a). Fano resonance and field properties illustrated in
Fig.1(b) were obtained via simulation.

Fig.1 Trimeric Babinet metasurface and related transmission spectra圖1 三孔縫Babinet超表面與相關(guān)透射譜
Fano resonance derives from the interference between a radiative bright mode and a sub-radiative dark mode[32]. For the sake of elucidating the principle of Fano resonance, we first explore the features of a typical Fano resonance through simulation. A transmittance spectrum exhibiting Fano resonance is shown in
Fig.1(b), and was obtained by setting the default parameters as follows:w=6 μm,h=30 μm,d=10 μm,px=py=p=50 μm. The predicted transmission coefficient decreases from 0.996 to 0.015 in the frequency range of 4.44-4.46 THz. However, in the range from 4.46 to 5.20 THz, the transmission coefficient increases slowly from 0.015 to 0.999. The Fano resonance possesses high asymmetry and an extremely sharp line, which is a distinct feature different from other resonances, and this significant characteristic derives from the interference between the out-of-phase dark mode at ‘1’ and the in-phase bright mode at ‘2’ in
Fig.1(b). The upper left inset illustratesExfor the dark mode appearing in the Fano dip signified by ‘1’, which shows that the field has the out-of-phase(+-+) distribution in the slits. The distribution ofExin the bright mode denoted by ‘2’ is shown in the lower right inset; this field has the in-phase(---) distribution. A Fano resonance is produced by wide-band bright mode interfering with an out-of-phase field profile dark mode[31]. The specified structural parameters were tuned to illustrate the properties of the bright and dark modes in detail.
2 Results and discussion
The evolution of the transmission spectra of the trimeric unit cell was investigated by altering the width parameterwwhile fixing the other parameters asd=10 μm,h=30 μm, andp=50 μm. The transmittance as a function ofwis shown in
Fig.2(a). The narrow blue line corresponds to the dark mode at 4.41 THz at the dip point, and the broad red area represents the bright mode. Aswis increased, the broad transmission peak shows a slight blueshift, while the narrow transmission dip remains almost fixed at 4.41 THz. This illustrates that the position of the dark mode relative to the bright mode can be independently adjusted by the slit width.
Fig.2(b) and 2(c) show transmission spectra and quality factors respectively for four different widths (w=5.2, 5.8, 6.4 and 7.0 μm). With increasingw, the frequency of the dark mode (indicated by the black dashed line) stays unchanged, while the bright mode denoted by the pink dashed line shows a slight blueshift. In
Fig.2(c), the quality factor (Q-factor) for the Fano resonance decreases from 20.90 (corresponding tow=5.2 μm ) to 16.92 (corresponding tow=7.0 μm). Therefore, we can enhance theQ-factor for the Fano resonance by loweringw.
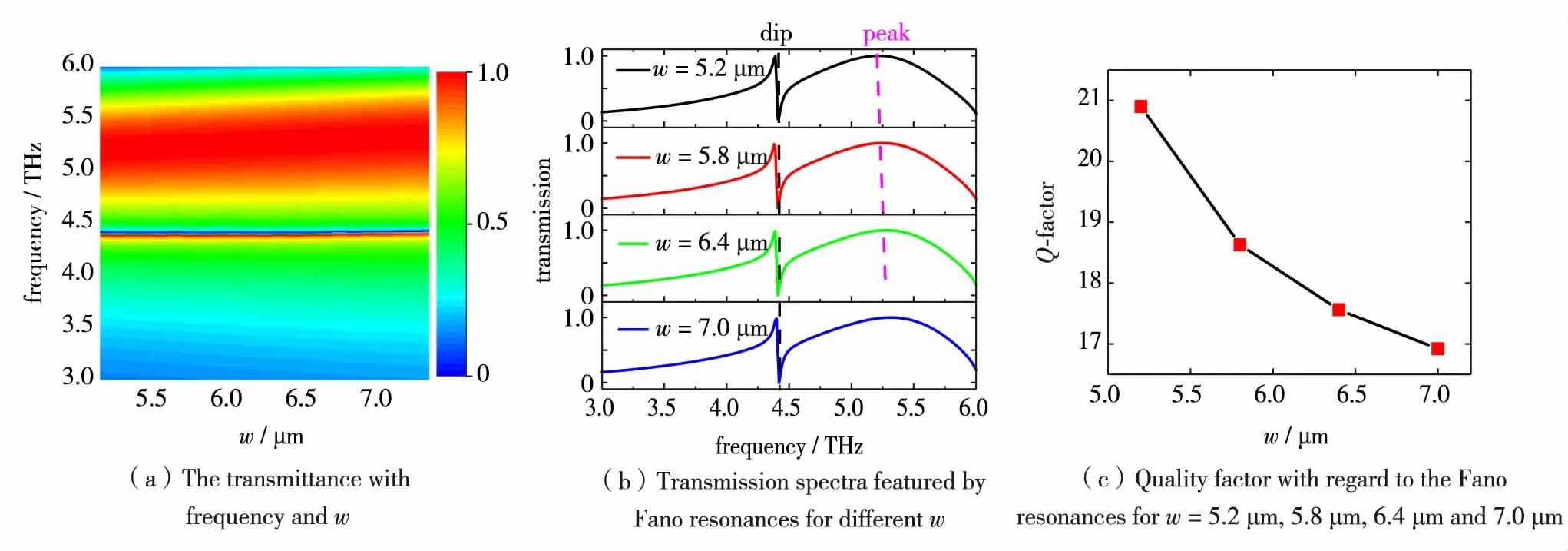
Fig.2 Related data about altering w圖2 改變w的相關(guān)數(shù)據(jù)
The dependence of the Fano resonance on the inter-slit spacingdwas also investigated, fixing the other parameters asw=6 μm,h=30 μm, andp=50 μm.
Fig.3(a) shows the simulated transmittance as a function ofd. In this case, the sharp transmission dip corresponding to the dark mode, manifested by the blue belt in the range 5.06-5.33 THz (corresponding tod=7.3-13.0 μm), shows a redshift with increasingd. Conversely, the red area in the range 5.59 - 6.00 THz (corresponding tod=7.3-12.7 μm), which corresponds to that the bright mode is blueshifted with increasingd.
Fig.3(b) and 3(c) show transmission spectra and quality factors respectively for four different spacings (d=8, 9, 10 and 11 μm). With increasingd, the dark mode denoted by the pink and black dashed lines shows an almost linear redshift, while the bright mode denoted by the red dashed line shows a blueshift, and theQ-factor increases from 11.24 (corresponding tod=8 μm) to 35.28 (corresponding tod=11 μm). Increasingdresults in enhancement of the quality factor for the Fano resonance.
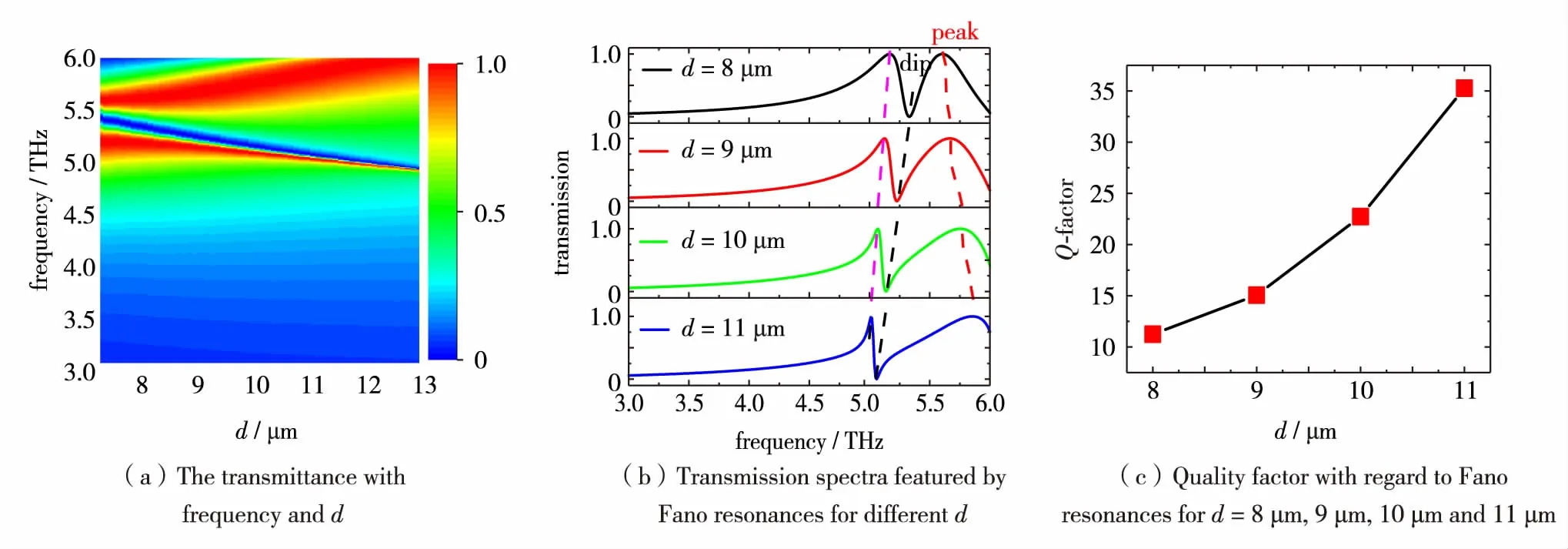
Fig.3 Related data about altering d圖3 改變d的相關(guān)數(shù)據(jù)
Fig.4(a) shows the influence on the Fano resonance due to altering the slit lengthhwhile fixing the other parameters asd=10 μm,w=6 μm andp=50 μm. In this case, the sharp transmission dip (dark mode) indicated by the blue area in the range 5.87-3.25 THz (h=20-42 μm) shows a redshift with increasingh; and the wide transmission peak (bright mode) corresponding to the red area ranging from 5.98 to 4.29 THz (h=23-42 μm) is also redshifted ashis increased.
Fig.4(b) and 4(c) respectively show transmission spectra and quality factors for four different lengths (h=22, 26, 30 and 34 μm). With increasingh, the peak denoted by a pink dashed line shows redshift, and the dark (bright) mode denoted by a black (red) dashed line also shows redshift. TheQ-factor decreases from 22.875 (corresponding toh=22 μm) to 12.200 (corresponding toh=34 μm). Making the slits longer results in a lower quality factor for the Fano resonance.
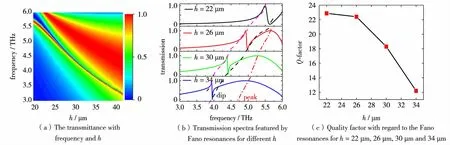
Fig.4 Related data about altering h圖4 改變h的相關(guān)數(shù)據(jù)
3 Conclusions
To sum up, we present a model of a facile symmetric trimeric Babinet metasurface cell made up of three dipolar resonators. The genetic mechanism underlying the Fano resonance is revealed by simulating the transmitted electric field component distributions and transmission spectrum. The radiative bright mode and sub-radiative dark mode for the Fano resonance are affected by adjusting three structural parameters. The bright mode can be tuned independently by varying the slit widthw. Linear tuning of the dark mode with increasingdand redshift for both modes of Fano resonance are obtained by parametric analysis.Q-factors of Fano resonance are also predicted, as they may be relevant to potential future applications. This structure has promise in various devices such as sensors, filters, optical switches, photodetectors, and energy-harvesting devices with advanced performance.
References/參考文獻(xiàn):
[1] GLYBOVSKI S B, TRETYAKOV S A, BELOV P A, et al. Metasurfaces: from microwaves to visible[J]. Physics Reports, 2016, 634(24): 1-72.
[2] ZHELUDEV N I, KIVSHAR Y S. From metamaterials to metadevices[J]. Nature Materials, 2012, 11(11): 917-924.
[3] MONTICONE F, ALU A. Metamaterial, plasmonic and nanophotonic devices[J]. Reports on Progress in Physics, 2017, 80(3): 036401.
[4] CHEN Houtong, TAYLOR A J, YU Nanfang. A review of metasurfaces: physics and applications[J]. Reports on Progress in Physics Physical Society, 2016, 79(7): 076401.
[5] DENG Zilan, ZHANG Shuang, WANG Guoping. A facile grating approach towards broadband, wide-angle and high-efficiency holographic metasurfaces[J]. Nanoscale, 2016, 8(3): 1588-1594.
[6] DENG Zilan, ZHANG Shuang, WANG Guoping. Wide-angled off-axis achromatic metasurfaces for visible light[J]. Optics Express, 2016, 24(20): 23118-23128.
[7] PENDRY J B. Negative refraction makes a perfect lens[J]. Physial Review Letters, 2000, 85(18): 3966-3969.
[8] PANOIU N C, OSGOOD R M. Numerical investigation of negative refractive index metamaterials at infrared and optical frequencies[J]. Optics Communications, 2003, 223(4/5/6): 331-337.
[9] PU Mingbo, CHEN Po, WANG Chuangtao, et al. Broadband anomalous reflection based on gradient low-Q meta-surface[J]. AIP Advances, 2013, 3(5): 052136.
[10] SUN Shulin, YANG Kuangyu, WANG C M, et al. High-efficiency broadband anomalous reflection by gradient meta-surfaces[J]. Nano Letters, 2012, 12(12): 6223-6229.
[11] DENG Zilan, LI Xiangping, WANG Guoping. A multifunctional metasurface: from extraordinary optical transmission to extraordinary optical diffraction in a single structure[DB/OL]. (2017-05-27)[2018-12-01]. https://arxiv.org/abs/1705.10171
[12] SMITH D R, MOCK J J, STARR A F, et al. Gradient index metamaterials[J]. Physical Review E: Statistical, Nonlinear, and Soft Matter Physics, 2005, 71(3 Pt 2B): 036609.
[13] HOANG T V, LEE J H. Generation of multi-beam reflected from gradient-index metasurfaces[J]. Results in Physics, 2018, 10: 424-426.
[14] LUK’YANCHUK B, ZHELUDEV N I, MAIER S A, et al. The Fano resonance in plasmonic nanostructures and metamaterials[J]. Nature Materials, 2010, 9(9): 707-715.
[15] DENG Zilan, YOGESH N, CHEN Xiaodong, et al. Full controlling of Fano resonances in metal-slit superlattice[J]. Scientific Reports, 2015, 5:18461.
[16] DENG Zilan, LI Guixin. Metasurface optical holography[J]. Materials Today Physics, 2017, 3:16-32.
[17] DENG Zilan, DENG Junhong, ZHUANG Xin, et al. Diatomic metasurface for vectorial holography[J]. Nano Letters, 2018, 18(5): 2885-2892.
[18] DENG Zilan, DENG Junhong, ZHUANG Xin, et al. Facile metagrating holograms with broadband and extreme angle tolerance[J]. Light: Science & Applications, 2018, 7(1): 78.
[19] LIU Zhonghui, YE Jian. Highly controllable double Fano resonances in plasmonic metasurfaces[J]. Nanoscale, 2016, 8(40): 17665-17674.
[20] WU C, KHANIKAEV A B, SHVETS G. Broadband slow light metamaterial based on a double-continuum Fano resonance[J]. Physical Review Letters, 2011, 106(10): 107403.
[21] PAPASIMAKIS N, ZHELUDEV N I. Metamaterial-induced transparency: sharp Fano resonances and slow light[J]. Optics and Photonics News, 2009, 20(10): 22-27.
[22] ZHAN Yaohui, LEI Dangyuan, LI Xiaofei, et al. Plasmonic Fano resonances in nanohole quadrumers for ultra-sensitive refractive index sensing[J]. Nanoscale, 2014, 6(9): 4705-4715.
[23] TU Zhengrui, GAO Dingshan, ZHANG Meiling, et al. High-sensitivity complex refractive index sensing based on Fano resonance in the subwavelength grating waveguide micro-ring resonator[J]. Optics Express, 2017, 25(17): 20911-20922.
[24] ZHELUDEV N I, PROSVIRNIN S L, PAPASIMAKIS N, et al. Lasing spaser[J]. Nature Photonics, 2008, 2(6): 351-354.
[25] DENG Zilan, DONG Jianwen. Lasing in plasmon-induced transparency nanocavity[J]. Optics Express, 2013, 21(17): 20291-20302.
[26] WANG Feng, WANG Zhengping, SHI Jinhui. Theoretical study of high-Q Fano resonance and extrinsic chirality in an ultrathin Babinet-inverted metasurface[J]. Journal of Applied Physics, 2014, 116(15): 153506.
[27] CONG Longqing, MANJAPPA M, XU Ningning, et al. Fano resonances in terahertz metasurfaces: a
Figure of merit optimization[J]. Advanced Optical Materials, 2015, 3(11): 1537-1543.
[28] BOZHEVOLNYI S I, EVLYUKHIN A B, PORS A, et al. Optical transparency by detuned electrical dipoles[J]. New Journal of Physics, 2011, 13(2): 023034.
[29] YAN Jiahao, LIU Pu, LIN Zhaoyong, et al. Directional Fano resonance in a silicon nanosphere dimer[J]. ACS Nano, 2015, 9(3): 2968-80.
[30] ZHANG Shuang, GENOV D A, WANG Yuan, et al. Plasmon-induced transparency in metamaterials[J]. Physical Review Letters, 2008, 101(4): 047401.
[31] DENG Zilan, FU Tao, OUYANG Zhengbiao, et al. Trimeric metasurfaces for independent control of bright and dark modes of Fano resonances[J]. Applied Physics Letters, 2016, 108(8): 081109.
[32] LASSITER J B, SOBHANI H, KNIGHT M W, et al. Designing and deconstructing the Fano lineshape in plasmonic nanoclusters[J]. Nano Letters, 2012, 12(2): 1058-1062.
[33] ZHU Yu, HU Xiaoyong, HUANG Yongyang, et al. Fast and low-power all-optical tunable Fano resonance in plasmonic microstructures[J]. Advanced Optical Materials, 2013, 1(1): 61-67.
[34] CAO Tun, WEI Chenwei, SIMPSON R E, et al. Fast tuning of double Fano resonance using a phase-change metamaterial under low power intensity[J]. Scientific Reports, 2014, 4: 4463.
[35] TASSIN P, ZHANG Lei, ZHAO Rongkuo, et al. Electromagnetically induced transparency and absorption in metamaterials: the radiating two-oscillator model and its experimental confirmation[J]. Physical Review Letters, 2012, 109(18): 187401.
[36] VERSLEGERS L, YU Zhongfu, RUAN Zhichao, et al. From electromagnetically induced transparency to superscattering with a single structure: a coupled-mode theory for doubly resonant structures[J]. Physical Review Letters, 2012, 108(8): 083902.
[37] PENG Bo, OZDEMIR S K, CHEN Weijian, et al. What is and what is not electromagnetically induced transparency in whispering-gallery microcavities[J]. Nature Communications, 2014, 5: 5082.
[38] WAN Weiwei, ZHENG Wenwei, CHEN Yanfeng, et al. From Fano-like interference to superscattering with a single metallic nanodisk[J]. Nanoscale, 2014, 6(15): 9093-9102.
[39] WU C, KHANIKAEV A B, ADATO R, et al. Fano-resonant asymmetric metamaterials for ultrasensitive spectroscopy and identification of molecular monolayers[J]. Nature Materials, 2011, 11(1): 69-75.

