周平,李耀堂

(1.文山學(xué)院數(shù)理系,云南 文山 663000;2.云南大學(xué)數(shù)學(xué)與統(tǒng)計學(xué)院,云南 昆明 650091)

非負(fù)矩陣Hadam ard積和M-矩陣Fan積的特征值界的估計

周平1,李耀堂2

(1.文山學(xué)院數(shù)理系,云南 文山 663000;2.云南大學(xué)數(shù)學(xué)與統(tǒng)計學(xué)院,云南 昆明 650091)

矩陣的Hadamard積和Fan積是矩陣?yán)碚撗芯康闹匾獑栴}之一.對于兩個非負(fù)矩陣A和B的Hadamard積,給出了它的譜半徑上界的兩個新的估計式;同時對于兩個非奇異M-矩陣A和B的Fan積,給出了它的最小特征值下界的兩個新的估計式;算例表明,所得估計式在某些情況下比現(xiàn)有估計式更為精確,并且這些估計式都只依賴于矩陣A和B的元素,更容易計算.

非負(fù)矩陣;M-矩陣;Hadamard積;Fan積;譜半徑;最小特征值

1 預(yù)備知識

2 兩個非負(fù)矩陣的H adam ard積的譜半徑的上界

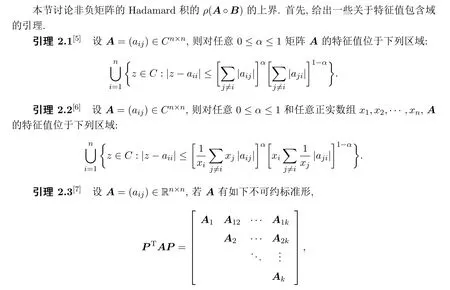
3 q(A?B)的下界

參考文獻

[1]陳景良,陳向暉.特殊矩陣[M].北京:清華大學(xué)出版社,2000.

[2]程光輝,成孝予,黃廷祝.M-矩陣和H-矩陣在Fan積下的Oppenheim型不等式[J].純粹數(shù)學(xué)與應(yīng)用數(shù)學(xué), 2006,22(2):253-255.

[3]Horn R A,Johnson C R.Topic in M atrix Analysis[M].New York:Cambridge University Press,1991.

[4]楊忠鵬,馮曉霞.兩個 Herm itian矩陣的 Hadamard乘積的特征值估計 [J].純粹數(shù)學(xué)與應(yīng)用數(shù)學(xué),2001, 17(1):86-89.

[5]Ljiljana Cvetkovic.H-m atrix theory and eigenvalue localization[J].Num er.A lgor.,2006,42:229-245.

[6] 李艷艷,李耀堂.矩陣 Hadamard積和 Fan積的特征值界的估計 [J].云南大學(xué)學(xué)報:自然科學(xué)版, 2010,32(2):125-129.

[7]Berman A,Plemmons R J.Nonnegative Matrices in the Mathematical Sciences[M].New York:Academ ic Press,1979.

[8]Fang M Z.Bounds on eigenvalues of Hadamard product and the Fan product ofmatrices[J].Linear A lgebra and its App lications,2007,425:7-15.

[9]Huang R.Som e inequalities for the Hadam ard product and the Fan product ofm atrices[J].Linear A lgebra and its App lications,2008,428:1551-1559.

[10]Liu Q B,Chen G L.On two inequalities for the Hadam ard p roduct and the Fan p roduct of m atrices[J]. Linear A lgebra and its App lications,2009,431:974-984.

[11]Li Y T,Li Y Y,W ang R W,et al.Som e new bounds on eigenvalues of the Hadam ard product and the Fan p roduct ofmatrices[J].Linear A lgebra and its App lications,2010,432:536-545.

[12]Horn R A,Johnson C R.Topic in M atrix Analysis[M].Beijing:Peop le′s Posts and Telecommunications Press,2005.

Estimating of bounds on eigenvalues of the H adam ard p roduct for nonnegative m atrices and the Fan p roduct of M-m atrices

Zhou Ping1,Li Yaotang2

(1.School of M athem atics and Physics,Wenshan University,Wenshan 663000,China; 2.School of M athem atics and Statistics,Yunnan University,Kunm ing 650091,China)

The Hadamard p roduct and the Fan product ofmatrices are im portant prob lem s in thematrices theories.For the Hadam ard product of two nonnegative m atrices A and B,two new upper bounds of the spectral radius are given.For the Fan p roduct of two M-m atrices A and B,two new lower bounds of the smallest eigenvalues are given.The given numerical exam p les show that these estimating formulas im prove several existing resu lts in som e cases,and these bounds are easier to calcu late for they are on ly depending on the entries ofm atrices A and B.

nonnegativematrix,M-matrix,Hadamard product,Fan p roduct,spectral radius, sm allest eigenvalue

O151.21

A

1008-5513(2012)06-0826-08

2011-12-03.

國家自然科學(xué)基金(10961027).

周平(1987-),碩士,助教,研究方向:矩陣?yán)碚摷捌鋺?yīng)用研究.

李耀堂(1958-),博士,教授,研究方向:數(shù)值計算及其應(yīng)用研究.

2010 M SC:15A 42,15A 69