Structural,electronic and magnetic properties of Fe-doped strontium ruthenates
Nan Liu(劉楠), Xiao-Chao Wang(王曉超), and Liang Si(司良),2,?
1School of Physics,Northwest University,Xi’an 710127,China
2Institute of Solid State Physics,TU Wien,Vienna 1040,Austria
Keywords: first-principles calculations,double perovskites,correlation effects,dynamical mean-field theory
1.Introduction
Perovskites oxides[1,2]have emerged as key players in hosting a wide range of exotic physical phenomena, including high-TCsuperconductivity,[3]ferroelectricity[4,5]and Mott-insulator transition.[6,7]Among these oxides, strontium ruthenate stands out as one of the most extensively investigated strongly correlated perovskite materials due to its remarkable physical properties.[8,9]Two significant classes of ruthenates, namely (double) perovskites SrRuO3(SrRuBO6)and Ruddlesden–Popper-type layered Srn+1RunO3n+1, have recently gained prominence as promising candidates for various technological applications.The intricate interplay among crystal distortions, lattice effects, spin and orbital degrees of freedom, electronic correlations, and spin–orbital coupling in SrRuO3and Sr2RuO4gives rise to extraordinary physical states.These include anomalous Hall effects and the presence of magnetic monopoles,[10]skyrmion states,[11–14]and unconventional chiral p-wave superconductivity, along with other fascinating properties.[9,15–19]However,due to the moderately strong correlations of Ru-4d orbitals, the observability of the important physical phenomena in ruthenates is limited compared to the 3d transition metal oxides(TMOs).For instance,the ferromagnetic Curie temperature in perovskite SrRuO3is around 150 K–160 K,[8]and the critical temperature for superconductivity in Sr2RuO4is approximately 1 K.[9]
In comparison to 4d and 5d TMOs, 3d TMOs consistently exhibit higher Curie or N′eel temperatures.Examples include Fe2O3,[20]LaFeO3,[21]and La2/3Sr(Ca)1/3MnO3.[22]Previous experimental studies have shown that incorporating chemical doping techniques with (double) perovskite structures can lead to the development of new materials with novel properties, making them highly desirable for various technological applications.[23,24]Consequently, the physical properties of both SrRuO3and Sr2RuO4have been found to be sensitive to material engineering techniques such as chemical doping,[25–28]strain,[29]and external field effects.[30,31]By manipulating these factors,modifications to the electronic structure and magnetic ordering in ruthenates can be induced.This includes the emergence of magnetic competition,[32]reshaping of electronic statesN(Ef) near the Fermi level,[33–35]occurrence of crystal orthorhombic distortion,[25,36]and positioning of the Fermi surface in closer proximity to the Van Hove singularity of theγ-band.[37]These controls allow for the fine-tuning of the electronic and magnetic properties in ruthenates,offering opportunities for tailoring materials for specific applications.
The magnetic transition and operational temperature of 4d transition metal oxides(TMOs),such as ruthenates,can be naturally expected to be enhanced by the controlled incorporation of 3d transition metal dopants like Fe.The doping concentration can be precisely regulated by adjusting the doping amount and processing parameters.In the context of epitaxial growth of perovskite oxides,Fe doping refers to the intentional addition of iron (Fe) atoms to theB-site ofABO3perovskite oxide materials.Various methods, such as solid-state reactions and atomic layer deposition,can be employed to achieve Fe doping.Furthermore, Fe doping is anticipated to induce charge transfer phenomena[38]between Fe-3d and Ru-4d orbitals, resulting in unexpected exotic phenomena.[39]Due to the difference in electronegativity, electrons from the Ru-4d orbitals are expected to transfer to Fe-3d states,leading to the formation of Fe-3d5and Ru-4d3configurations.[40]This sets the stage for possible interactions between the possible highspin Fe and Ru sites.Therefore, conducting further investigations into the electronic and magnetic structures of heavily Fe-doped SrRuO3and Sr2RuO4holds crucial importance and offers fundamental insights into these systems.
This study aims to investigate the structural, magnetic,and electronic properties, as well as correlation effects of heavy Fe-doping in SrRuO3and Sr2RuO4through DFT and DMFT calculations.We present a comparative analysis of the structural,electronic,and magnetic characteristics of both systems.The introduced Fe atoms are found to be distributed separately within SrRuO3and Sr2RuO4.DFT+Ucalculations reveal a weakened hybridization between Fe-3d and Ru-4d orbitals, leading to a significant charge transfer from Ru to Fe.Consequently, the Fe atoms adopt a Fe3+state while the Ru atoms adopt a Ru5+state.The presence of electronic correlations within both Fe-3d and Ru-4d orbitals results in substantial magnetic moments, giving rise to strong superexchange effect and the formation of ferrimagnetic(FIM)orders in SrFe0.5Ru0.5O3and Sr2Fe0.5Ru0.5O4.The Hund’s exchange interaction plays a crucial role in the establishment of high-spin states for both Fe3+and Ru5+ions.To account for dynamical correlation effects, DFT+DMFT calculations are further performed, demonstrating that the FIM states in SrFe0.5Ru0.5O3and Sr2Fe0.5Ru0.5O4persist even at room temperature.
2.Method
DFT structural relaxations are performed to reach the ground state structures for SrFe0.5Ru0.5O3and Sr2Fe0.5Ru0.5O4.After obtaining the ground structures with lowest DFT total energy, static calculations of band, densityof-states (DOS) are performed using the VASP[41,42]and WIEN2K[43,44]codes with the Perdew–Burke–Ernzerhof version of the generalized gradient approximation (GGAPBE)[45]and a dense 11×11×11k-mesh for the 2×2×2 supercell of SrFe0.5Ru0.5O3(Figs.1(a)–1(d)),and a 6×6×3k-mesh for the√supercell of Sr2Fe0.5Ru0.5O4(Figs.1(e)–1(k)).In VASP calculations, we consider the following electron configurations as valence electrons: Sr 4s24p65s2, Ru 5s14d7, Fe 4s13d7, and O 2s22p4.Correlation effects in Fe-3d and Ru-4d orbitals are considered by employing DFT+Umethod,[46,47]the interaction parameters for Fe-3d and Ru-4d are intra-orbitalU(Fe)=5.0 eV,Hund’s exchangeJ(Fe)=0.5 eV,inter-orbitalV(Fe)=U-2J=4.0 eV;U(Ru) = 3.0 eV,J(Ru) = 0.3 eV,V(Ru) = 2.4 eV.These parameters are obtained from constrained random phase approximation (cRPA)[48]and agree with previous studies and have been proved effective for reproducing experimental observations,[49,50]e.g., the helical spin order consistent with experiments on SrFeO3.[51]
As an input for the DMFT calculations, a low-energy effective Hamiltonian is generated by projecting the Fe-3d + Ru-4d derived DFT bands computed by WIEN2K around the Fermi level onto Wannier functions[52,53]using WIEN2WANNIER.[54,55]These are supplemented by a local density–density and Kanamori interaction, respectively.We employ the fully localized limit as a double counting correction scheme.[46]We solve the resulting manybody Hamiltonian from 100 K to 300 K within employing a continuous-time quantum Monte Carlo solver in the hybridization expansions[56]using W2DYNAMICS.[57,58]Realfrequency spectra are obtained with the ANA CONT code[59]via analytic continuation using the maximum entropy method(MaxEnt).[60,61]
3.Results and discussion
3.1.Structural properties
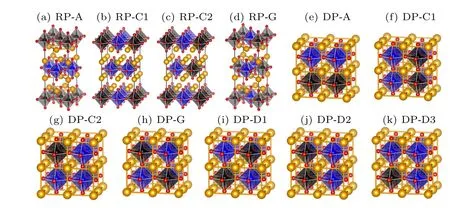
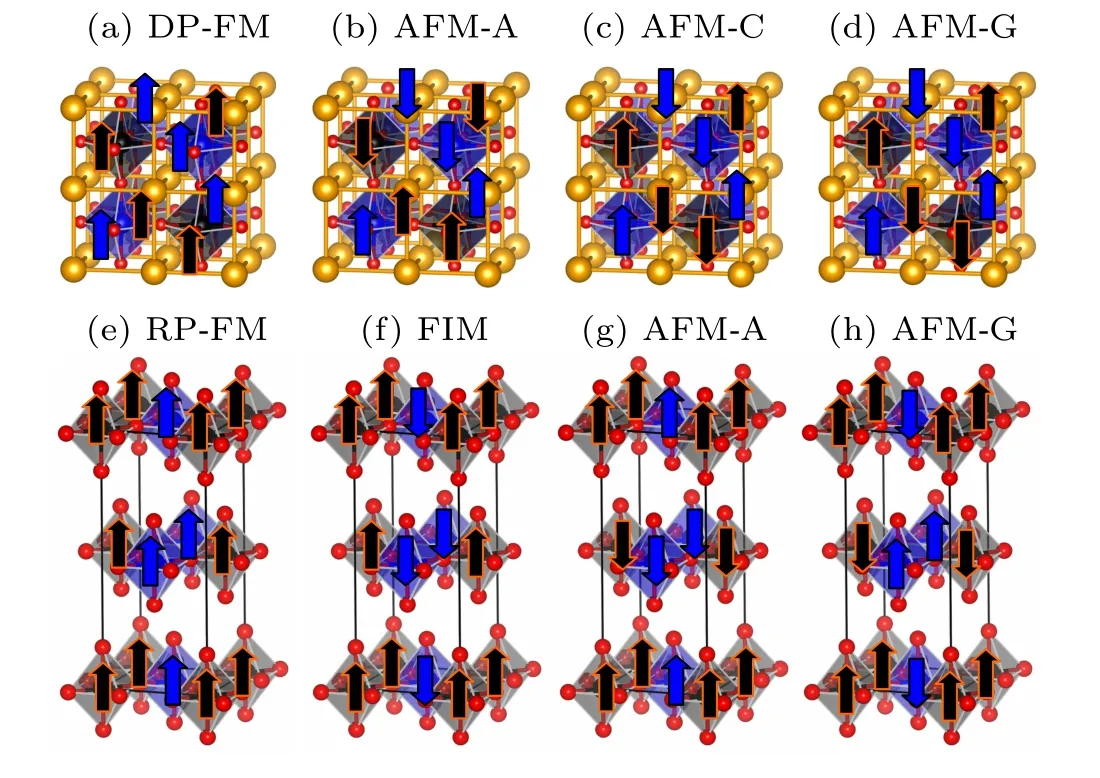
To explore all possible structures for double-perovskite type(DP)SrFe0.5Ru0.5O3and Ruddlesden–Popper type(RP)Sr2Fe0.5Ru0.5O4,we list the possible structures in Figs.1(a)–1(k) and Table 1 provides a summary of the (non-spinpolarized) DFT total energy and the corresponding space group.As shown in Table 1, the G-type structure, where the doped Fe atoms are separated by Ru atoms, exhibits the lowest energy state for both DP SrFe0.5Ru0.5O3(Fig.1(h)) and RP Sr2Fe0.5Ru0.5O4(Fig.1(d)).These results indicate that in SrRuO3and Sr2RuO4,the doped Fe atoms tend to be separated(G-type order)rather than forming atomic clusters or exhibiting antisite disorder.[62]However, achieving a high-quality film of pure G-type double perovskites (DP) SrFe0.5Ru0.5O3or RP Sr2Fe0.5Ru0.5O4may pose challenges due to potential antisite disorder.[62]Previous experiments have indicated that Sr2FeRuO6may exhibit spin-glass behavior[63,64]or longrange room-temperature magnetism[40]when the Ru and Fe ions are randomly distributed or when a perfect double perovskite structure is synthesized.
The relaxed in-plane lattice constants for DP SrFe0.5Ru0.5O3and RP Sr2Fe0.5Ru0.5O4are found to be 7.758 ?A (Fig.1(h)) and 5.384 ?A (Fig.1(d)), respectively.In terms of pseudocubic coordination, these correspond to 3.879 ?A and 3.807 ?A, respectively.These values are comparable to the in-plane lattice constants of SrVO3(3.890 ?A),SrTiO3(3.905 ?A), and LaAlO3(3.778 ?A).The similarity in lattice constants suggests the possibility of epitaxial growth and highlights the potential of these materials for applications in oxide devices.
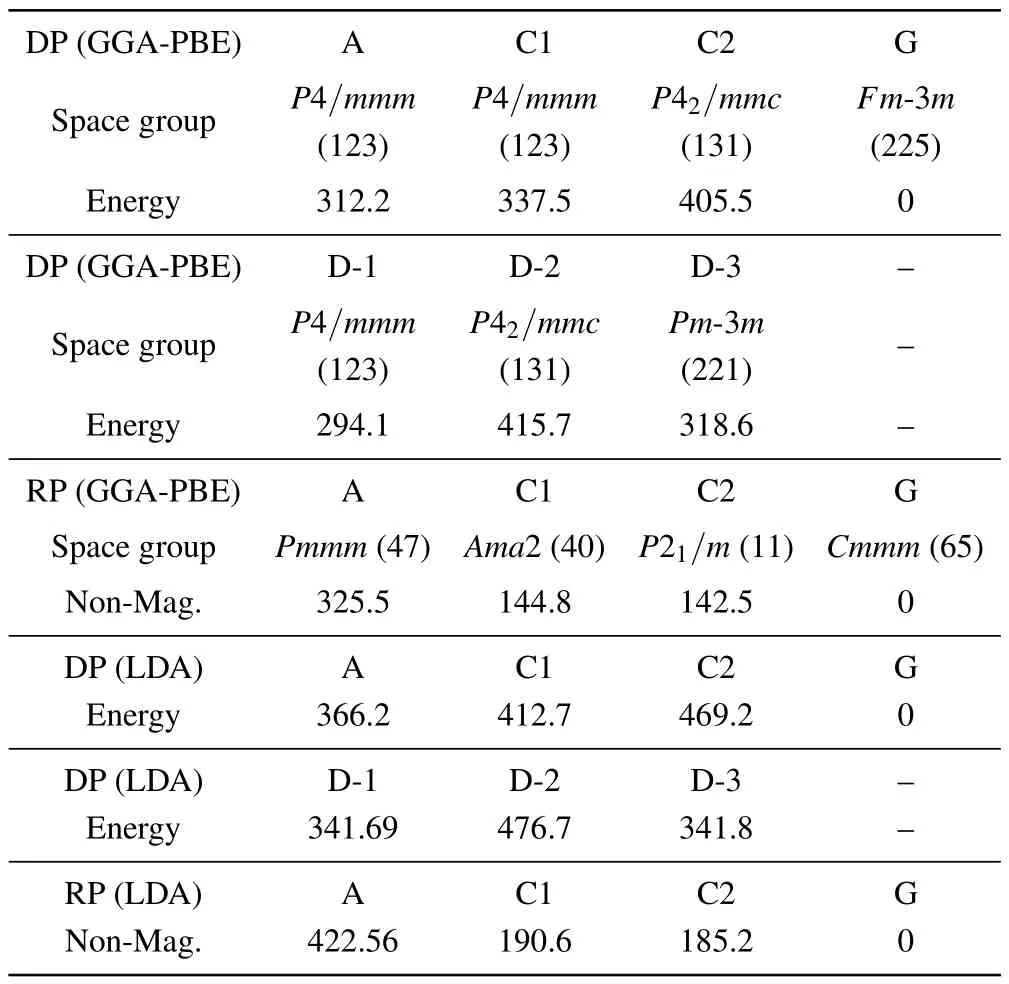
DP(GGA-PBE) A C1 C2 G Space group P4/mmm P4/mmm P42/mmc Fm-3m(123) (123) (131) (225)Energy 312.2 337.5 405.5 0 DP(GGA-PBE) D-1 D-2 D-3 –Space group P4/mmm P42/mmc Pm-3m –(123) (131) (221)Energy 294.1 415.7 318.6 –RP(GGA-PBE) A C1 C2 G Space group Pmmm(47) Ama2(40) P21/m(11) Cmmm(65)Non-Mag.325.5 144.8 142.5 0 DP(LDA) A C1 C2 G Energy 366.2 412.7 469.2 0 DP(LDA) D-1 D-2 D-3 –Energy 341.69 476.7 341.8 –RP(LDA) A C1 C2 G Non-Mag.422.56 190.6 185.2 0
3.2.Electronic properties
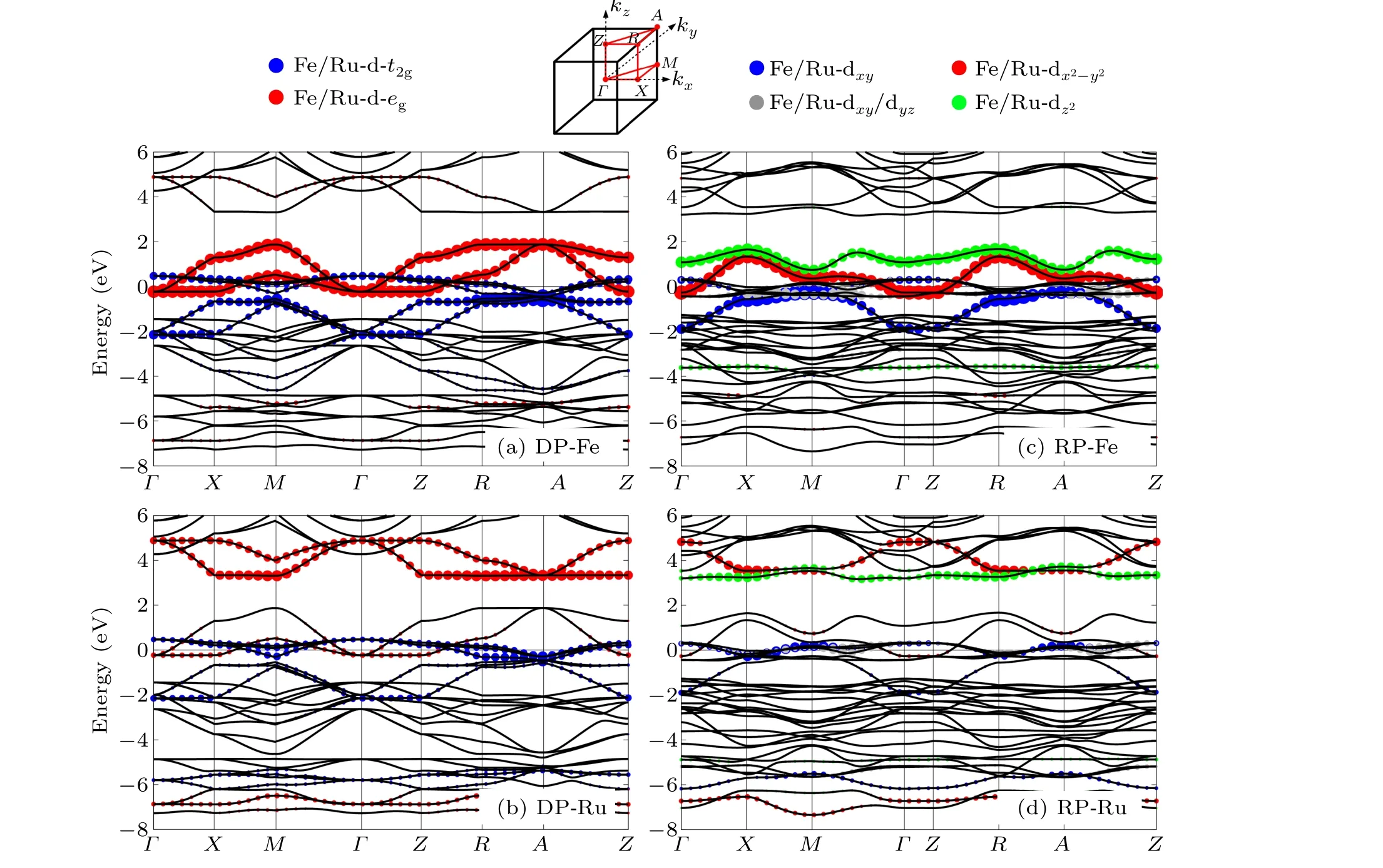
Using the DFT-optimized ground state structures of DP SrFe0.5Ru0.5O3(Fig.1(h)) and RP Sr2Fe0.5Ru0.5O4(Fig.1(d)), we proceed to calculate the (non-spin-polarized)band structure and DOS, as presented in Figs.2 and 3, respectively.In the case of DP SrFe0.5Ru0.5O3, as depicted in Fig.2(a), the Fe-d orbitals exhibit a relatively smaller crystal field splitting between thet2gandegorbitals.In comparison to Ru-4d orbitals, Fe-3d orbitals are more localized and experience less repulsion from the oxygen (O) ligands.The on-site hopping terms of Fe-t2g,Fe-eg,Ru-t2g,and Ru-eg,which are obtained from Wannier projections,are determined as-0.421 eV, 1.661 eV,-0.264 eV, and 3.052 eV, respectively.Consequently,the crystal field splitting(?)betweent2gandegfor Fe and Ru is calculated as 2.082 eV and 3.316 eV,respectively.The smaller?for Fe leads to electronic occupations ategorbitals, this results in the formation of a highspin Fe state under strong Hund’s exchangeJ.In contrast,the larger?for Ru leaves the Ru-t2gstates near the Fermi surface(Ef: 0 eV) while theegstates are located at higher energies ranging from 2.5 eV to 5.0 eV(as shown in the bottom panel of Fig.3(a)).The DOS in Fig.3(a)demonstrates hybridization between Fe-t2gand Ru-t2gorbitals,forming a hybridized peak slightly aboveEf.Additionally, a broader hybridization peak is observed between Fe-egand Ru-egorbitals within the energy range from 0 to 2 eV.The hybridization with Fe-3d effectively reduces the bandwidth of Ru-4d orbitals, as evidenced by the reduced bandwidth (~2.5 eV) of Ru-t2gcompared to~3.2 eV bandwidth in bulk SrRuO3.This reduction in bandwidth plays a crucial role in enhancing electronic correlations and resulting in significant spin-splitting, as discussed in the next section.
Please note that in the non-spin-polarized DOS of the Gtype DP SrFe0.5Ru0.5O3(Fig.3(a)),theegorbitals of both Fe and Ru are mostly unoccupied,and no significant charge transfer is observed.This indicates that both Ru and Fe are close to their nominal d4state.The DOS of DP SrFe0.5Ru0.5O3exhibits similarities with Sr2CrRuO6,[62]with the only difference being that the Cr-d orbitals are occupied by three electrons instead of five in Fe-d orbitals.
In the case of G-type RP Sr2Fe0.5Ru0.5O4,the inter-layer hopping along thez-direction is eliminated due to the layered crystal structure of Ruddlesden–Popper phase.This is evidenced by the DOS of Fe-dz2and Ru-dz2shown in the bottom panel of Fig.3(b).For RP Sr2Fe0.5Ru0.5O4,the bandwidth of dxz/dyzand dz2orbitals for both Fe and Ru is significantly reduced compared to that of DP SrFe0.5Ru0.5O3,resulting from the layered structure of the material.
3.3.Magnetic properties
We proceed to investigate the magnetic properties of DP SrFe0.5Ru0.5O3and RP Sr2Fe0.5Ru0.5O4by calculating the spin-polarized DFT+U(U: Coulomb interaction) total energies for all possible magnetic orders.Figures 4(a)–4(d)and 4(e)–4(h)depict the potential magnetic states of DP SrFe0.5Ru0.5O3and RP Sr2Fe0.5Ru0.5O4, respectively.The DFT+Utotal energies of all magnetic orders are presented in Table 2.In the case of DP SrFe0.5Ru0.5O3,the A-type(AFMA)and C-type(AFM-C)antiferromagnetic orders converge to non-magnetic states during self-consistent DFT+Uiterations,indicating their instability.However, the ferromagnetic (FM)and G-type (AFM-G) states successfully converge.It is important to note that since the magnetic moments on Ru and Fe are not equal,approximately 5μB/Fe and 3μB/Ru,the AFM-G state is essentially a ferrimagnetic(FIM)state with a net magnetic moment of(5-3)/2=1.0μBper SrFe0.5Ru0.5O3.
Table 2 reveals that the FIM state(Fig.4(d))is the ground state with the lowest energy while the FM state (Fig.4(a)) is a metastable state with an energy 151.1 meV higher than the FIM state.By fitting the total energies of the FM and FIM states into a simple 3D Heisenberg model,we estimate that the nearest exchange energy (Jinter-site) between Ru and Fe is approximately 13 meV.This suggests a potentially high magnetic transition (N′eel) temperature in the range of several hundred Kelvin.These findings for DP SrFe0.5Ru0.5O3,specifically the FIM magnetic state, align with experimental results reported in Ref.[40],which reported a room-temperature FIM state in the double perovskite Sr2FeRuO6that is isochemically identical to DP SrFe0.5Ru0.5O3.Additionally,both the FM and FIM states exhibit an insulating behavior with a non-zero band gap.It is expected that an external magnetic field could induce a spin flip from the antiferromagnetically coupled Ru and Fe to ferromagnetically coupled pairs,leading to a reduction in electric resistance.Therefore, DP SrFe0.5Ru0.5O3holds promise for exhibiting the magnetoresistance effect.
In the case of RP Sr2Fe0.5Ru0.5O4, we categorize the possible magnetic orders based on the intra-layer AFM/FM and intra-layer FM/AFM couplings: FM (intra-layer FM+inter-layer FM), FIM (intra-layer AFM+inter-layer FM),AFM-A (intra-layer FM+inter-layer AFM), and AFM-G(intra-layer AFM+inter-layer AFM).By performing spinpolarized DFT+Ucalculations,we find that the ground state is the FIM state (its energy is set as 0) while the metastable state is the AFM-G state with an energy of 2.2 meV.This indicates that the magnetism of RP Sr2Fe0.5Ru0.5O4is primarily governed by a strong intra-layer AFM coupling between Fe and Ru (Jinter-site~7 meV), along with a much weaker inter-layer coupling(Jinter-layer~1 meV).The reduced value ofJinter-site can be attributed to the cutting-off of hopping interactions along the out-of-plane direction (z).In DP SrFe0.5Ru0.5O3, theJinter-site value is approximately 13 meV,which is stronger due to its three-dimensional (3D) nature.In contrast, in RP Sr2Fe0.5Ru0.5O4, the hopping along thezdirection is hindered by the layered structure, resulting in aJinter-site value of roughly half of DP SrFe0.5Ru0.5O3,approximately 7 meV.
The negligible inter-layer coupling withJinter-layer approximately 1 meV corresponds to a temperature of only around 11 K.This suggests that the coexistence of the FIM and AFMG states is possible, where local strain or crystal disorder in realistic samples could locally stabilize one state in certain regions.Additionally, the application of an external magnetic field is expected to facilitate the transformation from the AFMG to the FIM state.Consequently,the resistance can be effectively reduced, similar to DP SrFe0.5Ru0.5O3.Therefore, RP Sr2Fe0.5Ru0.5O4holds promise as a candidate for magnetoresistance effects.
We also perform similar magnetic order calculations including spin–orbital coupling (SOC) in calculations.With SOC, non-collinear magnetic order setups are allowed and they might lead to lower energy compared with the collinear magnetic order as shown in Figs.4(d) and 4(h).We test different combinations by changing the magnetic orientations of Fe- and Ru-moment, e.g., by allowing their magnetic moment along (001), (010), (001), (110), (111) and other possible directions.However,our DFT+U+SOC results demonstrate that collinear G-type orders with both Fe- and Rumoment alongz(001) direction are ground states for both SrFe0.5Ru0.5O3and Sr2Fe0.5Ru0.5O4, as shown in Figs.4(d)and 4(h).
Our findings reveal the following: (i)DP SrFe0.5Ru0.5O3exhibits a robust G-type structure and a semiconducting state with a FIM order.(ii) RP Sr2Fe0.5Ru0.5O4possesses a Gtype structure with a strong intra-layer FIM order and a weak intra-layer coupling of approximately 1 meV.This suggests that the FIM and AFM-G configurations have similar energies,indicating a significant magnetic competition among various magnetic states.This competition may give rise to phenomena such as spin-glass behavior,[66]spin-density waves,[27]or commensurate/incommensurate magnetic ordering.[28]Moreover,this could explain the experimentally observed uncertain magnetism in Fe-doped ruthenates.[27,28,66]
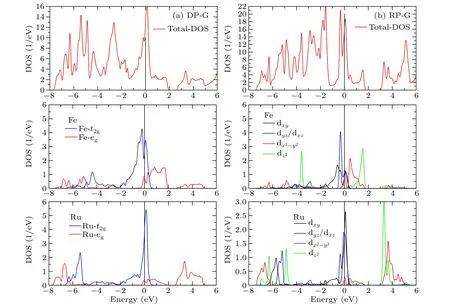
We proceed with the calculation of the spin-polarized DOS for DP SrFe0.5Ru0.5O3and RP Sr2Fe0.5Ru0.5O4.Figures 5(a) and 5(b) illustrate the DOS of DP SrFe0.5Ru0.5O3and RP Sr2Fe0.5Ru0.5O4, respectively.In the case of DP SrFe0.5Ru0.5O3, the Fe dopant atoms are in the Fe3+state with a 3d5electronic configuration, as indicated by the fully occupied spin-majority Fe-t2gandegorbitals (middle panel of Fig.5(a)).The full spin polarization at Fe and the Fe3+valence states are consistent with those observed in Sr2FeMoO6.[67]
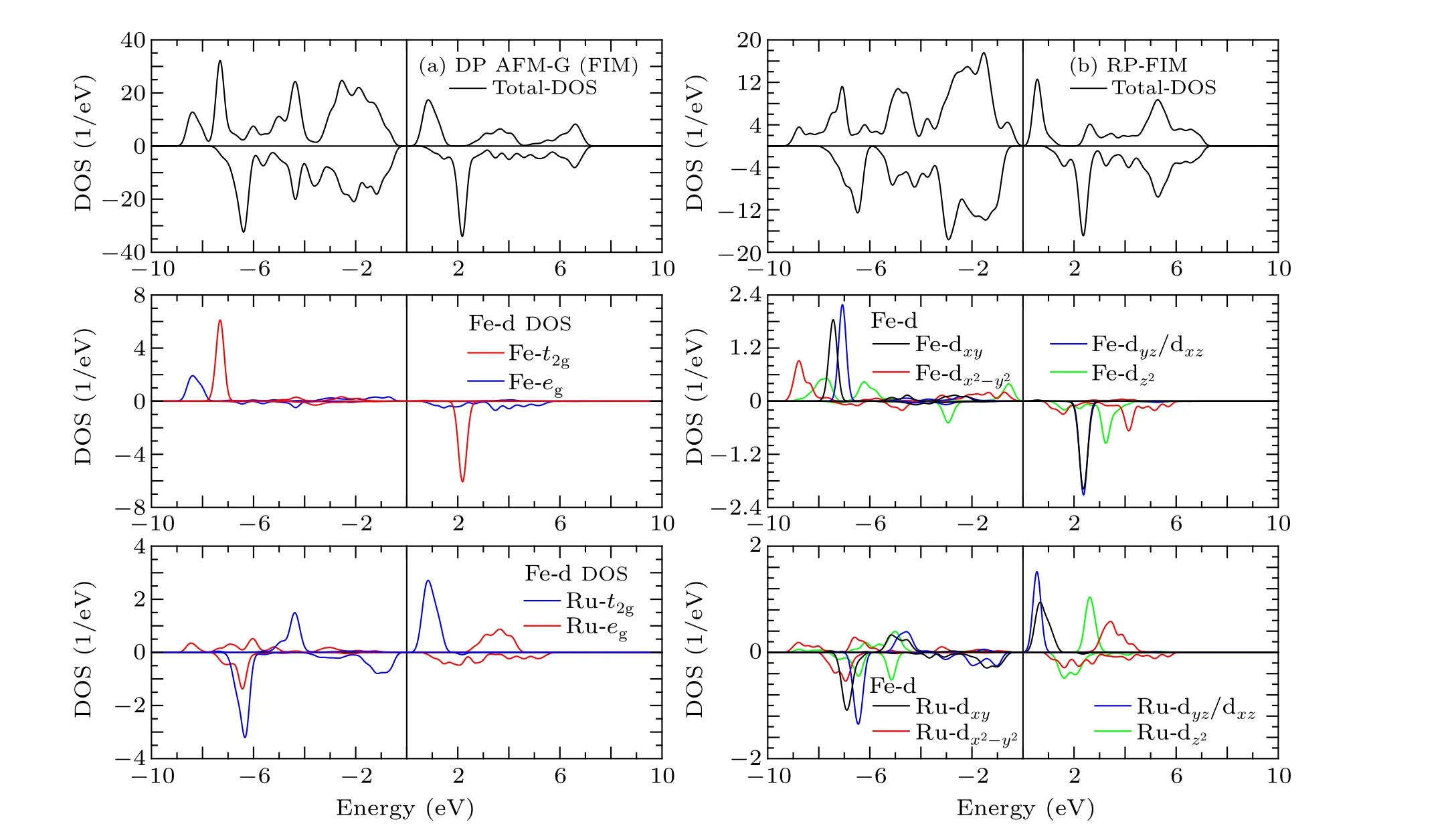
It is worth noting that in the spin-polarized DOS of both compounds (Figs.5(a) and 5(b)), the states near the Fermi level(Ef)are not predominantly composed of hybridized states between Fe and Ru.This suggests a weaker hybridization compared to the non-spin-polarized DOS shown in Figs.3(a)and 3(b).Fe exhibits a strong spin splitting of approximately 8 eV, which is significantly larger than the values of around 2.5 eV for Fe in Sr2FeMnO6,[67]3.5 eV for Cr in Sr2CrRuO6,[62]and 2.0 eV for Cr in Sr2CrMoO6.[68]Additionally, the spin splitting of Ru-t2gin DP SrFe0.5Ru0.5O3is approximately 7 eV,almost three times larger than that in bulk SrRuO3, which is around 2 eV.[48]This indicates that the reduced electronic hoppings and decreased itinerancy make both Fe and Ru more localized compared to their bulk states in SrFeO3and SrRuO3.Consequently, a larger intrinsic magnetic moment develops at the Fe and Ru sites.Due to the weaker hybridization between Fe and Ru in the spin-polarized state,the superexchange interaction between the magnetic Fe and Ru ions is enhanced because it is proportional to the spin moment on Fe and Ru.Along with the half-metallic state exhibited in DP SrFe0.5Ru0.5O3, the superexchange mechanism consequently appears to play an essential role in stabilizing the FIM order.
The DOS of RP Sr2Fe0.5Ru0.5O4exhibits notable similarities to that of DP SrFe0.5Ru0.5O3.For example, the spin-up Fe-t2gstates are located around-7 eV, while the spin-down Fe-t2gstates are at approximately 2 eV.Similarly,the spin-up Ru-dyz/dxzstates are observed around-6 eV, and the spindown states are found at around 1 eV.The layered structure of RP Sr2Fe0.5Ru0.5O4leads to a splitting between the degeneratet2gandegorbitals.These findings suggest that Fedoped ruthenates share similarities with Sr2CrMoO6,[68]such as weakened 3d–4d hybridization and the significant role of superexchange in stabilizing the FIM order.
3.4.Dynamical correlation effects
To investigate the temperature effect, we employ the DMFT method, as DFT is a zero-temperature theory.We consider two different Hamiltonians, Kanamori and density–density interactions, at temperatures of 200 K and 300 K.The DMFT calculations confirm the presence of FIM order in DP SrFe0.5Ru0.5O3and RP Sr2Fe0.5Ru0.5O4, respectively,consistent with the results obtained from DFT+Ucalculations.Figure 6 displays the Kanamori DMFT spectra of DP SrFe0.5Ru0.5O3and RP Sr2Fe0.5Ru0.5O4at room temperature(T=300 K).In DP SrFe0.5Ru0.5O3,the Fe and Ru atoms are antiferromagnetically coupled, with the spin-majority channel being spin-up for Fe and spin-down for Ru.The spinupt2gandegorbitals of Fe are fully occupied while the spin-downt2gandegorbitals are only slightly occupied, indicating a high-spin Fe3+(3d5) state.As for Ru, the spindownt2gorbitals are occupied while the spin-downegorbital and other states in the spin-up channel are all empty,indicating a high-spin Ru5+(4d3) state.The magnetic moment per SrFe0.5Ru0.5O3in the DP phase is approximately 1μBat 300 K.In RP Sr2Fe0.5Ru0.5O4, the DMFT spectra exhibit remarkable similarities to those of DP SrFe0.5Ru0.5O3,with the only difference being the degeneracy splitting within thet2gandegorbitals.Specifically, the dxy(dx2-y2)orbital is not symmetric to the dyzand dz2orbitals as observed in DP SrFe0.5Ru0.5O3.These DMFT results indicate that the magnetism in both systems is expected to persist even at room temperature.To make summary, the magnetic exchange parameters from DFT+U,and N′eel that is estimated from magnetic exchange parametersJand computed from DMFT as shown in Table 3,one can see that the values from DMFT are definitely higher than the estimated values fromJ, indicating possible important roles of correlation effects in Fe-doped ruthenates.Additionally,we also compute the net magnetic moments using the density–density interactions,and it is found that the net moments of both systems increased by 10%, highlighting the significance of Hund’s exchange interaction(J).

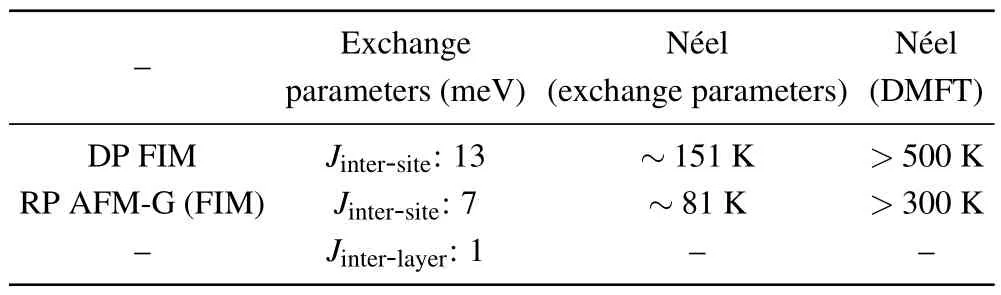
–Exchange N′eel N′eel parameters(meV) (exchange parameters) (DMFT)DP FIM Jinter-site: 13 ~151 K >500 K RP AFM-G(FIM) Jinter-site: 7 ~81 K >300 K–Jinter-layer: 1––
Note that the ruthenates we have studied in this paper are all at exactly 50%Fe-doping,however,crystal and lattice defects, as well as inter-atomic mixing, and even the formation of oxygen vacancies, are usually unavoidable in practical experiments.For oxides, the above types of crystal defects, as well as oxygen vacancies, are expected to induce doping of electrons or holes.Any additional electron or hole doping will close to the Mott band gap,leading to the formation of metallic phases.Moreover, this will, to some extent, increase the double-exchange interaction of the system,and we expect that a moderate amount of hole or electron doping will enhance the double-exchange interaction while decreasing the strong electronic correlation effects at Ru and Fe as well as the superexchange interaction, which will likely lead to the transition of both the Fe-doped ruthenates from FIM to FM state.[69–71]
4.Conclusion
Motivated by the potential for exotic physical properties induced by Fe-doping in ruthenates, we conducted a comprehensive study on the structural, electronic, and magnetic properties of Fe-doped double perovskite SrRuO3and Ruddlesden–Popper Sr2RuO4, specifically SrFe0.5Ru0.5O3and Sr2Fe0.5Ru0.5O4.TMOs with 3d electrons often exhibited remarkable magnetic properties around room temperature,and thus, we anticipated that Fe-doping would enhance the magnetic performance of SrRuO3and Sr2RuO4by raising the Curie or N′eel temperatures.
Our investigations reveal that the Fe atoms tend to segregate,leading to the formation of G-type structure with Fe and Ru atoms in both SrFe0.5Ru0.5O3and Sr2Fe0.5Ru0.5O4.These compounds adopt a double-perovskite-type three-dimensional(3D) and two-dimensional (2D) structure, respectively.The interplay between weakened 3d–4d hybridization, enlarged spin-splitting, and enhanced correlation effects results in a substantial charge transfer from Ru to Fe,resulting in Fe3+and Ru5+states.The superexchange interaction between Fe3+and Ru5+ions gives rise to a robust FIM semiconducting ground state in both systems.By considering dynamical correlation effects,band gaps of up to 2 eV are observed.
Due to their FIM ground states, significant spin-flip behavior is expected under the influence of a strong external magnetic field, implying the potential for remarkable magnetoresistance effects.To investigate the survival of the FIM states in SrFe0.5Ru0.5O3and Sr2Fe0.5Ru0.5O4at higher temperatures,we perform temperature-dependent DMFT calculations.The results demonstrate that the FIM states persist even at elevated temperatures,including room temperature(300 K).The ability to stabilize semiconducting FIM states through Fe doping in SrRuO3and Sr2RuO4renders Fe doping a promising strategy for overcoming the limitations of working and operating temperatures in 4d transition metal oxides such as ruthenates.
Acknowledgments
Project supported by the starting funds from Northwest University.We thank Paul Worm and Karsten Held for fruitful discussion and support of computational resources.Calculations have been mainly done on the Vienna Scientific Clusters(VSC)and supercomputer at the School of Physics of Northwest University.
- Chinese Physics B的其它文章
- The application of quantum coherence as a resource
- Special breathing structures induced by bright solitons collision in a binary dipolar Bose–Einstein condensates
- Effect of short-term plasticity on working memory
- Directional-to-random transition of cell cluster migration
- Effect of mono-/divalent metal ions on the conductivity characteristics of DNA solutions transferring through a microfluidic channel
- Off-diagonal approach to the exact solution of quantum integrable systems

