HUANG Ziwei,CHENG Xiang,and ZHANG Nan

(1.State Key Laboratory of Advanced Optical Communication Systems and Networks,School of Electronics Engineering and Computer Science,Peking University,Beijing 100871,China;2.Key Laboratory of Wireless Sensor Network&Communication,Shanghai Institute of Microsystem and Information Technology,Chinese Academy of Sciences,Shanghai 200050,China;3.Wireless Algorithm Department,Product Research and Development System,ZTE Corporation,Shanghai 201203,China)

Abstract:A novel non-geometrical stochastic model (NGSM) for non-wide sense station?ary uncorrelated scattering (non-WSSUS) vehicle-to-vehicle (V2V) channels is proposed.This model is based on a conventional NGSM and employs a more accurate method to re?produce the realistic characteristics of V2V channels,which successfully extends the ex?isting NGSM to include the line-of-sight(LoS)component.Moreover,the statistical proper?ties of the proposed model in different scenarios,including Doppler power spectral density(PSD),power delay profile (PDP),and the tap correlation coefficient matrix are simulated and compared with those of the existing NGSM.Furthermore,the simulation results dem?onstrate not only the utility of the proposed model,but also the correctness of our theoreti?cal derivations.

Keywords:vehicle-to-vehicle; non-WSSUS channels; non-geometrical stochastic model;LoS component;statistical properties

1 Introduction

Vehicle-to-vehicle (V2V) communication plays a key role in intelligent transportation systems (ITS),which aims at improving the traffic efficiency,minimizing traffic accidents and enabling some new applications[1].As a newly emerging communication technique,V2V com?munication faces research challenges and standardization prob?lems,which limit its further development.Therefore,much re?search attention has been attracted to V2V channel modeling for facilitating the analysis and design of V2V communication systems[2],[3].

As addressed in[4],[5],V2V channel models can be catego?rized as geometry-based deterministic model (GBDM) [6] and stochastic model,and the latter can be further classified as non-geometrical stochastic model (NGSM) [7],[8] and geome?try-based stochastic model (GBSM).The GBDM is character?ized by V2V physical channel parameters in a completely de?terministic approach,whereas its computational complexities increase with the accuracy requirement.Compared with GB?DM,stochastic models have better tradeoff between accuracy and complexity,and thus have been widely used currently in V2V channel modeling.The authors in[9]–[15]proposed sev?eral GBSMs,which used the simplified ray tracing principle and equivalent scatterer concept to simulate propagation envi?ronment.Though the GBSM can be easily adapted to diverse scenarios,it is more complex than the NGSM.The NGSM de?termines physical parameters of the V2V channel in a com?pletely stochastic manner without presuming any underlying geometry.In the literature [7],[8],two conventional wideband NGSMs were proposed and are both based on the tapped delay line(TDL)structure.

The wideband NGSM developed in [7] is a conventional channel model standardized by IEEE 802.11p.The NGSM in[7] includes the line-of-sight (LoS) component and has variable types of Doppler spectra for different delays.Specifically,due to the short distance of V2V communication,it often includes the LoS component,especially for the scenario with low vehicu?lar traffic density (VTD).In order to identify the presence of LoS component,the model employs the Ricean fading.More?over,in the NGSM [7],each tap contains several unresolvable subpaths,and subpaths with different delays have different types of Doppler spectra,e.g.,flat shape,round shape,classic 3 dB shape,and classic 6 dB shape.However,for simplicity,the NGSM[7]is based on the wide sense stationary uncorrelated scattering (WSSUS) assumption and only employs the Ricean fading,which cannot mimic the severe fading in V2V channels.

In the wideband NGSM [8],two characteristics of the V2V channels not considered in[4]are taken into account,i.e.,nonstationarity and severe fading.Due to unpredictable traffic and changes in the size,location,as well as velocity of scatterers,the number of multipath components and strengths alter frequently,resulting in the non-stationarity in both time and frequency do?mains for V2V channels.The NGSM [8] employs the first-order two-state Markov chains and generates correlated stochastic vari?ables to describe the non-stationarity in both time and frequency domains,respectively.Moreover,in V2V channels,the fading of multipath component is often worse than Rayleigh fading due to severer delay dispersion and Doppler dispersion.Hence,the model also describes the severe fading by means of the Weibull distribution,in which the tap amplitudes follow the Weibull dis?tribution,with the fading parameter determining fading severity.However,since the NGSM [8] considers both non-stationarity and severe fading,it is difficult to further identify the existence of LoS component and possess variable types of Doppler spectra for different delays.

Based on the measurement data presented in above two con?ventional NGSMs,we can summarize four important and unique characteristics that exist in V2V channels,i.e.,nonstationarity,severe fading,the existence of LoS component,and variable types of Doppler spectra for different delays.However,both NGSMs implement only half of the above four characteristics.Therefore,neither of the two conventional NGSMs can meet the requirements.Motivated by this,a new NGSM is desirable to be developed for non-WSSUS V2V chan?nels.

To fill the aforementioned gap and accurately describe the characteristics of the V2V channel,we propose an improved model based on the NGSM [8] for non-WSSUS V2V channels.The proposed model not only considers the non-stationarity but also describes the severe fading in V2V channels,keeping the advantages of the NGSM [8].Moreover,to mimic the V2V channel non-stationarity in frequency domain,channel models should generate correlated taps,which consist of the amplitude part and phase part.Since the amplitude and phase of taps are independent,the distribution of amplitude and phase should be properly modeled respectively.Specifically,the tap ampli?tude statistics can be modeled as Weibull distribution to mimic the severe fading.For simplicity,most papers defined a uni?formly distributed phase over the interval as shown in [8] and[16].However,the uniformly distributed phase leads to the corresponding channel taps having no ability to include the LoS component.To include the LoS component,the authors in[17] proposed a Laplace distributed phase.However,the La?place distributed phase cannot mimic the scenarios without the LoS component.To further fill the gap,we propose a non-uni?formly distributed phase,which is properly combined with the fading parameter used in the severe fading modeling.By changing value of the fading parameter,the proposed model can flexibly mimic different V2V scenarios with and without LoS components.The main contributions of this paper can be summarized as follows.

1) For the first time,we prove that it is inappropriate to im?pose a uniformly distributed tap phase,which causes the ab?sence of LoS component.

2) We propose an improved NGSM with the non-uniformly distributed phase for non-WSSUS V2V channels,which ex?tends the NGSM [8] to identify the presence of the LoS compo?nent.Therefore,the proposed model has the ability to mimic the aforementioned first three unique characteristics of V2V channels.The simulation results demonstrate that the LoS component is successfully added into Doppler power spectral density(PSD).

3) The effectiveness and accuracy of the proposed V2V channel model are validated by extensive simulations.

The rest of this paper has the following structure.In Section 2,we describe the constriction steps of the NGSM in [8] and propose an improved NGSM.The simulation results of the pro?posed model for different scenarios,including Doppler PSDs,power delay profile (PDP),and the tap correlation coefficient matrix are provided and analyzed in Section 3 in comparison with the NGSM [8].Finally,conclusions are drawn in Sec?tion 4.

2 Channel Models for Non-WSSUS V2V Channels

In this section,we first review the constriction steps of the NGSM in [8] and prove that imposing a uniformly distributed tap phase is inappropriate.Then,an improved NGSM with non-uniformly distributed tap phase is proposed.

2.1 Conventional NGSM with Uniform Phase Distribution

The constriction steps of existing NGSM [8] include three parts:non-WSS modeling,non-US modeling,and severe fading modeling.In this section,we will briefly describe the constric?tion steps of the model and prove that it is too restrictive to im?pose a uniform phase distribution in the NGSM[8].

2.1.1 Non-WSS Modeling

Due to unpredictable traffic and changes in the size,loca?tion,and velocity of scatterers,the number of multipath com?ponents and strengths alter frequently.Based on the litera?ture [18],the NGSM in [8] represents the non-WSS charac?teristic by employing the“birth and death”process with per?sistence processZk(t)={0,1}in V2V channel model,where tap“off”means below 25-dB threshold from the main tap.Such thresholding methods [19]–[21] are widely used in the literature to limit the number of taps to those that have the non-negligible energy[8].

In addition,the state transition process of the on/off process can be described by first-order two-state Markov chains,and the transition (TS) matrix and the steady-state (SS) matrix [8]can be given by

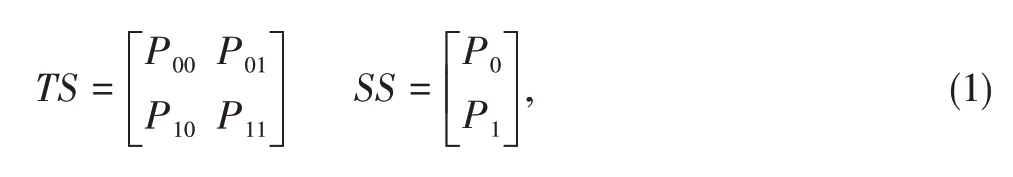
where each elementPijin matrixTSis defined as the probabili?ty of going from stateito statej,and eachSSelementPjgives the“steady-state probability”associated with thejth state.

Then,the channel impluse repulse (CIR) of the NGSM in [8]can be expressed as

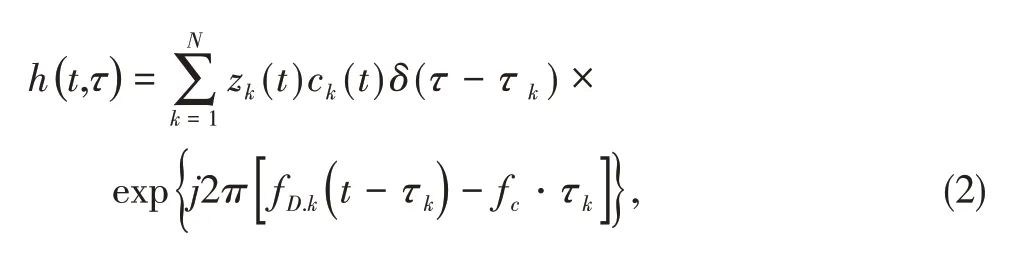
whereZk(t)={0,1}is a persistence process used to account for the finite“l(fā)ifetime”of the propagation paths.h(t,τ)denotes the channel output at timetdue to an impulse input at timet-τ.ck(t) represents thek-th received amplitude,the exponential term represents thek-th received phase,and thek-th echo path has a time-varying propagation delayτk.Theδfunction is a Di?rac delta (impulse),andfcis the carrier frequency in Hz.The termfD,Krepresents the Doppler shift,which is associated with thek-th received multipath echo.

2.1.2 Non-US Modeling

Non-US characteristic reflects the impacts of correlation on different paths/taps,which represents the delay domain charac?teristics.To accurately represent correlated scattering (non-US) characteristic,the model generates complex Gaussian sto?chastic variables.As shown in Fig.1,the correlated complex Gaussian stochastic variables can be generated with any de?sired correlation coefficients.

The process to generate correlated complex Gaussian sto?chastic variables with correlationρGfrom pairs of uncorrelated Gaussian stochastic variables is as follows:1) Generate uncor?related complex Gaussian stochastic variablesVthrough the in?dependent Gaussian stochastic variables;2)using Cholesky de?composition of the correlation matrixLLH=ρGto determine the coloring matrixV,whereLHis the Hermitian transpose ofL; 3)Generate correlated complex Gaussian stochastic variables by means ofW=LV.

2.1.3 Severe Fading Modeling

In V2V channels,due to more severe delay dispersion,Dop?pler dispersion,and non-stationarity characteristics,the fading of multipath component is often worse than Rayleigh fading.As addressed in[22],overall,the best fit for the largest number of taps is obtained by means of the Weibull distribution.Therefore,in the NGSM [8],the tap amplitude statistics are modeled as the flexible Weibull distribution,which can be written as

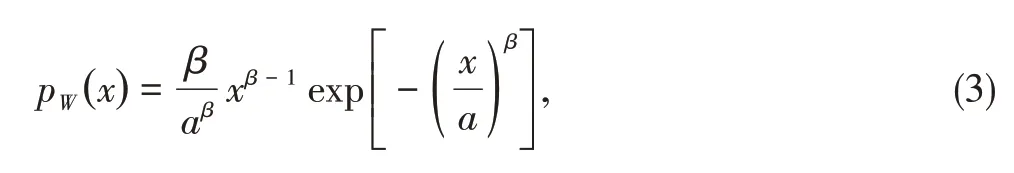
whereα=denotes a scale parameter andβis a fading parameter to represent fading severity.Whenβ=2,the Weibull distribution can be transformed to the wellknown Rayleigh distribution,and asβincreases,the situation in which the signal becomes more deterministic.Whenβis large enough,it means that the LoS component will exist in the V2V communications.However,whenβ< 2,the severe fad?ing will exist in the V2V channel.

As can be seen from the above analysis,severe fading model?ing can be implemented by the Weibull stochastic process,whereas Weibull stochastic process can be obtained by a com?plex Gaussian stochastic process [23].Specifically,the detail construction steps of the NGSM in [8] is shown in Fig.2.As can be readily observed from the figure,the time correlated do?main of the model is implemented by means of the linear con?volution for the Gaussian stochastic process.In order to main?tain the type of Doppler spectra,the NGSM in [8] employs the separation of amplitude and phase,in which only the ampli?tude is transformed with complex exponentiation 2/β.Where?as,the phase is not be transformed,which is directly separated from the complex Gaussian stochastic variables.

The phase is gained directly from the complex Gaussian sto?chastic process and if we do not consider the time correlation,the phase will follow a uniform distribution over [ -π,π].Consequently,the tap amplitude follows the Weibull distribu?tion and the tap phase follows the uniform distribution.Thus,the stochastic variables can be expressed as

▲Figure 1.Generating correlated complex Gaussian stochastic vari?ables.

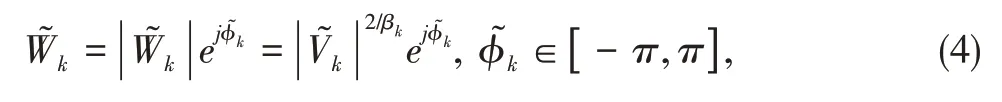
where the number of taps is assumed to beKandis the tap phase of the NGSM[8].

Due to the phasewith a uniformly distributed over[ -π,π],the mean of the NGSM[8]can be calculated as

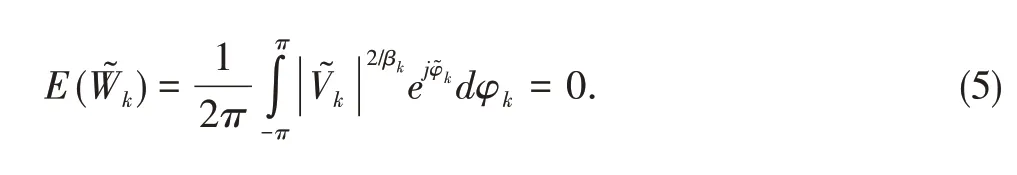
The uniformly distributed tap phase denotes zero-mean inphase and quadrature components,which causes the absence of LoS component in NGSM [8].However,based on the afore?mentioned analysis,the existence of LoS component is one of the important and unique characteristics of V2V channels.Consequently,we can conclude that it is inappropriate to im?pose a uniformly distributed tap phase.To fill up the afore?mentioned gaps and accurately describe the characteristics of the V2V channel,an improved model with a non-uniform phase distribution is described thereafter.

2.2 Improved NGSM with Non-Uniform Phase Distribution

In this section,an improved NGSM with non-uniformly dis?tributed tap phase is proposed,which is based on the existing NGSM [8].The process to develop the improved model also consists of three parts:non-WSS modeling,non-US modeling,and severe fading modeling.However,the proposed model em?ploys a more accurate method to represent the characteristics of V2V channels,which extends the NGSM[8]to have the abil?ity to include the LoS component.

As can be seen from the above analysis,LoS component can?not be included in the NGSM [8].This is because the tap phase is directly gained from the separation from Gaussian sto?chastic process and follows a uniform distribution in the inter?val[ -π,π],which causes the absence of the LoS component.Thus,the uniformly distributed tap phase must be changed.Specifically,in the Weibull stochastic process,the amplitude and the phase of complex Gaussian stochastic variables are both transformed with complex exponentiation 2/β,and then the complex Gaussian stochastic variables are separated into the amplitude part and the phase part since the amplitude and phase of the complex stochastic variables are independent on each other.As a result of the above transformation,βaffects equivalently the amplitude part and the phase part.Conse?quently,the tap amplitude follows the Weibull distribution and the tap phase follows non-uniform distribution.Above all,the constriction steps of the improved mode are shown in Fig.3.

Withβbeing increased,the resulting tap phase concentrates within a smaller phase range,as expected.Consequently,an impulse at zero occurs asβ→∞.Also,whenβ=2,a uni?formly distributed phase occurs,and the stochastic variables of the improved model can be expressed as

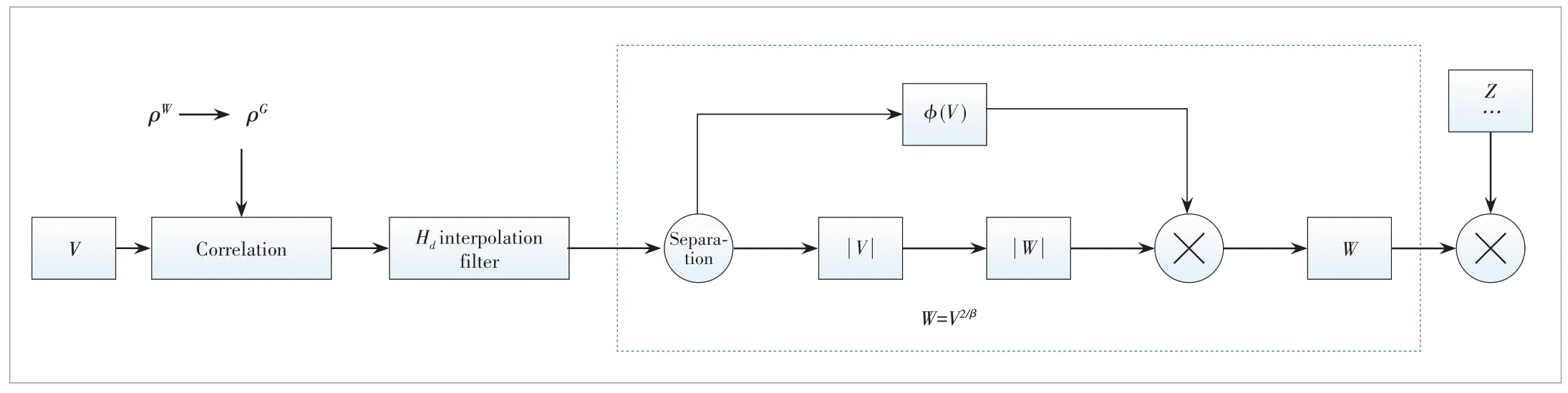
▲Figure 2.The constriction steps of the NGSM [8] (V is an independent and identical complex Gaussian stochastic variable,and Z is a post-operation such as a persistence process).

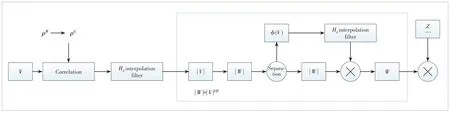
▲Figure 3.The constriction steps of the improved model (V is an independent and identical complex Gaussian stochastic variable,and Z is a post-oper?ation such as a persistence process).

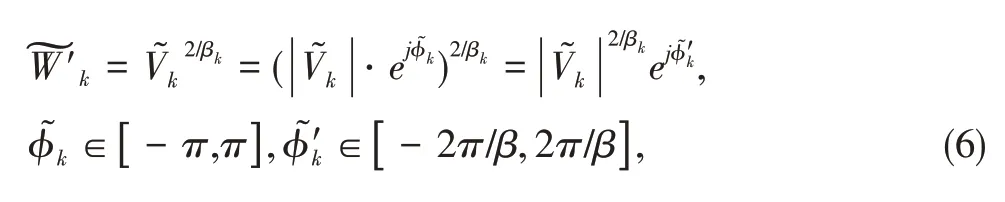
where the number of taps is assumed to beKandis the tap amplitude,which follows the Weibull distribution.is the tap phase of the improved model and follows the non-uniform distribution,which is a linear function of the uniformly distrib?uted phase.Specifically,the tap phase of the improved model can be given by

Similarly,the mean of the improved model can be calculated as

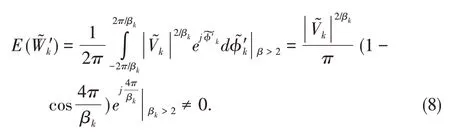
Equation (8) shows that asβincreases,the tap phase concen?trates within a smaller range,resulting in≠0.Further?more,on comparing (5) and (8),we can conclude that the im?proved model with non-uniformly tap phase no longer denotes zero-mean in-phase and quadrature components,the Doppler PSD will show a dominant frequency component,which is able to describe the existence of LoS component [24].Thus,the re?sults demonstrate the advantage of our proposed method.

Further analysis shows that each tap of V2V channels exhib?its serious Doppler spread due to the high mobility of vehicles,and the phase distribution ranged from[ -π,π]is not enough to represent the serious Doppler environment adequately.The non-uniformly distributed tap phase that derived from the con?ventional definition of the fading parameterβis more accurate?ly used to describe the characteristics of V2V channels.Equa?tion(8)allows further observations:theandβis positive?ly correlated,which means that withβbeing increased,the sig?nal becomes more deterministic and the LoS component is also larger[24].

It is also worth noting that the transformation of phase does not influence the amplitude of complex Gaussian stochastic vari?ables,so that this improvement does not have impact on the type of Doppler spectra and the tap correlation coefficient matrix.

3 Simulation Results and Analysis

In this section,the statistical properties of the proposed mod?el in different scenarios,including Doppler PSD,PDP,and the tap correlation coefficient matrix are simulated and compared with those of the NGSM in [8].To better compare the perfor?mance of the two models,the following key parameters are uti?lized to obtain the simulation results,which are the same as those in [8].Specifically,the key parameters can be set as the carrier frequencyfc=5.12 GHz,the bandwidthBW=10 MHz,and the duration of each“birth and death”process state (i.e.,the coherence time of the channel)Tc=0.0005 ～0.001s.Moreover,all the simulation scenarios are also the same as those in [8],including Small City (S),Urban-Antenna Outside Car(UOC),Urban-Antenna Inside Car (UIC),Open-Area High Traf?fic Density(OHT),and Open-Area Low Traffic Density(OLT).

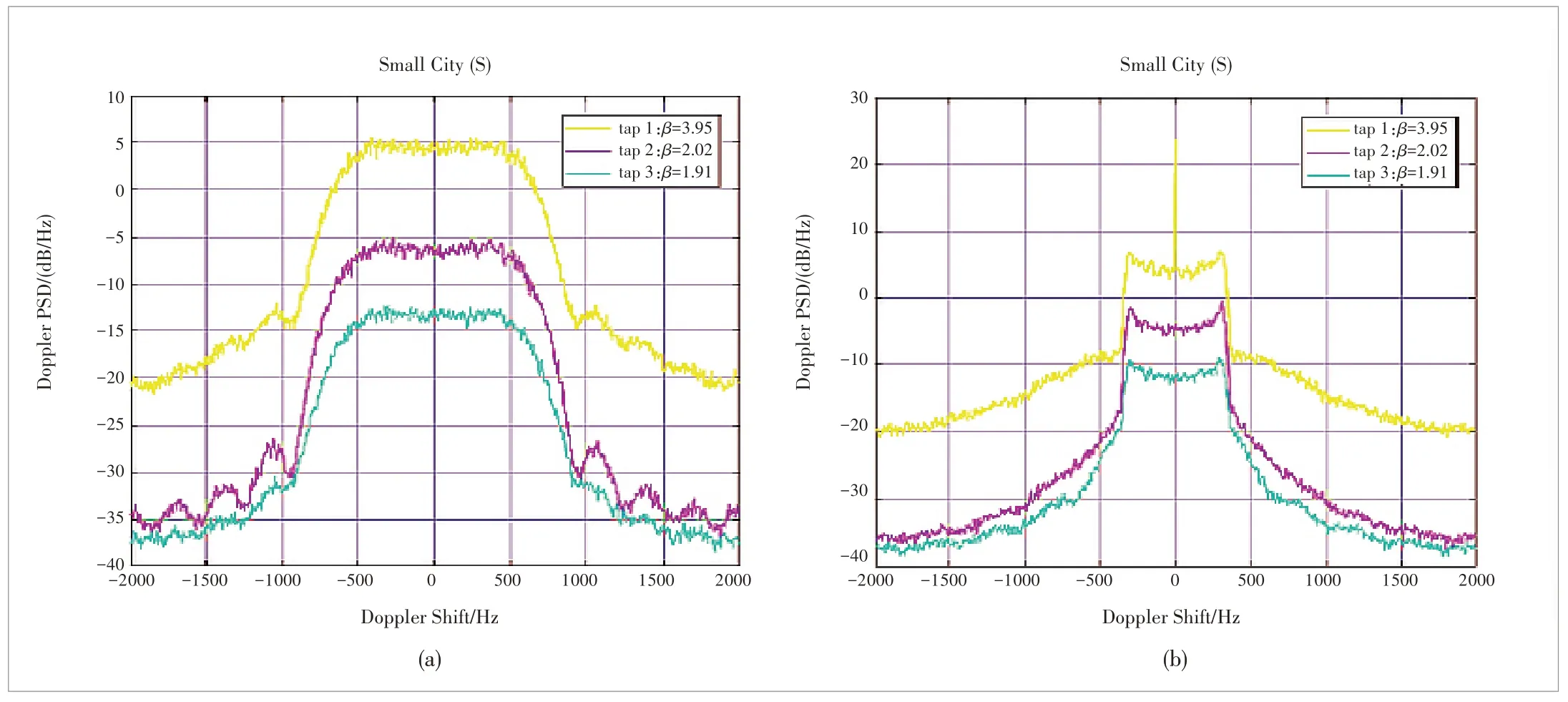
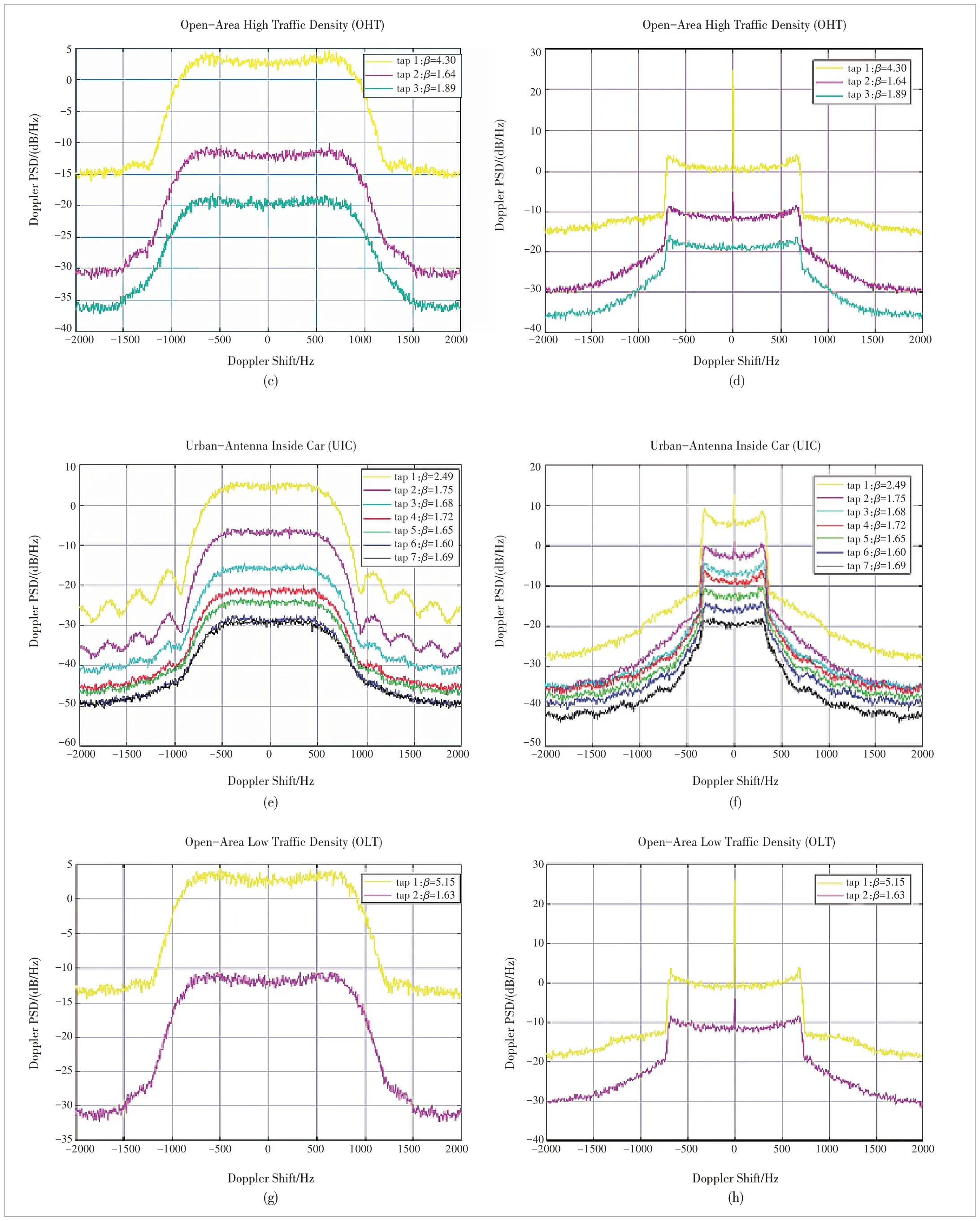
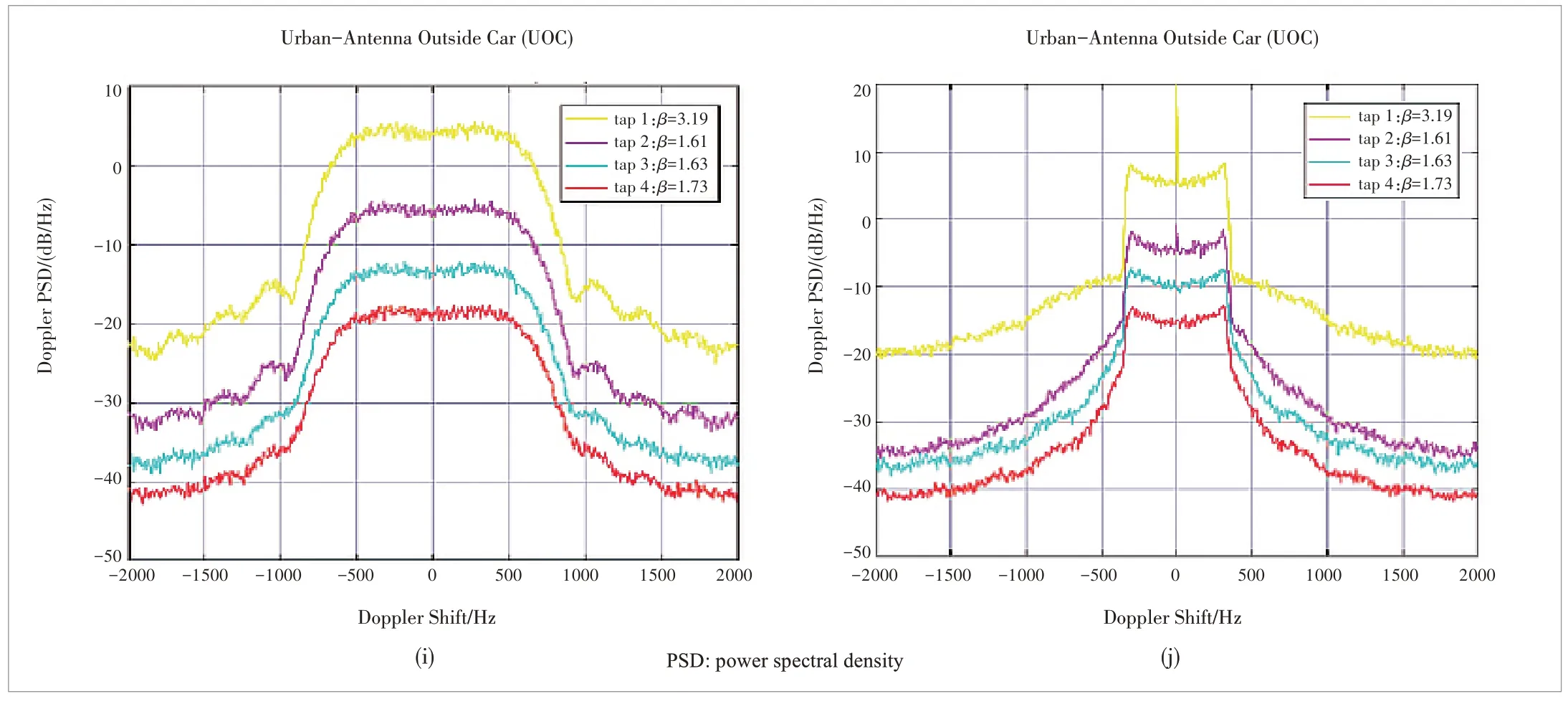
▲Figure 4.The Doppler PSD of different models for different scenarios.(a)Doppler PSD of the model in [8]for S scenario;(b)Doppler PSD of the im?proved model for S scenario;(c)Doppler PSD of the model in[8]for OHT scenario;(d)Doppler PSD of the improved model for OHT scenario;(e)Dop?pler PSD of the model in [8] for UIC scenario; (f) Doppler PSD of the improved model for UIC scenario; (g) Doppler PSD of the model in [8] for OLT scenario; (h) Doppler PSD of the improved model for OLT scenario; (i) Doppler PSD of the model in [8] for UOC scenario; (j) Doppler PSD of the im?proved model for UOC scenario.

Fig.4 provides the comparison between the Doppler PSD of the model in [8] and the improved model.Due to the similar Doppler PSD for each scenario,for simplicity,we select scenario S to analyze the simulation results.As can be readily observed from Fig.4a,for scenario S,the maximum Doppler shiftfD,maxis about 500 Hz and the type of Doppler PSD is“classic 3dB”.Specially,we notice that Doppler PSD of the NGSM [8] shows no dominant LoS component.In Fig.4b,we show the simula?tion results of the improved model for scenario S.It is obvious that maximum Doppler shiftfD,maxis still 500 Hz and the Dop?pler PSD is also“classic 3 dB”.As expected,Doppler PSD of the improved model has a strong narrow peak in the mid?dle,which is characteristic to communications in presence of LoS component [25]–[27].Moreover,the energy of taps with fading parameterβ< 2 is obviously lower than that of other taps,which is consistent with the model in [8].This is be?cause in the improved model,we also employβ< 2 to de?scribe the severe fading in V2V channels.The excellent agreement between the theoretical and measured Doppler PSD confirms the utility of the improved model.Therefore,the simulation results demonstrate that the improved model also has the ability to mimic the severe fading,keeping the advantages of the model in[8].

To further validate the utility of the proposed model,we com?pare our model with measurement data and model in [7] as shown in Fig.5.It is clear that Doppler PSD of the model in[7] has a dominant narrow peak in the middle to identify the presence of LoS component,which is consistent with that of the improved model.Therefore,we can conclude that on the basis of maintaining the merits of the model in [8],the improved model can mimic the existence of LoS component and better mimic the characteristics of V2V channels.

In Table 1,we show the tap correlation coefficient matrix of the NGSM in [8] and the improved model for scenario UIC,re?spectively.The tap correlation coefficient matrix is defined asρ=[ri,j]=where[ri,j] is the coefficient be?tween tapiandj,var(?) denotes variance and cov(?) de?notes covariance.Since the correlation coefficient matrix is symmetric about the diagonal,we only need to specify the up?per or lower triangular part; for brevity,the lower triangular part corresponds to correlations between taps for the improved model,whereas the upper triangular part corresponds to corre?lations between taps for the NGSM in [8].Through comparison between the corresponding parameters under scenario UIC,we can observe that the corresponding parameters of the improved model only fluctuate with a few correlation coefficient values,but most of them remain consistent.This is because the change of phase distribution does not influence the amplitude of complex Gaussian stochastic process,so this improvement does not have impact on the tap correlation coefficient matrix.The excellent agreement between the simulation results and measured data confirms the utility of the improved model.Therefore,the improved model also has the ability to imple?ment the non-stationarity in frequency domain,maintaining the advantages of the NGSM[8].

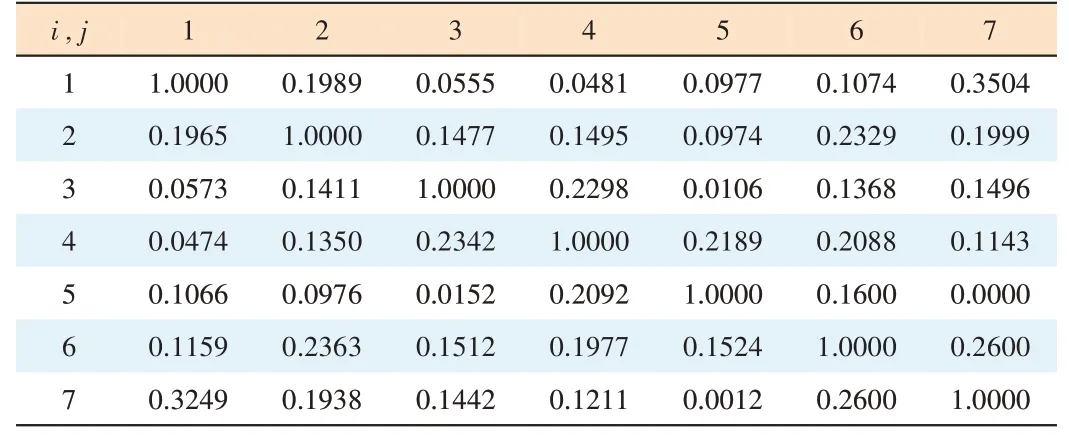
▼Table 1.Correlation matrices of the non-geometrical stochastic model(NGSM)in[8]and improved model for scenario UIC(lower/upper trian?gular part:improved model/NGSM in[8])

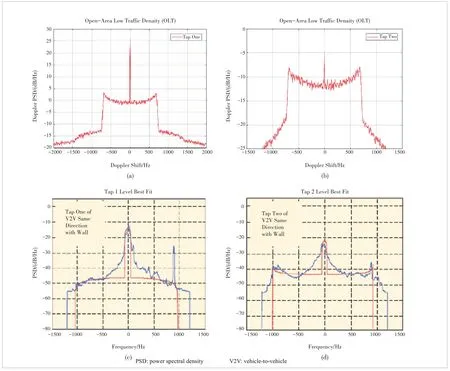
▲Figure 5.The Doppler PSD of different models.(a)(b)Doppler PSD of the improved model;(c)(d)Doppler PSD of the model in[7].

Fig.6 shows a comparison between the PDP of the NGSM in[8]and the improved model in each scenario:UIC,UOC,OHT,S,and OLT [8].As can be seen from the figure,both in the NGSM [8] and the improved model,the spread delay in UIC scenario is more severe than others.This is because in the UIC scenario,antenna is inside the vehicles,resulting in more obstacles in channel propagation.We can also notice that in OLT scenario,the scattering and reflection caused by moving vehicles are less than other scenarios with short spread delay,and the energies are concentrated in the first tap,which is also confirmed in the literature [27].Furthermore,it is obvious that PDP of two models for each scenario has some difference.The cause for this difference is the presence of the dominant LoS component in the improved model.Comparatively speaking,the LoS component is successfully added in the improved mod?el and the energy is more centered in the first path.In this way,the proportion of energy in other paths has a visible de?cline and the fading is more obvious,which means multipath effect is more apparent as well.Therefore,the comparison of PDP also validates that the improved model properly includes the LoS component.

As can be seen from the above analysis,we compare the NGSM in [8] with the improved model,and the results can be shown in Table 2.

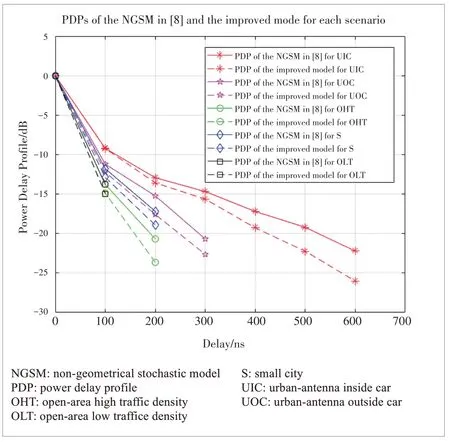
▲Figure 6.PDP of the NGSM in [8] and the improved model for each scenario.

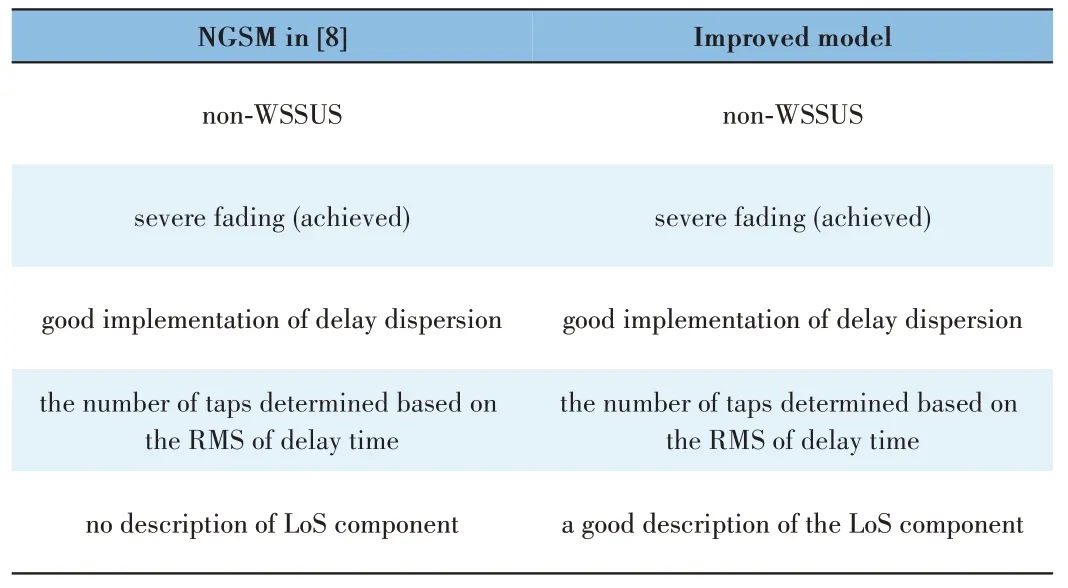
▼Table 2.Comparison of the NGSM in[8]and the improved model

Table 2.shows the comparison results between the NGSM in [8] and the improved model.We can readily observe that both models are based on the assumption of non-WSSUS and describe the severe fading by means of Weibull distribution.In addition,the improved model remains the tap correlation co?efficient matrices of the NGSM [8] fortunately,realizing the correlated scattering between each tap.Moreover,from the simulation results of the PDP,it is also obvious that both mod?els implement delay dispersion well.However,compared with the NGSM [8],the improved model includes a dominant LoS component into the Doppler PSD and thus has the ability to de?scribe the presence of LoS component.Consequently,we can conclude that the improved model fills the aforementioned gap and is more accurate to describe the characteristics of V2V channels.

4 Conclusions

In this paper,a novel NGSM for non-WSSUS V2V channels has been proposed,which is based on a conventional NGSM in[8].The proposed NGSM employs a method of generating nonuniformly distributed phase in the Weibull distribution,which extends the NGSM [8] to include the LoS component.It also has been demonstrated by the simulation results that compared with the NGSM [8],the proposed model has added a dominant LoS component into Doppler PSD and thus has explicitly iden?tified the presence of LoS component.Furthermore,the com?parison of the PDP has shown that the energy is more centered in the first path in the proposed model.Therefore,it has been verified by the simulation results that the proposed model is more accurate to describe the characteristics of V2V channels.