李偉佳,張萬里,林安

(重慶師范大學(xué)數(shù)學(xué)科學(xué)學(xué)院,重慶 401331)

近似鄰近點算法收斂性的一個注記

李偉佳,張萬里,林安

(重慶師范大學(xué)數(shù)學(xué)科學(xué)學(xué)院,重慶 401331)

近似鄰近點算法在最優(yōu)化理論與方法研究中具有重要作用.在不同誤差準(zhǔn)則下,近似鄰近點算法具有不同的收斂性.利用極大單調(diào)算子等工具給出了一個具體的例子,解釋了在一些誤差準(zhǔn)則下近似鄰近點算法的收斂性.

近似鄰近點算法;極大單調(diào)算子;誤差準(zhǔn)則

1 引言及預(yù)備知識

1970年,Martinet在文獻(xiàn)[1]中首次提出了鄰近點算法.1976年,Rockafellar在文獻(xiàn)[2]中運用鄰近點算法求解極大單調(diào)算子的零點.在實希爾伯特空間H中,極大單調(diào)算子T為H?H的集值映射.Rockafellar將集值映射方程的解作為新的迭代點,其中由于 Minty在文獻(xiàn)[3]中證明了該方程解的唯一性,所以其中Pk=I+ckT-1,I為H上的恒等映射.Rockafellar在文獻(xiàn)[2]中進(jìn)一步提出了近似鄰近點算法其誤差準(zhǔn)則為以下兩種：

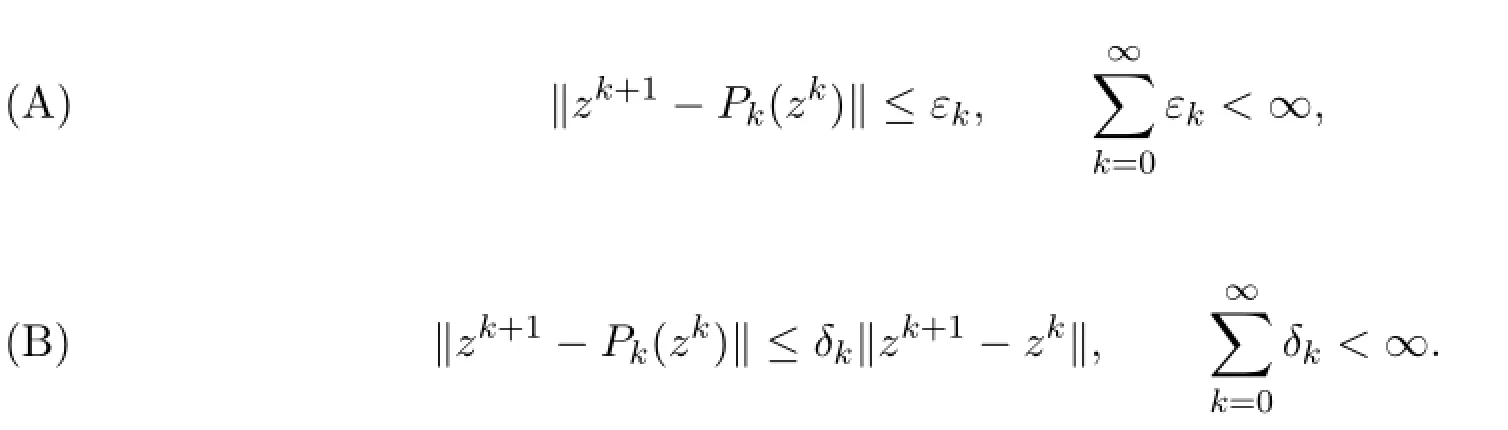
準(zhǔn)則(A)保證了鄰近點算法的收斂性.在該準(zhǔn)則下,Rockafellar將的解作為新的迭代點.進(jìn)一步,文獻(xiàn)[4-6]推廣了近似鄰近點算法,研究了相應(yīng)算法的收斂性.本文主要通過具體例子解釋了將收斂準(zhǔn)則(A)削弱為

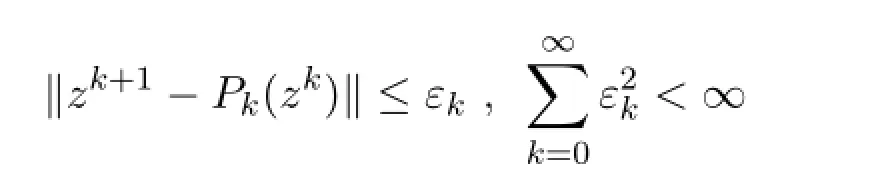
的情況下,近似鄰近點算法不必收斂.下面給出本文將用到的一些基本概念：

定義 1.1[2]設(shè)H為實希爾伯特空間,其內(nèi)積記為〈·,·〉.稱集值映射T：HH為單調(diào)算子.如果對于任意的z,z′∈H和任意的w∈T(z),w′∈T(z′)均有〈z-z′,w-w′〉≥0.

定義 1.2[2]設(shè)H為實希爾伯特空間,其內(nèi)積記為〈·,·〉.稱集值映射T：HH為極大單調(diào)算子.如果T為單調(diào)算子且T的圖像

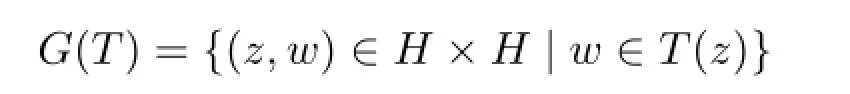
不真包含于任何其他單調(diào)算子T′：HH的圖像.

2 近似鄰近點算法收斂性的注記

為了討論近似鄰近點算法的收斂性,我們給出下面的一些引理.

引理2.1定義集值映射T：

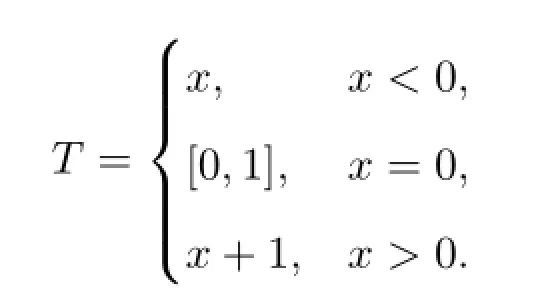
則T為極大單調(diào)算子.

引理2.2令

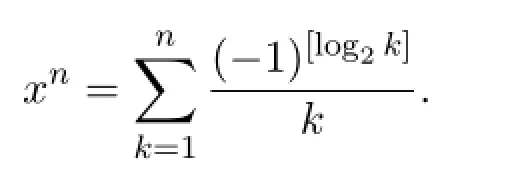
則{xn}為有界數(shù)列且滿足xn∈[0,1].

定理2.1在準(zhǔn)則

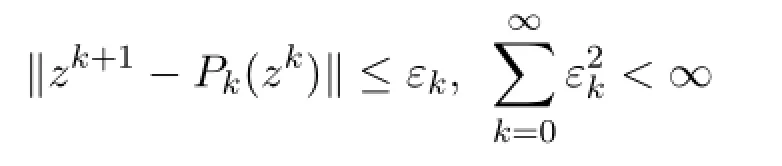
下,近似鄰近點算法不必收斂.

例2.1令

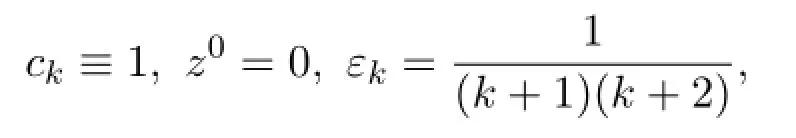
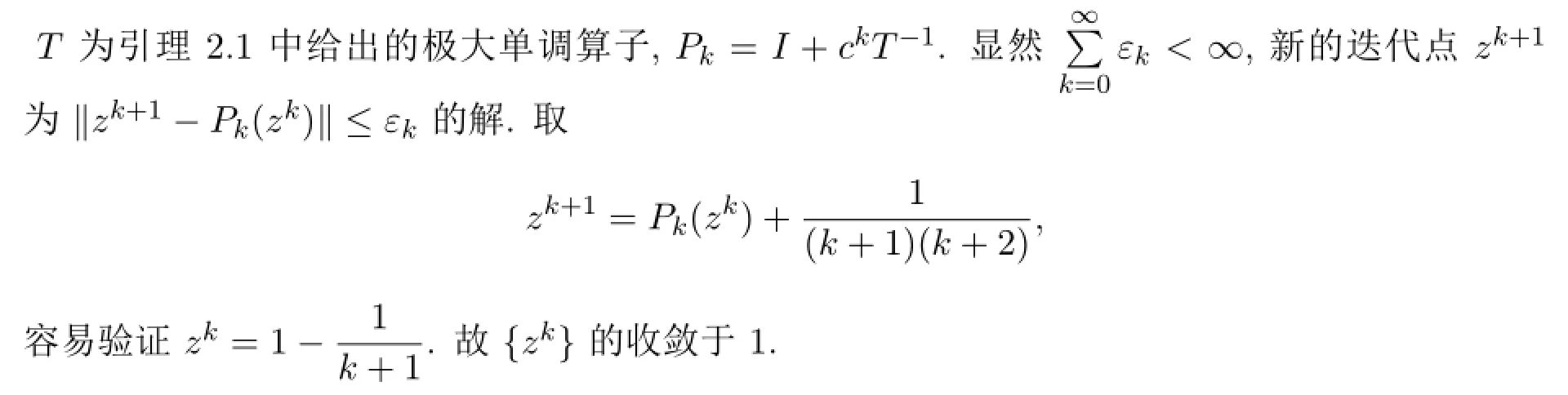
[1]Martinet B.Régularisation d′inéquations variationnelles par approximations successives[J].Rev.Francaise Informat.Recherche Opérationnelle,1970,4：154-158.

[2]Rockafellar R T.Monotone operators and the proximal point algorithm[J].SIAM Journal on Control and Optimization,1976,14：877-898.

[3]Minty G J.Monotone(nonlinear)operators in Hilbert space[J].Duke Mathematical Journal,1962,29：341-346.

[4]Chen Zhe,Zhao Kequan.A proximal-type method for convex vector optimization problem in Banach spaces[J].Numerical Functional Analysis and Optimization,2009,30：70-81.

[5]Chen Zhe,Huang Haiqiao,Zhao Kequan.Approximate generalized proximal-type method for convex vector optimization problem in Banach spaces[J].Computers and Mathematics with Applications,2009,57：1196-1203.

[6]Chen Zhe,Huang Haiqiao,Zhao Kequan.Proximal augmented Lagrangian and approximate optimal solutions in nonlinear programming[J].Journal of Applied Mathematics and Informatics,2009,27：149-159.

A note for the convergence of approximate proximal point algorithm

Li Weijia,Zhang Wanlin,Lin An
(College of Mathematics Science,Chongqing Normal University,Chongqing 401331,China)

Approximate proximal point algorithm has been playing an important role in optimization theory and methods.Under various approximate calculation,approximate proximal point algorithm has different convergence.In this paper,by means of some tools including as maximal monotone operator etc.,a concrete example is presented to illustrate the convergence of approximate proximal point algorithm with some approximate calculations.

approximate proximal point algorithm,maximal monotone operator,approximate calculation

O221.2

A

1008-5513(2016)06-0624-06

10.3969/j.issn.1008-5513.2016.06.008

2015-03-19.

國家自然科學(xué)基金(11301574);第二批重慶市高等學(xué)校青年骨干教師資助計劃;重慶市研究生科研創(chuàng)新項目(CYS15154).

李偉佳(1990-),碩士生.研究方向：最優(yōu)化理論與方法.

2010 MSC:90C30,90C48