Effects of Solvent and Impurities on Crystal Morphology of Zinc Lactate Trihydrate*
(楊薌鈺)(錢剛)(張相洋)(段學(xué)志)(周興貴)**
State Key Laboratory of Chemical Engineering, East China University of Science and Technology, Shanghai 200237, China
Effects of Solvent and Impurities on Crystal Morphology of Zinc Lactate Trihydrate*
YANG Xiangyu(楊薌鈺), QIAN Gang(錢剛), ZHANG Xiangyang(張相洋), DUAN Xuezhi(段學(xué)志)and ZHOU Xinggui(周興貴)**
State Key Laboratory of Chemical Engineering, East China University of Science and Technology, Shanghai 200237, China
The crystal morphology of zinc lactate trihydrate in the absence or presence of impurities (viz. succinic acid, L-malic acid and D-malic acid) is investigated by molecular simulation based on surface docking model and COMPASS force field. Combing simulation results with our previous experimental results, it is found that the solvent mainly has an inhibition effect on the (0 0 2) surface, and succinic acid impurity will inhibit the growth of (0 0 2) and (0 1 1) surfaces while two enantiomers of malic acid impurity will inhibit the (0 0 2) and (1 0 0) surfaces, which are in good agreement with the experimental results.
crystal morphology, molecular simulation, solvent effect, impurity adsorption
1 INTRODUCTION
Crystallization is widely used for separation and purification of food, fine chemicals and pharmaceuticals, and the mild operation is especially suitable for APIs (active pharmaceutical ingredients) processing [1]. The product purity is of primary concern for any separation and purification process. However, for solid products, in addition to the purity and the polymorphs, the morphologies are also very important because they have substantial impacts on downstream processing (such as filtering, washing and drying) and product performance (such as the rate of dissolution and the bioavailability of pharmaceuticals) [2]. A tight control of the crystal morphology, in addition to the purity, is therefore of great significance in industry.
The crystal morphology is essentially determined by the internal structure of crystal. Hence, a variety of theories and models relating crystal growth and morphology to crystal lattice have been proposed. However, the morphologies of most crystals are also easily influenced by external factors such as temperature [3], supersaturation [4], solvent [5, 6] and impurity [7]. These factors, especially the solvent or trace amount of impurities, have dramatic effects on crystal nucleation and crystal growth, and hence the crystal morphology. Nevertheless, the effects induced by these factors are still not well understood.
Solvent, among all the external factors that have influences on the morphology of crystal, should be treated firstly before any other factors. It changes the final crystal morphology by altering the relative growth rate of crystal surfaces [8]. There are two mechanisms for a solvent to alter the relative growth rate. First, because of the adsorption of solvent on the crystal surface, an additional energy barrier for solvent desorption is required for crystal growth [9, 10]. Therefore, it tends to decrease the growth rate. Second, the adsorption of solvent on the crystal surface may provide a surface structure and environment that facilitate the adsorption of solute molecules. In this case, it increases the growth rate [4, 11].
In addition, industrial solutions are invariably impure, and the impurities in the crystallizing solution can modify crystal morphology by stunting the growth of crystal in certain directions. From the thermodynamic point of view, the growth will be stopped by impurities if the supersaturation is lower than the critical supersaturation, i.e., the residual supersaturation threshold [12, 13], and the growth will be slowed if the supersaturation is above the critical value. From the kinetic point of view, the regular docking sequence of oncoming solute molecules will be interrupted, since the active sites on the surface are occupied by impurity molecules [7]. In some cases, impurities can actually build into crystal lattice.
As crystallization process can be depicted as a molecular recognition procedure on interface, the effects of solvent and impurity can then be explained in the manner of molecular interactions among crystal molecules, solvent molecules and impurity molecules. Molecular modeling techniques make the estimation of the atom-atom interactions possible [14]. Moreover, molecular simulation gives a well pre-understanding of crystal behavior, which helps experimental design and observation.
Bravais-Friedel-Donnay-Harker (BFDH) and attachment energy (AE) methods are generally used to predict the morphology of pure crystal. Based on the AE model, the influence of solvent and impurity are invested by simulating the relationship between foreign molecules and crystal molecules on interface. Kiang et al. [1, 15] predicted the habit and surface energetics of a pharmaceutical compound and validatedthe simulation result with experimental observation by scanning electron microscope (SEM), X-ray powder diffraction (XRPD) and atomic force microscope (AFM). Leeuw and Cooper [16] considered different interaction sites of a perfect crystal surface and found that the adsorbate would preferentially attach to either the acute or the obtuse steps. Dang et al. [17] found that cations appeared to have significant effect on phosphoric acid hemihydrate crystal shape than anions. These classical approaches are the first important steps in morphological analysis, despite the fact that they are not adequate for quantitative predictions.
Zinc lactate trihydrate [(CH3CH(OH)COO)2Zn·3H2O, ZLT] is extensively used as food additives. The ZLT may contain several impurities, as coming from the upstream fermentation process for lactic acid production, which may affect the morphology of ZLT during crystallization. To facilitate the downstream processing of ZLT or to make the final product more aesthetic, some additives may be introduced to control the morphology of ZLT during crystallization. Despite a large number of experimental studies of impurities effects on crystal growth, a generally accepted molecular level description based on the crystal-impurity interactions has not been provided.
In the present work, the effect of solvent and some selected impurities, e.g. (D-, or L-) malic acid, and succinic acid, on crystal morphology of ZLT is investigated by molecular simulation. These impurities are chosen because of their structural similarity with lactate group in ZLT molecule, and they are expected to have strong effect on growth process of ZLT. With a properly chosen force field, the crystal morphologies of ZLT in presence of different impurities are predicted, which agree very well with the present experimental observation.
2 METHODS
Molecular simulation of the ZLT morphology is performed on Material Studio 4.0, with Forcite and Morphology packages and using the COMPASS (Condensed-phase Optimized Molecular Potentials for Atomistic Simulation Studies) force field [18]. Forcite is used to optimize the crystal structure and to study the adsorption effect of foreign molecules, while Morphology is used to calculate the pure crystal morphology.
First, geometry optimization with the Smart algorithm is carried out for potential energy minimization of an initial unit cell of ZLT. Then BFDH law and AE method [19] are used to calculate the pure crystal morphologies. Because both methods were originally designed to predict the morphologies from either internal arrangement or crystal molecular interactions, and can not be used directly for the prediction of crystal morphologies in the presence of foreign molecules, the surface docking approach [20, 21] is applied to predict the influence of solvent and different impurities on the crystal morphology of ZLT. The surface docking approach consists of the following two steps.
(1) Based on the optimized structure of ZLT, the main facets are cleaved for the calculation of the adsorption effects of foreign molecules. All surfaces are rebuilt to be at least 3.0 nm in surface dimensions in order to better represent the bulk material. A threedimensional periodical cell of crystal vacuum slab is then created. The thickness of vacuum is set to 5.0 nm, so that the non-bond interactions can reach their asymptotic values.
(2) A foreign molecule is docked randomly on a given surface. Minimization is performed firstly for a proper initial position. Then, dynamics (with the NVT ensemble) is carried out for a geometrically and energetically optimal adsorption configuration. During dynamics simulation, all the atoms are taken into account in the energy expression of COMASS forcefield, but the bulk crystal molecules are constrained by fixing their Cartesian coordinates. As for the foreign molecule, no constraints are enforced and it is absolutely free to move in any directions. After dynamics computation, the structure of the foreign molecule is minimized, and the binding effects of adsorbates on crystal surfaces are calculated by

A higher absolute value of Eadsorptionindicates a stronger binding effect of impurity molecules.
Seeded batch cooling crystallization experiments were carried out to investigate the growth kinetics and the growth morphology in presence of impurities such as malic acid, succinic acids, etc. The detailed experimental procedures are referred to Zhang et al [13, 22]. Fig. 1 shows the habits produced in pure and impure aqueous solutions. Correspondingly, the XRD (X-ray diffraction) diagrams of the crystals grown from pureand impure mother liquors are shown in Fig. 2. The positions of the main peaks are the same, but the relative intensities of the peaks vary, indicating that the crystals grown from different conditions have different preferential orientations.
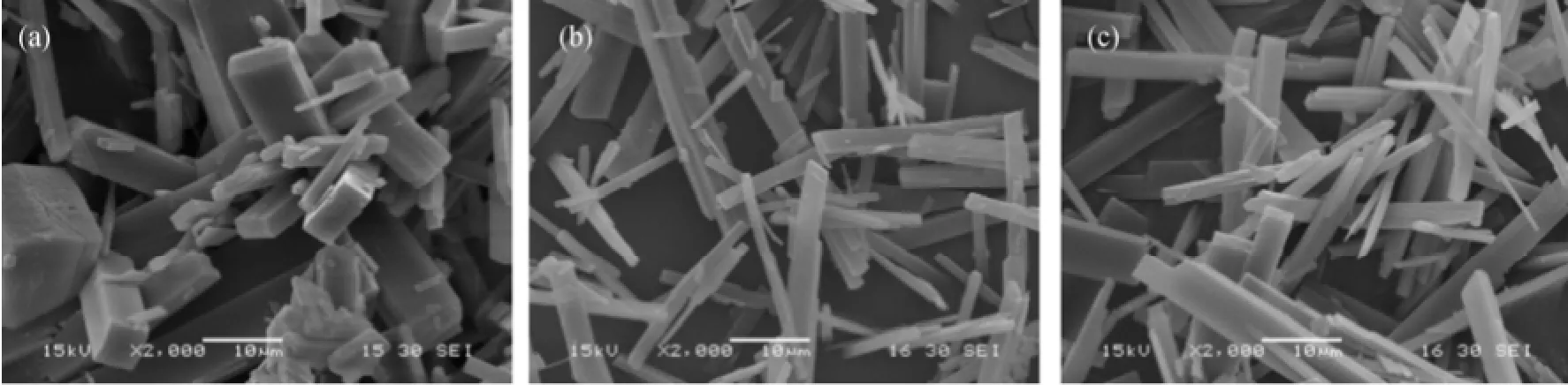
Figure 1 SEM images of ZLT grown in (a) pure solution and in presence of (b) malic acid and (c) succinic acid

Figure 2 Comparison of XRD spectra diagrams of ZLT from simulation and experiments
3 RESULTS AND DISCUSSION
3.1 Crystal structure
The crystal structure of ZLT, including the unit cell dimensions, space group and atom coordination has already been determined by Singh et al. [23]. It has a monoclinic structure (a=0.938 nm, b=0.583 nm, c=2.200 nm, β=90.9°) with P21/c space group. However, the structure is not complete because there are 6 undefined light hydrogen atoms. An initial unit cell is then built on the basis of the structure parameters determined by Singh et al., and the 6 undefined light hydrogen atoms are added in a random manner. Geometry optimization is then performed on this unit cell which reduced the total energy of the unit cell significantly. With the structure optimized, its powder X-ray diffraction pattern is computed (by assuming the crystals are 500 μm in all three dimensions), and is found to be well in line with that determined by experiment, as shown in Fig. 2. The difference between the peak intensities can be attributed to the different size distribution of the crystals prepared in experiments.
The COMPASS forcefield is also used to determine the unit cell of malic acid and succinic acid crystals, and the structure parameters are found to agree well with those available from that reported in Cambridge Crystallographic Data Centre (CCDC). This authenticates the validity of the COMPASS forcefield for this system.
3.2 Crystal morphology
The BFDH method and AE method are used to predict the habit of ZLT, and the results are presented in Fig. 3. Both methods predict a habit with the same three dominant facets, but the area percentages of the facets are very different (Table 1). The habit predicted by the BFDH method has a cube-like appearance, while the habit predicted by AE method, which is more close to the experimental pattern under a microscope (Fig. 1), is less developed along z-axis.

Table 1 The facet area percentages of ZLT habit calculated from BFDH and AE method
The zinc ions in ZLT crystal form a distorted octahedron arrangement with six oxygen atoms, four from lactate groups and two from water groups, and there are strong non-bond interactions in the unit cell. Crystal graph procedure helps to estimate the non-bond interactions between crystal molecules (Table 2). These interactions reflect the growth orientation and play an important role in determining the crystal habit. However, in the BFDH method, only the submultiples of the interplanar spacing are taken into account, and the energy, types of atoms and bonds, and charge distribution within the bulk crystals are not considered. In the AE method, both the crystal arrangement and the non-bond interactions are considered, the later being measured by the attachment energy which further determines the shape of the crystal [24-26]. Thus,the habit from AE method can be more accurate comparing with that from the BFDH method.

Table 2 The main non-bond interactions in ZLT crystal cell

Figure 3 Simulated crystal habits of ZLT through BFDH (left) and AE (right) methods

Figure 4 Cleaved crystal surfaces along the habit facets

Figure 5 Solvent effect on ZLT crystal
Based on the optimized crystal cell of ZLT, the main crystal surfaces are prepared. On the facets, different functional groups with diverse orientations and densities are exposed, as shown in Fig. 4. Only the methyl groups are exposed on top of (0 0 2) and (1 0 0) surface, while on (0 1 1) surface several functional groups are exposed. Thus, in comparison with (0 0 2) and (1 0 0), the (0 1 1) surface, the polar groups on it in particular, would be more appealing to the oncoming molecules.
Moreover, the lactate groups form two ring structures with the zinc atoms, and the dihedral angles between the rings and the surface planes are different on these three facets. The two rings of the ZLT molecule incline toward the (0 1 1) facet with very small angles. In contrast, these rings are nearly perpendicular to the (1 0 0) facet and almost exactly perpendicular to the (0 0 2) facet. In the view of molecular dynamics, the vertical arrangement of the rings will hinder the surface diffusion of adsorbed solute molecules owing to steric hindrance. (0 1 1) surface is therefore expected to grow the fastest, while (0 0 2) surface grows the slowest and tends to be present in a large area percentage in the final crystals.
3.3 Solvent effect
Figure 5 (a) depicts the optimized configurations of an adsorbed water molecule on the three crystal surfaces. In order to compare the competition of the adsorption between the solvent and the solute molecules during crystal growth, the binding of ZLT molecules onto crystal surface were also determined, as shown in Fig. 5 (b). The result shows that H2O will preferentially adsorb on each surface (Table 3). However, the optimal adsorption sites of H2O are not always the active docking sites of ZLT molecules. On (0 0 2) surface, the H2O molecule forms strong hydrogen bonds with two adjacent crystal molecules, and so does the oncoming solute molecule. The adsorbedH2O molecule will therefore block the binding of ZLT molecule, and retard the crystal growth on this surface. On (1 0 0) and (0 1 1) surfaces, the H2O molecule adsorbs on a site close to that of the solute molecule, and the polarity of the H2O molecule will facilitate the deposition of ZLT molecule. Besides, the intrinsic growth rate of ZLT from the AE model, Rrel(0 0 2)< Rrel(1 0 0) Table 3 Adsorption energies (kJ·mol?1) of solvent andsolute molecules on different crystal surfaces 3.4 Impurity effect Of the two impurities used, malic acid has two enantiomers, while succinic acid has no enantiomorphism. Because of the enantiomorphism of malic acid, the adsorption of L-malic acid and D-malic acid on antipodal surfaces of a crystal is studied. The calculated adsorption energies are listed in Table 4. Because of the strong non-bond interactions, the impurities are strongly adsorbed on the surfaces, which slow down the relative growth rate. The conformations and the adsorption energies of the two enantiomers of malic acid are almost identical, and the effect of enantiomorphism is hardly discriminable. In addition, the enantiomorphs can not be resolved via adsorption on antipodal surfaces of ZLT crystal, as revealed by dynamics simulation. The adsorption energy sequence is Eads(0 0 2)>Eads(0 1 1)>Eads(1 0 0). Therefore, the morphology has expanded (0 0 2) and (0 1 1) surfaces and a shrunk (1 0 0) surface. Table 4 Adsorption energies (kJ·mol?1) of impurities on the three crystal surfaces In contrast, the modification effect of succinic acid on the ZLT morphology is different. The inhibition sequence of succinic acid on ZLT crystal surfaces is Eads(0 0 2)>Eads(1 0 0)>Eads(0 1 1), and the intrinsic growth rate sequence is Rrel(0 0 2) Molecular simulation has been performed to predict the crystal morphology of ZLT in the absence or presence of impurities. For pure ZLT crystals, the AE method is a more accurate method to predict the crystal morphology than the BFDH method. The surface-docking approach is employed to predict the effects of solvent and impurities on the crystal morphology of ZLT, and the results show that the inhibiting effect of H2O mainly occurs on (0 0 2) surface, and as for impurities, malic acid and succinic acid inhibit the growth of ZLT crystal facets with an order of (0 0 2)>(0 1 1)> (1 0 0) and (0 0 2)>(1 0 0)>(0 1 1), respectively. Moreover, the effects of both enantiomers of chiral malic acid on antipodal faces of ZLT crystal are the same. This agrees well with the experimental results of thinner morphology in the presence of malic acid and the longer habit in the presence of succinic acid. 1 Kiang, Y.H., Yang, C.Y., Staples, R.J., Jona, J., “Crystal structure, crystal morphology, and surface properties of an investigational drug”, Int. J. Pharm., 368 (1-2), 76-82 (2009). 2 Duan, X., Wei, C., Liu, Y., Pei, C., “A molecular dynamics simulation of solvent effects on the crystal morphology of HMX”, J. Hazard. Mater., 174 (1-3), 175-180 (2010). 3 Poornachary, S.K., Chow, P.S., Rrginald, B.H., “Effect of solution speciation of impurities on α-glycine crystal habit: A molecular modeling study”, J. Cryst. Growth, 310 (12), 3034-3031 (2008). 4 Bennema, P., “Theory of growth and morphology applied to organic crystals; possible applications to protein crystals”, J. Cryst. Growth, 122 (1-4), 110-119 (1992). 5 Segura, J.J., Verdaguer, A., Garzón, L., Barrena, E., Ocal, C., Fraxedas, J., “Strong water-mediated friction asymmetry and surface dynamics of zwitterionic solids at ambient conditions: L-alanine as a case study”, J. Chem. Phys., 134 (12), 124705 (2011). 6 Blagden, N., “Crystal engineering of polymorph appearance, the case of sulphathiazole”, Powder Technol., 121 (1), 46-52 (2001). 7 Enckevort, W.J.P., Berg, A.C.J.F., Kreuwel, K.B.G., Derksen, A.J.,Couto, M.S., “Impurity blocking of growth steps: Experiments and theory”, J. Cryst. Growth, 166 (1-4), 156-161 (1996). 8 Myerson, A.S., Handbook of Industry Crystallization, Elsevier, Boston (2001). 9 Lahav, M., Leiserowitz, L., “The effect of solvent on crystal growth and morphology”, Chem. Eng. Sci., 56 (7), 2245-2253 (2001). 10 Horst, J.H., Geertman, R.M., Heijden, A.E., Rosmalen, G.M., “The influence of a solvent on the crystal morphology of RDX”, J. Cryst. Growth, 198-199, 773-779 (1999). 11 Bourne, J.R., Davey, R.J., “The role of solvent-solute interactions in determining crystal growth mechanisms from solution: I. The surface entropy factor”, J. Cryst. Growth, 36 (2), 278-286 (1976). 12 Kubota, N., “Effect of impurities on the growth kinetics of crystals”, Cryst. Res. Technol., 36 (8-10), 749-769 (2001). 13 Zhang, X.Y., Févotte, G., Zhong, L., Qian, G., Zhou, X.G., Yuan, W.K., “Crystallization of zinc lactate in presence of malic acid”, J. Cryst. Growth, 312 (19), 2747-2755 (2010). 14 Piana, S., Gale, J.D., “Understanding the barriers to crystal growth, dynamical simulation of the dissolution and growth of urea from aqueous solution”, JACS, 127 (6), 1975-1982 (2005). 15 Kiang, Y.H., Shi, H.G., Mathre, D.J., Xu, W., Zhang, D., Panmai, S.,“Crystal structure and surface properties of an investigational drug—A case study”, Int. J. Pharm., 280 (1/2), 17-26 (2004). 16 Leeuw, N.H., Cooper, T.G., “A computer modeling study of the inhibiting effect of organic adsorbates on calcite crystal growth”, Cryst. Growth Des., 4 (1), 123-133 (2004). 17 Dang, L.P., Wei, H.Y., Zhu, Z., Wang, J.K., “The influence of impurities on phosphoric acid hemihydrate crystallization”, J. Cryst. Growth, 307 (1), 104-111 (2007). 18 Sun, H., “COMPASS: An ab initio force-field optimized for condensed-phase applications-overview with details on alkane and benzene compounds”, J. Phys. Chem. B, 102 (38), 7338-7364 (1998). 19 Hartman, P., Bennema, P., “The attachment energy as a habit controlling factor: I. Theoretical considerations”, J. Cryst. Growth, 49 (1), 145-156 (1980). 20 Lu, J.J., Ulrich, J., “The influence of supersaturation on crystal morphology—Experimental and theoretical study”, Cryst. Res. Technol., 40 (9), 839-846 (2005). 21 Lu, J.J., Ulrich, J., “Improved understanding of molecular modeling— The importance of additive incorporation”, J. Cryst. Growth, 270 (1-2), 203-210 (2004). 22 Zhang, X.Y., “Crystallization behaviour of zinc lactate in presence of impurities”, Ph. D. Thesis, East China University of Science and Technology, Shanghai (2010). (in Chinese) 23 Singh, K.D., Jain, S.C., Sakore, T.D., Biswas, A.B., “The crystal and molecular structure of zinc lactate trihydrate”, Acta Crystallogr. Sect. B, 31, 990-993 (1975). 24 Horst, J.H., Kramer, H.J., Rosmalen, G.M., Jansens, P.J., “Molecular modelling of the crystallization of polymorphs. Part 1, The morphology of HMX polymorphs”, J. Cryst. Growth, 237-239, 2215-2220 (2002). 25 Chen, J., Wang, J., Zhang, Y., Wu, H., Chen, W., Guo, Z., “Crystal growth,structure and morphology of hydrocortisone methanol solvate”, J. Cryst. Growth, 265 (1/2), 266-273 (2004). 26 Poornachary, S.K., Chow, P.S., Rrginald, B.H., “Impurity effects on the growth of molecular crystals-experiments and modeling”, Adv. Powder Technol., 19 (5), 459-473 (2008). Received 2012-02-21, accepted 2012-11-30. * Supported by the Shanghai Commission of Science and Technology (06SR07108) and the OpenProject of State Key Laboratory of Chemical Engineering (08C08). ** To whom correspondence should be addressed. E-mail: xgzhou@ecust.edu.cn

4 CONCLUSIONS
REFERENCES
 Chinese Journal of Chemical Engineering2014年2期
Chinese Journal of Chemical Engineering2014年2期
