Efficient ion acceleration driven by a Laguerre-Gaussian laser in near-critical-density plasma
Jia-Xiang Gao(高嘉祥) Meng Liu(劉夢), and Wei-Min Wang(王偉民),3,4,?
1Department of Physics and Beijing Key Laboratory of Optoelectronic Functional Materials and Micronano Devices,Renmin University of China,Beijing 100872,China
2Department of Mathematics and Physics,North China Electric Power University,Baoding 071003,China
3Key Laboratory of Quantum State Construction and Manipulation(Ministry of Education),Renmin University of China,Beijing 100872,China
4IFSA Collaborative Innovation Center,Shanghai Jiao Tong University,Shanghai 200240,China
Keywords: Laguerre-Gaussian laser, laser-driven ion acceleration, particle-in-cell simulations, near-criticaldensity plasma
1.Introduction
With the recent rapid development of ultrashort ultraintense lasers,proton beams with cutoff energy of near 100 MeV can be generated from the interaction of intense lasers with solid foils.[1-4]A few features of the laserdriven ion accelerator, including higher ion density and shorter bunch or more compact size,[5,6]can be applied in various prospective areas,covering proton radiography for cancer treatment,[7]fast ignition of nuclear fusion,[8]and nuclear physics,[9,10]etc.However,a quasi-monoenergetic beam with low divergence,[11]is still a critical requirement for most of these attractive applications under the present laboratory conditions.Usually, the proton beams have a divergence up to tens of degrees generated in experiments.[12-14]
To overcome this limitation,significant efforts have been devoted to concentrate the transversely distributed proton beam at the center.For instance,funnel-like target,[15]curved targets,[16]density-modulated targets embedded in a substrate thick enough,[17]and plane targets with rectangular and cylindrical geometries at the back,[18]have been widely used to control the ion trajectory near the laser propagation axis.Improving the beam focusing properties will enable the chargeseparated fields on the surface of the structure targets to efficiently guide proton beams.In addition, a new type of movable electrostatic field with a helical wire excited by laser irradiating on a target can concentrate and accelerate the protons inside simultaneously.[19]However, the application of these laser-driven proton beams is restricted by the expense of the complex assembled target.It is highly desired to promote simple methods to generate a quasi-monoenergetic proton beam with low divergence for the coming petawatt (PW)laser systems.Recently,with the application of supersonic gas jet and nanosecond laser reshaping technology, the manufacture of a near-critical-density(NCD)plasma has made remarkable progress,[20-24]and there are some experimental results of laser-driven ion acceleration based on laser NCD plasmas interaction.[24,25]
In this paper,we propose to adopt a relativistic Laguerre-Gaussian (LG) laser pulse with an intrinsic hollow intensity distribution to accelerate and focus a proton beam in a nearcritical-density(NCD)target.Such an LG laser pulse with an intensity above 1019W·cm-2has been available,[26]and even a higher intensity LG laser of 1022W·cm-2could be delivered from the SULF 10 PW and SEL 100-PW laser facilities in the coming few years.[27]With the intensity ranging from 1021W·cm-2-1022W·cm-2,simulation results show that the hollow structure of the LG laser can compress the plasma at the center.[28]In this paper,we find that quasi-monoenergetic proton beams can be efficiently generated when an LG laser pulse interacts with an NCD target.The relativistic laser pulse can excite a large-amplitude longitudinal electrostatic field to accelerate the protons forward.Meanwhile, the centripetal distribution of this longitudinal electrostatic field substantially converges the protons near the laser propagating axis.While a conventional Gaussian laser pulse with the same intensity irradiates on an NCD plasma, the generated proton beam is usually non-monoenergetic in our PIC simulations.Moreover,the peak energy and the corresponding proton yields using the LG laser are higher than the case with the Gaussian laser pulse.
2.Vacuum field structure of a helical laser pulse
To obtain ultra-relativistic intensity LG laser pulse,a spirally varying thickness for the spiral phase plate should be used.[26]By making a paraxial approximation to the wave equation of LG laser in cylindrical coordinates,one can obtain the eigen solution of Laguerre Gaussian beam wave equation as follows:[29]
The laser is incident at the positionx=0 and propagates along the+xdirection.ris the distance from a spatial arbitrary point to thexaxis.φis the rotating angle around thexaxis.lis the azimuthal quantum number, which can take any integer.pis the radial quantum number, which can take any integer greater than or equal to 0.Andp <|l|should be required.Alllandptogether constitute a set of orthogonal complete LGlpbasis vectors, which can represent any spatial mode.For example,the vortex laser with ultra-relativistic laser intensity in the experiment mentioned above is obtained by the superposition of several low-order LG lasers in the form of incoherent superposition.[30]The Laguerre polynomialLlp(ζ)is
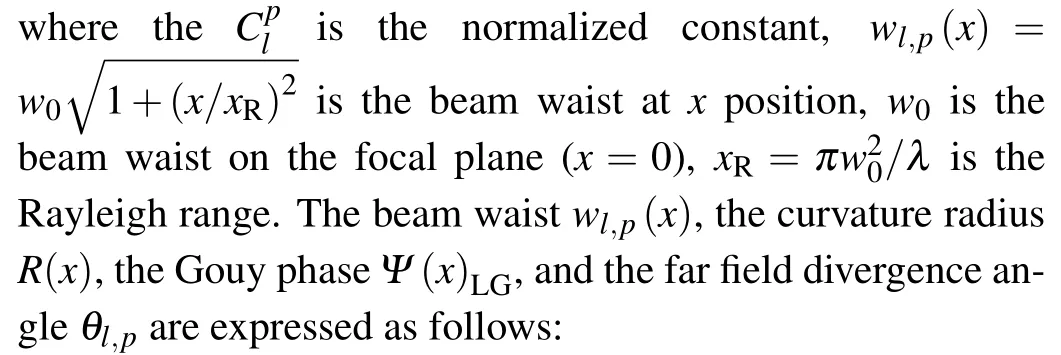
where the beam waistwg(x)and far-field divergence angleθgare the TEM00(transverse electromagnetic mode)of LG laser.According to Eq.(3), as the angular quantum number|l| and radial quantum numberpincrease, the beams diverge faster and faster as the propagation distance increases.Thus, the pulse energy of the high-order LG laser losses faster than the low-order LG laser.And a high-order LG laser is also more difficult to produce.So in this paper, we adopted the TEM00(l=0,p=0),i.e.,Gaussian pulse,and the low-order TEM10(|l|=1,p=0)LG pulse.
The amplitude profile of LG laser (aLG) and Gaussian laser(aG)is
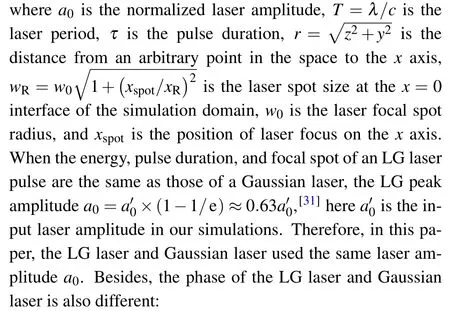
Equation(5)depicts that the LG laser has a helical phase varying withφ.Ψ(x)G=arctan(x/xR)is the Gaussian laser Gouy phase.
The electric field of the LG laser in cylindrical coordinates is as follows:[32-34]
wherep=0,Ll0(ζ)=1 is independentl.In this case, the transverse component perpendicular to the direction of propagation can be represented as
Hereη=kx+(kr2/2RC)-Ψ(x), and the longitudinal component parallel to the laser propagation direction is
A circularly polarized (CP) laser carries a spin angular momentum ofσ= 1.Hereσ= 1 andσ=-1 represent the left-handed CP laser and the right-handed CP laser,respectively.[35]Besides,each photon in the LG beam carries an orbital angular momentum(OAM)oflˉh.[36]Thus,the total angular momentum of each photon isJ=L+S,that is,each photon carries a total angular momentum of(l-σ)ˉh.In order to guarantee the LG pulse has an inward ponderomotive force and does not transfer its angular momentum to the protons during the interaction between the laser and an NCD plasma,the angular momentum of photons should equal 0.[37]
With the parameters ofa0=100,l=±1,p=0,σ=1,w0=7μm,we performed 2D PIC simulations for the LG laser interacting with an NCD plasma density ofne=10nc.The result indicates that the LG laser ofl=σ=1 can gain better acceleration when the laser only has focusing pondermotive force and will not transfer angular momentum to protons to impede the acceleration.In addition, according to Eq.(8),whenJ/=0,the LG beam will generate a changing longitudinal electric field varying with(l-σ)φ,which may hinder the formation of the uniform electron layer during the“hole boring” stage when laser irradiating on the plasma.[31]Thus, an LG laser ofl=1,p=0,σ=1 is applied in our simulations.
3.Simulation results
To achieve efficient ion acceleration using an LG laser pulse with an NCD plasma, we have carried out a series of two-dimensional(2D)particle-in-cell(PIC)simulations using the code EPOCH.[38]The CP laser pulse has a wavelength ofλ= 1 μm.The amplitude profiles of LG and Gaussian laser are determined by Eq.(4).The normalized amplitude of the CP laser pulsea'0?(I0λ2/2.74×1018)1/2.In typical simulations, a 30-fs laser pulse has the amplitudea'0from 60 to 120 and the beam waist sizew0=3λ.The wavefront of the laser pulse arrives at the vacuum-foil interfacex=10λatt=0.The semi-infinite NCD target is composed of protons H+and electrons e-with a uniform density profile.The densitynechanges from 6ncto 10ncas the laser intensity increases.The critical densitync=meω2/4πe2,ωis the angular frequency of the laser,meis the electron mass.In each simulation, a 45 μm×40 μm simulation box moves along thexaxis at the speed of light and is divided into 3600×1600 cells.An NCD plasma is located atx ≥10λand each cell has 50 macro-particles in the plasma region.Besides, the LG laser and Gaussian laser use the same laser amplitudea0,which has the following conversion relationship with the input LG laser amplitudea'0:a0?0.63a'0.
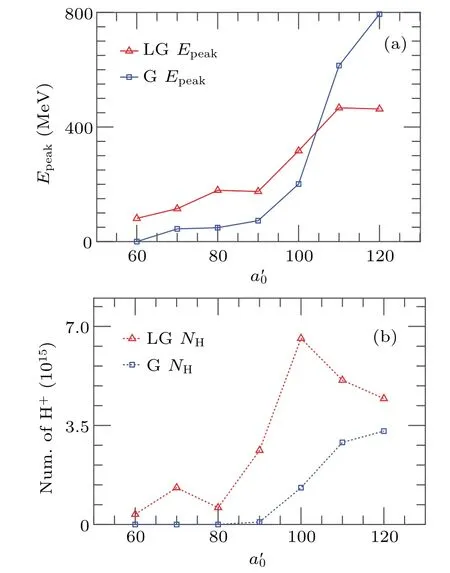
Fig.1.The proton peak energy Epeak (a)and the corresponding proton yield NH in energy peak(b).The red triangle lines are the interaction of LG with NCD plasma;the blue square lines are the cases for Gaussian beam.The laser beam waist w0=3λ,and the input laser amplitude a0 and the plasma density ne are taken as (60, 6nc), (70, 7nc), (80, 7nc),(90, 9nc), (100, 10nc), (110, 10nc), and (120, 10nc), respectively.We counted the protons at|y|≤3λ in panel(b).
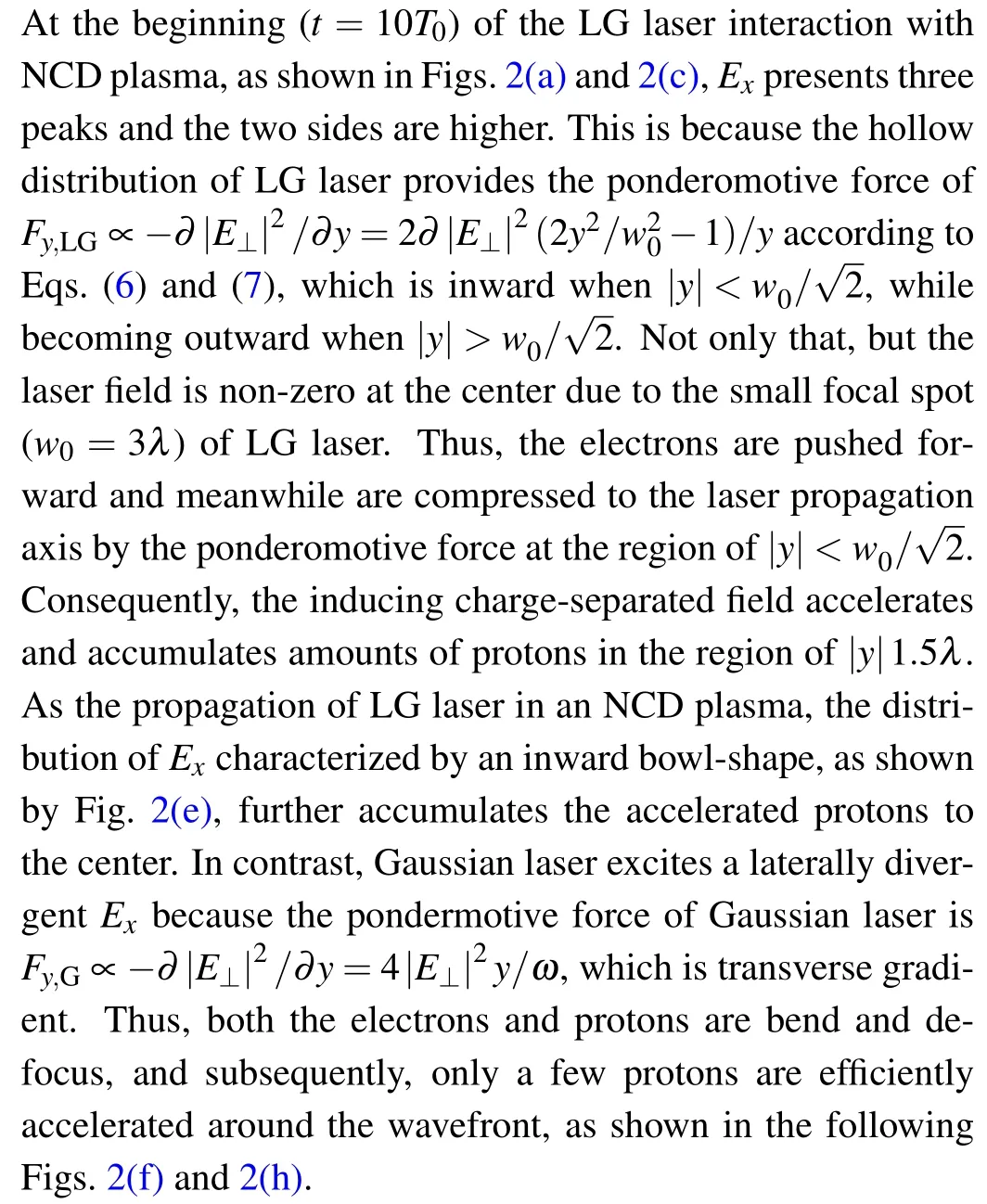
To characterize the accelerating fieldExexcited by LG laser and Gaussian laser in an NCD plasma, we compare the spatialtemporal evolution ofExand proton densitynHin Fig.3.Figures 3(a) and 3(c) indicate that the longitudinal electric fieldExexcited by LG laser has a greater intensity aty=3λthan that at the position ofy=0 due to the hollow distribution of laser field according to Eqs.(6) and (7).Furthermore,the propagating velocities ofExaty=0 andy=3λboth reach up to 0.6c(deriving from the slope of Figs.3(a)and 3(c),and then decrease to about 0.2c.These similar temporal evolutions ofExmaintain the inward bowl-shape distribution in Fig.2(e), which efficiently prompt lots of accelerated protons to accumulate around the laser propagating axis(y=0),as shown by Fig.3(e).On the contrary, figures 3(b)and 3(d)show thatExexcited by Gaussian laser has the highest intensity aty=0, while decreases quickly aty=3λ.And also,the propagating velocity can reach up to 0.7caty=0,which is much higher than the one aty=3λ.As a result, by combining Figs.2(h), 3(f), and 3(h), the stable accelerating field cannot maintain for a long duration, and thus the protons are expelled transversely rather than efficiently accelerated around the wavefront of Gaussian laser.
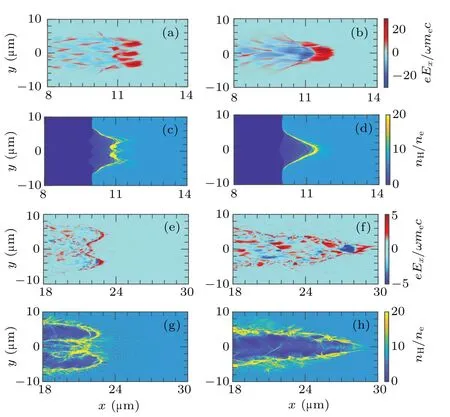
Fig.2.Spatial distribution of the longitudinal electrostatic field Ex [(a),(b), (e), (f)] and proton density nH/nc [(c), (d), (g), (h)].The comparisons of LG laser and Gaussian laser at t =10T0 are taken as [(a),(c)] and [(b), (d)]; the comparisons of LG laser and Gaussian laser at t=45T0 are taken as[(e),(g)]and[(f),(h)].The laser amplitude a'0 and target density ne are taken as(70,7nc).
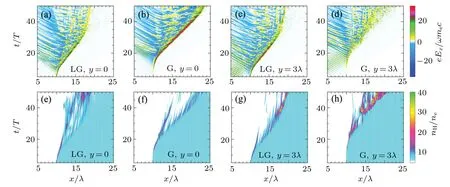
Fig.3.The temporal evolution of longitudinal electrostatic field distribution Ex [(a)-(d)] and proton density distribution nH [(e)-(h)].Panels [(a), (e)] and [(b), (f)] present the spatialtemporal evolutions of field at y=0 for LG laser and Gaussian laser, respectively.Panels [(c),(g)] and [(d), (h)] present the spatialtemporal evolutions of field at y=3λ for LG laser and Gaussian laser, respectively.The laser plasma parameters are the same as those in Fig.2.
Since an inward bowl-shape longitudinal electrostatic field, excited by the hollow field of LG laser, can move forward and maintain its spatial distribution for dozens of laser duration, the protons can be efficiently accelerated and centered.A proton beam with an energy peak ofEpdak?115 MeV is generated.The root-mean-square energy spread of this peak energy is aboutσ ?4.25 due to theand the corresponding divergence angle isθ <5°, as shown in Fig.4(a).In contrast, due to the repulsion to the protons by the outward pondermotive force of Gaussian laser, as shown in Fig.4(b),no high-energy proton was observed and the protons are dramatically divergent.We also show the phase spacepx-xof protons for LG laser and Gaussian laser,as shown by Figs.4(c)and 4(d),and the converged protons at|y|≤3λfor LG laser is accelerated topi≥0.2micbased on hole-boring radiation pressure.[39,40]
Besides, only by increasing the laser intensity to above 1022W·cm-2and enlarging the laser focal spot toω0=7λwe can get a quasi-monoenergetic peak ofEpdak?550 MeV for Gaussian laser,as shown in Fig.5(b).However,with the laser intensity ranging from 1021W·cm-2-1022W·cm-2available in the near future,[41,42]the LG laser pulse with small focal spot can excite a moving longitudinal electrostatic field in the NCD plasma with a bowl-shape distribution,achieving better proton acceleration.
We also employ the three-dimensional (3D) simulations when an LG laser with normalized amplitude ofa0=100 interacts with an NCD plasma ofne=10nc.Figure 6(a)shows that the protons converged to the peak density ofne?32ncat the center is a little lower than the peak density ofne?50ncfor 2D simulations.The corresponding energy peak ofEi?580 MeV, as shown by Fig.6(b), is higher than the peak ofEi?480 MeV in Fig.5(a).
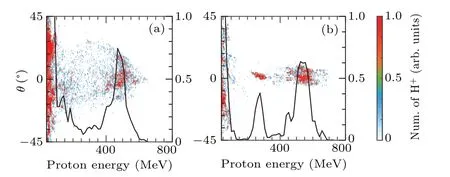
Fig.5.The comparison of the proton energy-angle (θ =arctan(vy/vx))between LG laser pulse (a) and Gaussian laser pulse (b) at t =55T0.We counted the protons at |y|≤7λ.The laser pulses have the focal spot size w0=7λ,and the laser amplitude and target density are taken as(100,10nc).
4.Conclusion
In summary,we have investigated by PIC simulation that a quasi-monoenergetic proton beam can be efficiently generated for an LG laser pulse with 1021W·cm-2-1022W·m-2in an NCD plasma.With a suitable density range of an NCD plasma, the longitudinal electrostatic field excited by LG laser can maintain the distribution characterized by the inward bowl-shape for dozens of laser durations.In this way,the protons can be accelerated and converged simultaneously by the electrostatic field,and therefore,a low-divergence proton beam with a peak energy of 115 MeV was obtained for LG laser withI0?5.3×1021W·cm-2.Our simulations have shown that the protons with no or much lower quasimonoenergetic peak energy are generated by the Gaussian laser with the same intensity.It is worth pointing out that this work could provide a reference for the efficient ion acceleration experiments with PW-class laser systems recently available.[41,42]
Acknowledgements
Project supported by the Strategic Priority Research Program of the Chinese Academy of Sciences (Grant No.XDA25050300), the National Natural Science Foundation of China (Grant No.12205366), the National Key Research and Development Program of China (Grant No.2018YFA0404801), the Fundamental Research Funds for the Central Universities (Grant No.2020MS138), and the Research Funds of Renmin University of China (Grant No.20XNLG01).
- Chinese Physics B的其它文章
- Corrigendum to“Reactive oxygen species in plasma against E.coli cells survival rate”
- Dynamic decision and its complex dynamics analysis of low-carbon supply chain considering risk-aversion under carbon tax policy
- Fully relativistic many-body perturbation energies,transition properties,and lifetimes of lithium-like iron Fe XXIV
- Measurement of the relative neutron sensitivity curve of a LaBr3(Ce)scintillator based on the CSNS Back-n white neutron source
- Kinesin-microtubule interaction reveals the mechanism of kinesin-1 for discriminating the binding site on microtubule
- Multilevel optoelectronic hybrid memory based on N-doped Ge2Sb2Te5 film with low resistance drift and ultrafast speed

