Γis clearly a closed convex cone with its vertex at 0. Next, (Γ)= chΓ, where chΓ is the convex hull of Γ. We say that cone Γ is regular if it is an open cone and the interior of its dual cone Γis non-empty.

2 Main Results

If 0 ＜p ＜∞and B is a nonempty connected open set in R, H(T) is the vector space of all holomorphic functions on T:= R+iB = {x+iy : x ∈R,y ∈B} and A(B) is the vector space of all holomorphic functions F ∈H(T) such that for any compact set K ?B,ρ(F)＜∞, where

If p ≥1, syggg00 is a separating family of seminorms and{V}is a convex local base for A(B).Therefore A(B) is a Fr′echet space [10] if p ≥1.

If p ≥1, we prove that A(B) has the Heine-Borel property. It will then follow from Theorem 1.23 in [10] that A(B) is not locally bounded, and hence is not normal.

If p ≥1, let E ?A(B) be closed and bounded, since the boundedness of E is equivalent to the existence of numbers M＜∞such that d(F) ≤Mfor any F ∈E, and there exists a δ＞0, K= {y : ?ξ ∈K,|ξ-y| ≤δ} ?K, based on the plurisubharmonic properties of |F|,

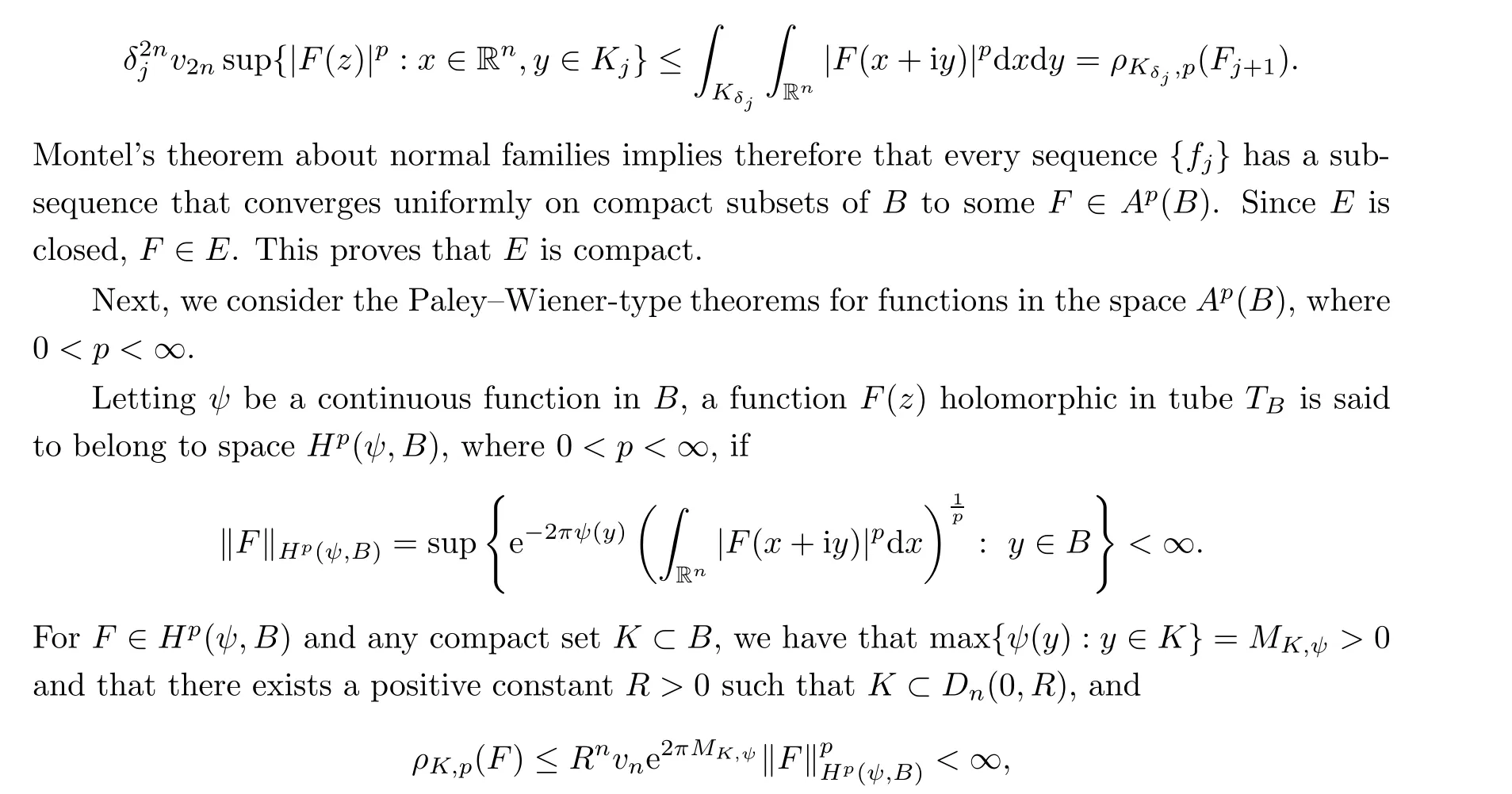
holds almost everywhere for t ∈R.