Determination of the thermal noise limit in test of weak equivalence principle with a rotating torsion pendulum?
Wen-Ze Zhan(占文澤),Jie Luo(羅杰),?,Cheng-Gang Shao(邵成剛),Di Zheng(鄭第), Wei-Ming Yin(殷蔚明),and Dian-Hong Wang(王典洪)
1 School of Mechanical Engineering and Electronic Information,China University of Geosciences,Wuhan 430074,China
2 MOE Key Laboratory of Fundamental Physical Quantities Measurement,School of Physics, Huazhong University of Science and Technology,Wuhan 430074,China
3 Wuhan Juzheng Environmental Scienceamp;Technology Co.,Ltd,Wuhan 430074,China
Determination of the thermal noise limit in test of weak equivalence principle with a rotating torsion pendulum?
Wen-Ze Zhan(占文澤)1,Jie Luo(羅杰)1,?,Cheng-Gang Shao(邵成剛)2,?,Di Zheng(鄭第)3, Wei-Ming Yin(殷蔚明)1,and Dian-Hong Wang(王典洪)1
1 School of Mechanical Engineering and Electronic Information,China University of Geosciences,Wuhan 430074,China
2 MOE Key Laboratory of Fundamental Physical Quantities Measurement,School of Physics, Huazhong University of Science and Technology,Wuhan 430074,China
3 Wuhan Juzheng Environmental Scienceamp;Technology Co.,Ltd,Wuhan 430074,China
Thermal noise is one of the most fundamental limits to the sensitivity in weak equivalence principle test with a rotating torsion pendulum.Velocity damping and internal damping are two of many contributions at the thermal noise,and which one mainly limits the torsion pendulum in low frequency is difficult to be verified by experiment.Based on the conventional method of fast Fourier transform,we propose a developed method to determine the thermal noise limit and then obtain the precise power spectrum density of the pendulum motion signal.The experiment result verifies that the thermal noise is mainly contributed by the internal damping in the fiber in the low frequency torsion pendulum experiment with a high vacuum.Quantitative data analysis shows that the basic noise level in the experiment is about one to two times of the theoretical value of internal damping thermal noise.
weak equivalence principle,torsion pendulum,thermal noise limit,internal damping
1.Introduction
The weak equivalence principle is the foundation of the general theory of relativity,which states that the gravitational mass and the inertial mass of any object is equivalent.[1–5]However,almost every theoretical attempt to unify the gravity to the other three interactions allows for a violation of the weak equivalence principle in some degree.[6,7]Therefore,a high precision measurement of the weak equivalence principle has greatly important scientific significance.
As an extremely sensitive measuring device,the torsion pendulum plays an important role in weak force detection, which has been recognized and applied widely to the experiment of gravitational physics over the last two centuries since Cavendish’s measurement of gravitational constant G.[8]During that span it has played the central role in the laboratory experiments of weak equivalence principle test,Newtonian gravitational constant determination,and the gravitational inverse square law test.[8–10]In the laboratory experiment of weak equivalence principle test,the torsion pendulum method obtained the most precise test result under the 10?13level,[1]which is first introduced by E?tv?s in 1900,[11]and significantly improved by the E?t-Wash group.[1,2,12,13]At present, the limitations of sensitivity and precision in weak equivalence principle test with a torsion pendulum are thermal noise,local gravitational gradients disturbances,and micro-seismic noise, among which thermal noise sets the most fundamental limit to the torsion pendulum of the highest accuracy.[14,15]The influence of gravitational gradients can be analyzed by a corresponding modulation experiment and the influence of micro-seismic disturbance also can be reduced to a minimum with a better experimental platform.But the thermal noise due to internal damping in the suspension system as an intrinsic noise of the torsion pendulum is unavoidable.
Thermal noise originates from the study of Brownian motion of particles,and then Einstein explained this motion as a random fluctuation phenomenon,which is often modeled as the internal damping or the velocity damping in the torsion pendulum experiment.[9,16]As the power spectral density of thermal noise is a constant in the usual experimental condition,the white noise model is used to analyze thermal noise usually.[14,17]Although the thermal fluctuation torque that acts on the system of the torsion pendulum is uncorrelated for two different times,the response of the torsion pendulum is always correlated.[14]In previous works,the thermal noise limit to weak signal detection with the torsion pendulum has been extensively studied by many authors with different methods.[14,15,18–20]These relevant studies have focused mainly on the uncertainty of period measurement of a torsion pendulum due to thermal noise.Besides,with the experimental data obtained,the use of the noise model and the quantitative analysis result of the thermal noise limit in the low frequency torsion pendulum are significant issues which needstudying.In this condition,a quantitative analysis of the thermal noise limit in low frequency or super low frequency signal is well worth studying.
In this paper,based on the conventional fast Fourier transform in the field of signal processing,a developed method is proposed to determine the thermal noise limit.After considering the influence of free oscillation,and the effects of the window and their corrections,a power spectrum density of high precision is obtained by this method,which is much smoother. An experimental data analysis for the test of the weak equivalence principle with a rotating torsion pendulum shows that the basic noise level in the low frequency torsion pendulum experiment is in good agreement with the internal damping model rather than the velocity damping model.A quantitative analysis further shows that the basic noise level in our experiment is one to two times of the theoretical value of the fundamental thermal noise limit.That is to say,it is reasonable to use the internal damping in the process of thermal noise analysis.This result is important for the precision determination of a signal with known frequency and test of weak equivalence principle in high accuracy.
2.Thermal noise limit in test of weak equivalence principle with a rotating torsion pendulum
In a test of the weak equivalence principle with a rotating torsion pendulum,the group of the Huazhong University of Science and Technology(HUST)modulate the possible violative signal to an adjustable-periodic signal,which is similar to the experiment of the E?t-Wash group.In this condition,the signal to be measured can be modulated to the turntable’s rotation frequency,and then the influence of 1/f noise is greatly reduced,[21]but the thermal noise still sets a fundamental limit to the experiment.
2.1.Test of weak equivalence principle with a rotating torsion pendulum
The rotating torsion pendulum method,developed by the E?t-Wash group of the University of Washington,has been commonly used to test the weak equivalence principle.[1,2,13]In this method,the whole system of the torsion pendulum is suspended from an air bearing turntable by a torsion fiber.The turntable provides a stable angular velocity ω,which is about 20 minutes per 360 degrees by the HUST group.As the torsion pendulum is particularly sensitive to the angular deflection about the fiber axis due to the force acting on the test bodies in different directions,in the measurement with a rotating torsion pendulum,we just need to measure the differential horizontal acceleration due to gravity for different test masses of the torsion pendulum.[2]
As shown in Fig.1,assuming that test masses m1and m2are made of two different materials located at r1and r2,the net force on the two test masses are F1and F2,and the fiber direction of the pendulum is opposite to the direction of the sum of the two net forces acting on the test masses.Therefore, the torque about the fiber of the simple torsion pendulum can be expressed as[21]

where n denotes the fiber direction of the pendulum and τ depends on the differential horizontal acceleration of the two forces acting on the test masses.In the experiment,though these two test masses are made of different materials,the quality is exactly the same.Thus the torque about the fiber can be written as
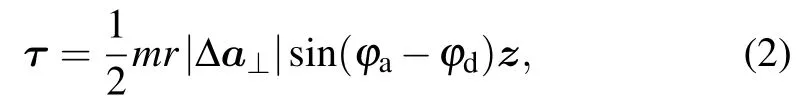
where m denotes test mass m=m1=m2,Δa⊥is the horizontal differential acceleration between the two test masses,?ais the angle of Δa⊥=(a1?a2)⊥,?dis the direction of the composition dipole with respect to the east,and z is the direction along the fiber.
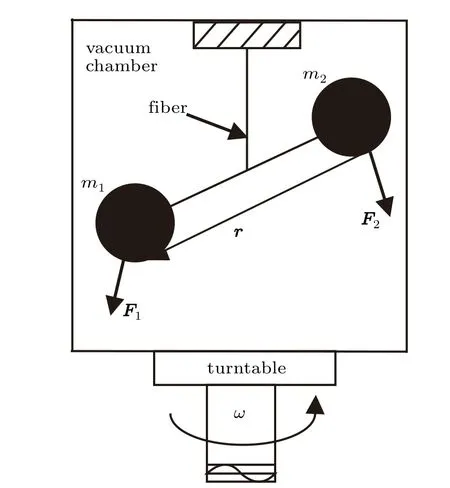
Fig.1.The schematic diagram of the test of weak equivalence principle with a rotating torsion pendulum.
The violation of the weak equivalence principle is usually expressed by the E?tv?s parameter as[1]

where ghis the horizontal acceleration component of the gravitational field g.As the laboratory of this experiment is located in Wuhan,Hubei province,the latitude of which is about 30°and gh≈1.46 cm/s2.Thus,in the experiment which takes the Earth as the gravitational source,it is necessary to measure the differences of the horizontal accelerations for different materials.In fact,no matter what source mass,as long as there is a component in the horizontal of the torsion pendulum,it can be used as a source to test the weak equivalence principle.Both the Sun and the Earth or objects near the Earth’s surface are used as a source usually.When the Sun is used as the source,the horizontal acceleration component of the gravitational field iscm/s2.According to Eqs.(2)and (3),in this experiment it is important to obtain the differences of the horizontal accelerations from the angular deflection of the torsion pendulum θ accurately.Consequently,the weak equivalence principle violations will be tested.
2.2.Thermal noise limit
Based on the basic experimental principles,in the test of the weak equivalence principle with a rotating torsion pendulum,one needs to determine the amplitude of the turntable signal with a very high precision.The precision of the torsion pendulum is inevitably limited by different noises.In the method of the rotating torsion pendulum,by using a continuously rotating torsion pendulum,the experimental signals that we detect will have periodic changes.At the same time,this method also effectively avoids some background noise with a 24 hour period,but thermal noise as a kind of intrinsic noise sets a fundamental limit to the experiment.
In the common analysis of the thermal noise limit,there are two typical widely used models,which are velocity damping and internal damping.The thermal noise spectrum of the twist angle for the torsion pendulum can be expressed as[21]
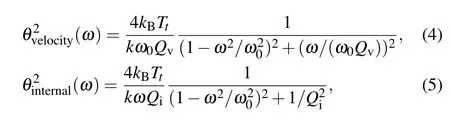
Velocity damping and internal damping are two of many contributions at the thermal noise.Although the two distinct damping mechanisms can both influence the torsion pendulum,a high precision torsion pendulum experiment is most commonly limited by the internal damping in the fiber.[9]That is because most modern experiments with torsion pendulum are operated at pressures below 10?4Pa.At this condition, the mean free path of gas molecules is longer than the typical distances between the pendulum and nearby surfaces,velocity damping becomes unimportant.
For instance,considering a usual experiment by means of the rotating torsion pendulum method,the contribution to the uncertainty of the torque by the thermal noise could be expressed as
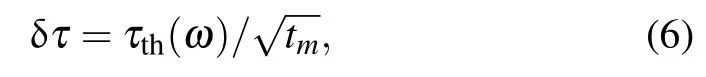
where tmis the total sample time andis the spectral density of the thermal driving force.With typical experiment parameters that the frequency of violative signal is set to be 1/1200 Hz,Tt=300 K,Qt=3000,and tm~3 days,in order to test the weak equivalence principle under the level of 10?12,the uncertainty of the torque by the thermal driving force will be 2.6×10?15Nm/and the corresponding uncertainty of the twist angle for the torsion pendulum is 0.73×10?6rad/
3.Obtaining the thermal noise limit in test of weak equivalence principle with a rotating torsion pendulum
In the study of power spectrum density,the conventional method is fast Fourier transform.Due to the window effect and the phase effect,the precision of the spectrum is limited. Based on the conventional method,a developed method is proposed to estimate the thermal noise limit in the low frequency experiment of the torsion pendulum.In order to facilitate the analysis of this method,the experimental signal{yi(t)}of torsion pendulum is expressed as
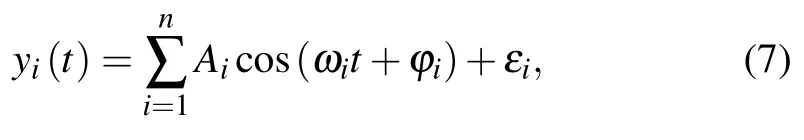
where Ai,ωi,and ?idenote the amplitude,angular frequency, and initial phase of the torsion pendulum signal,respectively; n denotes a positive integer;the parameter εirepresents the noise sequence.
Firstly,according to the data processing rules,[22]the data polluted by sudden external interference should be discarded, such as a sudden earthquake and a bad temperature drift,even when the basic noise level of the torsion pendulum was bad. We usually get rid of the data in the first few days of the data processing,and then select the middle segment to deal with.
Secondly,although the amplitude of the pendulum’s torsion free oscillation is small,to determine the thermal noise limit accurately,one needs to remove the free oscillation signal usually.[23]By moving the raw data point of one period of free oscillation,a new data sequence is obtained.Then we subtract the new sequence from the raw data sequence and get the result sequence
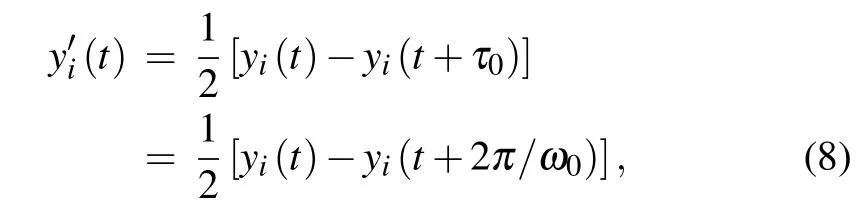
where τ0is the free oscillation period.It is obvious that not only is the free oscillation signal removed,but also the influences of are reduced.At the same time,the amplitudes of the raw signal sequence at different frequencies are slightly attenuated,which should be corrected.At the signal frequency of ω,the equation(8)can be written in the form

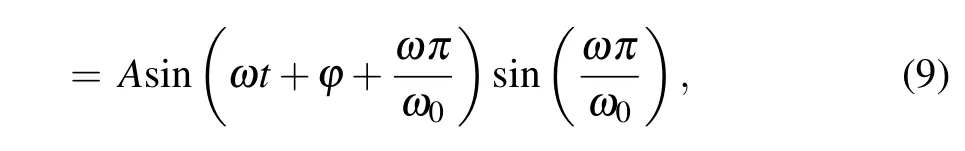
where A and ? denote the amplitude and initial phase of the filter data sequence at angular frequency ω,respectively.According to Eq.(9),it is clear that the amplitude is attenuated by the factor sin(ωπ/ω0),and the attenuation is related to the frequency.With this attenuation,the amplitude can correct to the true amplitude in the result.
Thirdly,since the total sampling time in our experiment is about 3 days,considering the influence of experimental environment fluctuation,the method of segmentation is usually used.In this process,the whole data sequence is divided into several segments and then processed separately.To avoid the effect of harmonic terms,the segments usually start at the initial phase which is 0 degrees,or its integral times.For each period,according to the orthogonality of the trigonometric function,the amplitudes of the components ajand bjare calculated from
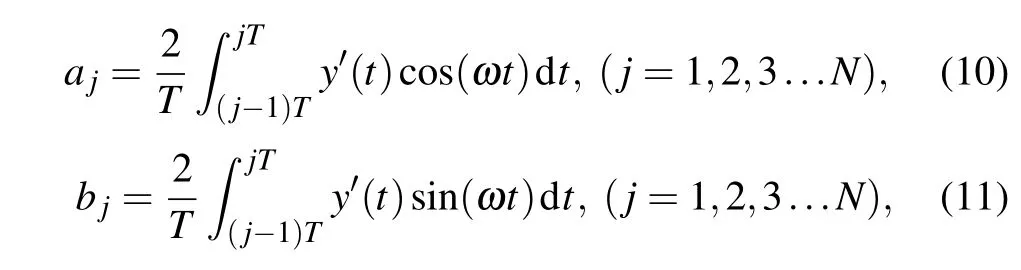
where T is the period of the segment and N represents the number of the period.For number computation,the integral is usually replaced by the sum.As a matter of fact,this requires that the data points of each segment are sufficient.In this condition,to obtain the result with higher precision,we add a window function to the data points sequence in each segment,and then the equations(10)and(11)can be further expressed as
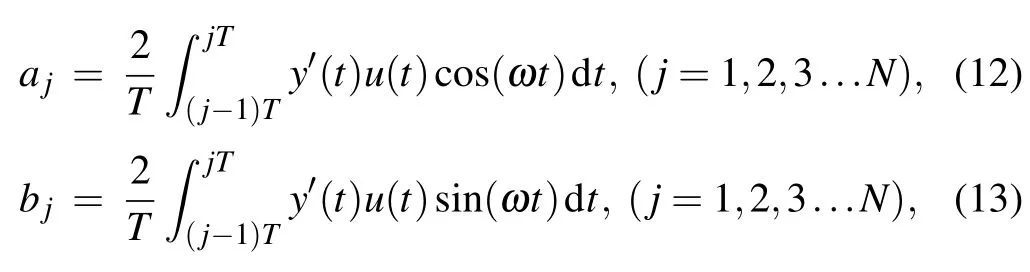
where u(t)denotes the window function.In this way,there is another attenuation factor introduced by the process of adding the window function,which should also be considered.Here we use the Hanning window function as an example,which is commonly used in spectral analysis.For convenience in calculation,at the frequency of ω,the amplitude can be set as a constant 1 before this processing,and then the Hanning window function in time domain can be expressed as
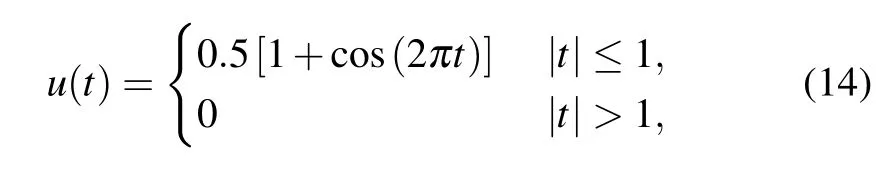
where u(t)is the normalized result of the length of the window.With the process of Fourier transform,the expression of the window function in the frequency domain is
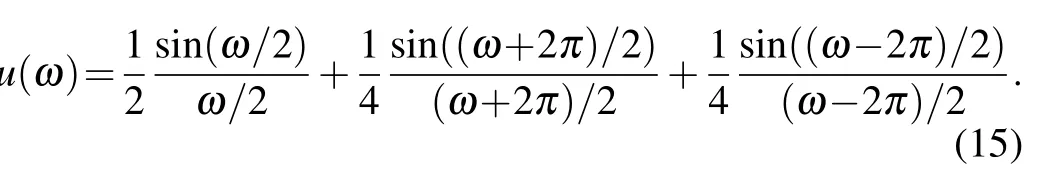
Considering that the amplitude of the signal should be unchanged after processing,the attenuation factor is equal to the ratio of the signal amplitudes before and after the process of adding the Hanning window function,and thus the attenuation factor can be obtained as
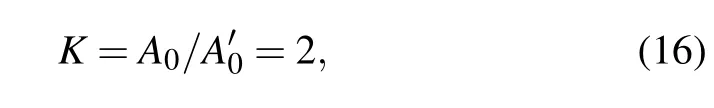
where A0denotes the amplitude of the signal before processing with the window function,denotes the signal amplitude of the peak after processing,and the value can be obtained by the L’Hospital rule of Eq.(15).
Based on the above data processing,the amplitude sequence{aj}and{bj}at the jthperiod are obtained,and then we can obtain the central values and uncertainties of the two coefficients a and b in the method of weighting all coefficients ajand bjequally.Therefore,the amplitude A of the experimental signal and its uncertainty ΔA are finally obtained.Here we consider that the uncertainty ΔA is caused by the basic noise in our experiment,as shown in Eq.(7).The fundamental noise sources that exist in our torsion pendulum experiments are usually complex,such as thermal noise,shot noise,and even environment noise.In addition,the influence of intrinsic noise mainly comes from thermal noise as one of the most common intrinsic noises,especially in low frequency.In this condition,it is obvious that we can determine the basic noise level accurately by this method,and then can choose the suitable model to assess the influence of thermal noise.What is more,from the numerical simulation results,we have shown the feasibility of this method and found that our method is better than the conventional method to obtain the power spectrum density.
4.Experimental results
For atypical data set of the experiment of testing the weak equivalence principle with a rotating torsion pendulum by the HUST group,it has been validly recorded for about 8 days with free oscillation signal and the interested frequency of the violative signal is set to be 1/1200 Hz.The free oscillation frequency of the torsion pendulum is about 1.27 mHz.Figure 2 shows the time domain figure of the rotational angle.In Fig.2,the top panel is the initial signal,the interval of the sample time is 1 s,and the bottom panel is the signal of the rotational angle after removing the free oscillation signal.It is obvious that the influence of the monotonic drift is limited.
Figure 3 is the power spectrum density of the signal shown in the top panel of Fig.2,which is obtained by the conventional fast Fourier transform method.There is a peak at the frequency of 1.27 mHz,which is the free oscillation frequency.There is another peak around 0.833 mHz,which is the rotating frequency of the turntable.It can be found that higher harmonics are the primary noise at the frequency that is greater than the free oscillation frequency.
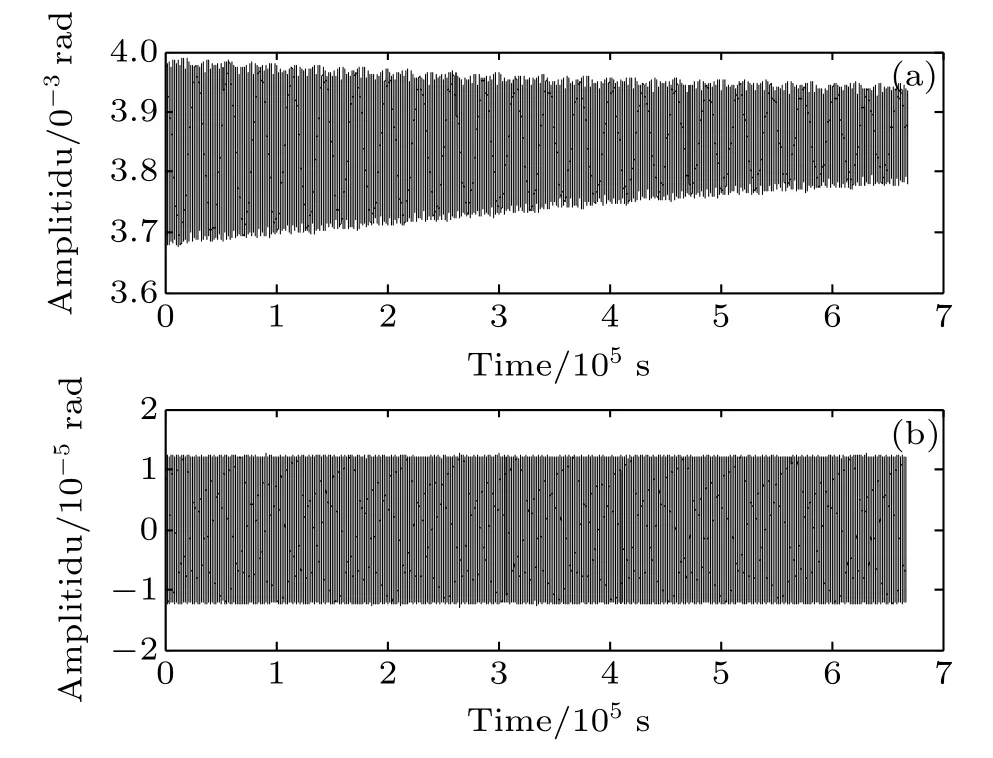
Fig.2.The time domain figure of the rotational angle.(a)The initial signal and(b)the signal after removing the free torsion signal.

Fig.3.The spectrum of the amplitude of the signal shown in the top panel of Fig.2,which is obtained by the conventional method of fast Fourier transform.
Figure 4 is the power spectrum density of the signal shown in the bottom panel of Fig.2,but this result is obtained by the proposed method in this paper.The bottom two lines represent the thermal noise limit of the velocity damping and the internal damping,respectively,which are obtained from Eqs.(4)and(5).
When ω<ω0,internal damping is the main contribution at the thermal noise.When ω>ω0,velocity damping is the main contribution at the thermal noise.When ω=ω0,the internal damping and the velocity damping have the same values,where the quality factors of the two models are assumed to be equal.By comparing Figs.3 and 4,it is obvious that the spectrum of the signal shown in Fig.4 is much smoother in the low frequencies,especially in the frequencies smaller than the free oscillation frequency.At the same time,the basic noise level in the low frequency torsion pendulum experiment is in good agreement with the internal damping thermal noise in the low frequencies.As shown in Table 1,by further quantitative analysis,we found that the difference between the basic noise level in our experiment and the internal damping thermal noise is about 1 to 2 times in low frequency,which may be caused by the microseismic noise or small fluctuations in temperature.The basic noise level in high frequency is not in good agreement with the internal damping thermal noise,mainly due to the influence of the shot noise of the autocollimation system.In addition,we have tested the result by adding a calibration signal in this experimental data,which is also shown in Table 1,and the results are exactly the same.Thus,the experiment result verifies that the thermal noise is mainly contributed by the internal damping in the low frequency torsion pendulum experiment with high vacuum,and it is reasonable to use the model of internal damping for the analysis of the influence caused by thermal noise.
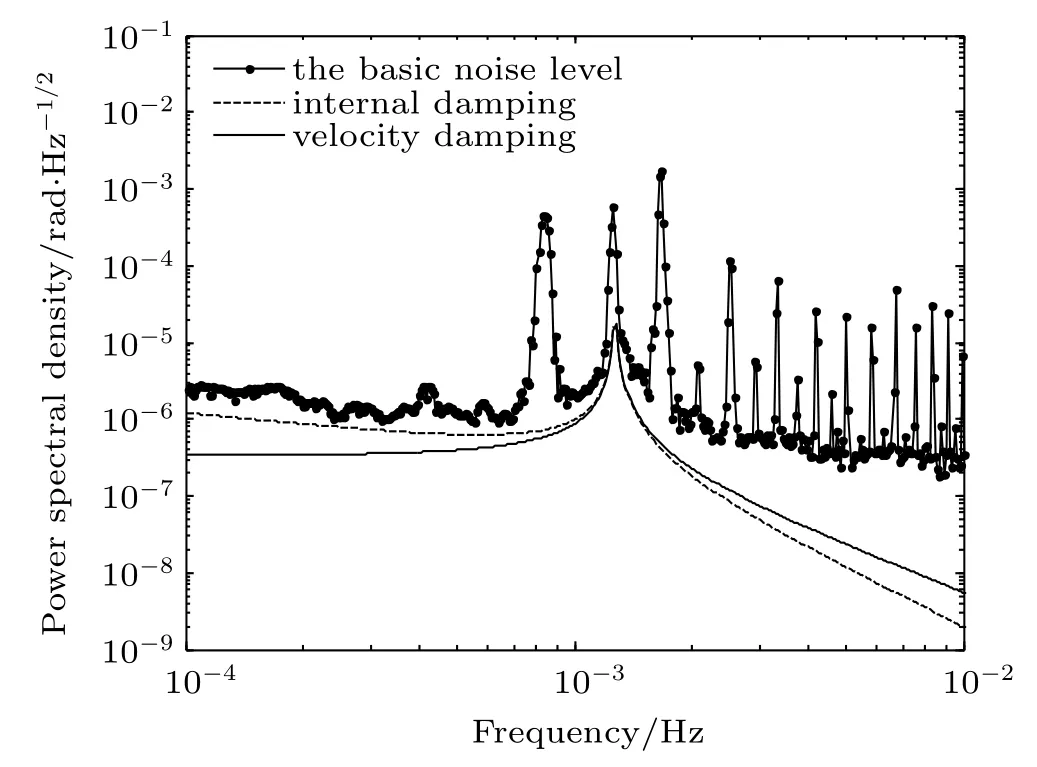
Fig.4.The spectrum of the amplitude of the signal shown in the bottom panel of Fig.2,which is obtained by the proposed method in this paper.
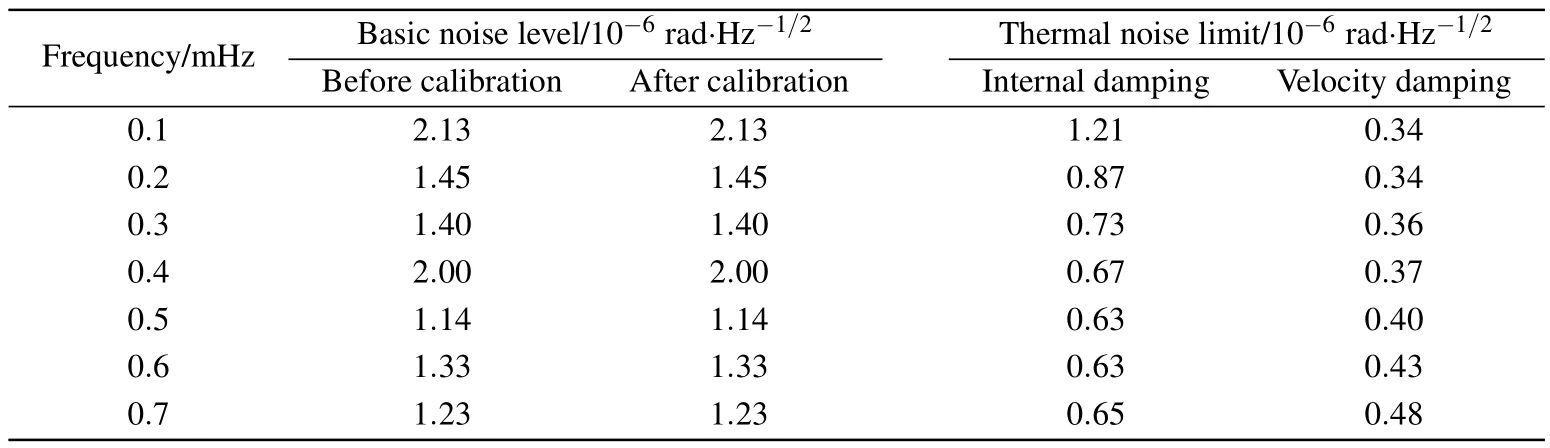
Table 1.The basic noise level in our experiment and the thermal noise limit.
5.Conclusion
Due to the influence of thermal noise,there exists a fundamental limit to the sensitivity and precision of the torsion pendulum.It is a significant system error in the test of weak equivalence principle with a rotating torsion pendulum,the influence of which is unavoidable.Besides,the velocity damping and the internal damping are two of many contributions at the thermal noise and it is difficult to verify which sets a dominative limit on the precision of the torsion pendulum in low frequency.In this paper,we obtained the power spectrum density of the basic noise level by the developed method.Different from the conventional spectrum analysis method of fast Fourier transform,this method can acquire a smoother power spectrum density.The result of processing experimental data shows that the basic noise level in the torsion pendulum experiment of low frequency is in good agreement with the internal damping thermal noise,which is about 1 to 2 times of the latter.It directly verifies that the high precision torsion pendulum experiment is mainly limited by the internal damping in the fiber and the internal damping model is reasonable to express the thermal noise limit in the low frequency torsion pendulum experiments with high vacuum,which are instructive and important to the test of the weak equivalence principle with a rotating torsion pendulum.At present,since many kinds of noises are not fully understood,there are still many works to do in this field.
Acknowledgment
The authors are heartily grateful to the Center for Gravitational Experiments of HUST for providing the experimental data.
[1]Schlamminger S,Choi K Y,Wagner T A,Gundlach J H and Adelberger E G 2008 Phys.Rev.Lett.100 041101
[2]Su Y,Heckel B R,Adelberger E G,Gundlach J H,Harris M,Smith G L,and Swanson H E 1994 Phys.Rev.D 50 3614
[3]Zhou Z B,Luo J,Dan Q,Wu Z G,Zhang Y Z and Nie Y X 2002 Phys. Rev.D 66 022002
[4]Luo J,Nie Y X,Zhang Y Z and Zhou Z B 2001 Phys.Rev.D 65 042005
[5]Zhu L,Liu Q,Zhao H H,Feng W F,Yang S Q,Shao C G,Tu L C and Luo J 2016 Sci.Sin.-Phys.Mech.Astron.46 073002(in Chinese)
[6]Wang J 2015 Chin.Phys.B 24 053702
[7]Damour T 1996 Class.Quantum Grav.13 A33
[8]Cavendish H 1798 Philos.Trans.R.Soc.Lond.17 469
[9]Adelberger E G,Gundlach J H,Heckel B R,Hoedl S and Schlamminger S 2009 Prog.Part.Nucl.Phys.62 102
[10]Feng G Q,Yang S Q,Tu L C and Luo J 2006 Chin.Phys.Lett.23 2052
[11]E?tv?s R V,Pekar D,and Feteke E 1922 Ann.Phys.68 11
[12]Adelberger E G,Stubbs C W,Heckel B R,Su Y,Swanson H E,Smith G L,Gundlach J H and Rogers W F 1990 Phys.Rev.D 42 3267
[13]Smith G L,Hoyle C D,Gundlach J H,Adelberger E G,Heckel B R and Swanson H E 1999 Phys.Rev.D 61 022001
[14]Chen Y T and Cook A 1990 Class.Quantum Grav.7 1225
[15]Ritter R C,Winker L I and Gillies G T 1990 Meas.Sci.Technol.10 499
[16]An X T,Li Y X and Liu J J 2007 Acta Phys.Sin.56 4105(in Chinese)
[17]Lamoreaux S K and Buttler W T 2005 Phys.Rev.E 71 036109
[18]Peter R S 1990 Phys.Rev.D 42 8
[19]Luo J,Shao C G,and Wang D H 2009 Class.Quantum.Grav.26 195005
[20]Luo J,Shao C G,Tian Y and Wang D H 2013 Phys.Lett.A 377 1397
[21]Wagner T A,Schlamminger S,Gundlach J H and Adelberger E G 2012 Class.Quantum Grav.29 18
[22]Tu L C,Li Q,Wang Q L,Shao C G,Yang S Q,Liu L X,Liu Q and Luo J 2010 Phys.Rev.D 82 022001
[23]Wu W H,Tian Y,Xue C,Luo J and Shao C G 2017 Chin.Phys.B 26 040401
5 April 2017;revised manuscript
18 May 2017;published online 31 July 2017)
10.1088/1674-1056/26/9/090401
?Project supported by the National Natural Science Foundation of China(Grant Nos.11575160 and 11275075)and the Natural Science Foundation of Key Projects of Hubei Province,China(Grant No.2013CFA045).
?Corresponding author.E-mail:luojiethanks@126.com
?Corresponding author.E-mail:cgshao@mail.hust.edu.cn
?2017 Chinese Physical Society and IOP Publishing Ltd http://iopscience.iop.org/cpb http://cpb.iphy.ac.cn
- Chinese Physics B的其它文章
- Improved control for distributed parameter systems with time-dependent spatial domains utilizing mobile sensor actuator networks?
- Geometry and thermodynamics of smeared Reissner–Nordstr?m black holes in d-dimensional AdS spacetime
- Stochastic responses of tumor immune system with periodic treatment?
- Invariants-based shortcuts for fast generating Greenberger-Horne-Zeilinger state among three superconducting qubits?
- Cancelable remote quantum fingerprint templates protection scheme?
- A high-fidelity memory scheme for quantum data buses?

