Rumor Spreading Model with Immunization Strategy and Delay Time on Homogeneous Networks?
Jing Wang(王靜)Ya-Qi Wang(王亞奇)? and Ming Li(李敏)
1Xi’an Research Institute of Hi-Tech,Xi’an 710025,China
2Department of Electronics Technology,Engineering University of the Chinese People’s Armed Police Force,Xi’an 710086,China
1 Introduction
Rumors have a long history,usually referring to the fabricated opinion on public affairs.[1?2]Traditional rumors spread from mouth to mouth.In recent years,with the rapid development of various social networks,rumors can quickly propagate by means of social networks,[3?4]which usually be called Internet rumors.Traditional rumors,especially Internet rumors,not only interfere with people’s daily lives,but even endanger the stability of society.[5?7]Therefore,it is of great theoretical signi ficance to explore the inherent propagation mechanism of rumors,and this has already aroused a widespread concern of many researchers both at home and abroad.
As early as the 1940s,sociologists began to study the spread of rumors.However,the modeling of rumor propagation began in 1965,when Daley and Kendall[8]introduced the first rumor spreading model,which is called the DK model.In the DK model,the population was divided into three categories:people who do not contact the rumor,people who have accepted the rumor and spread it,and people who have accepted the rumor but do not propagate it again.The classical DK model laid a foundation for the following research.The effect of the topological properties of networks on the spread of rumors was investigated by Sudbury.[9]Subsequently,with the development of complex network propagation theory,Zanette[10]applied the theory to construct a rumor-spread model,and discussed the propagation characteristics of the model on small-world networks.Based on the susceptible-infectedremoved(SIR)model used to describe the spreading process of epidemics,[11?13]Morenoet al.[14]built an SIR rumor propagation model,but where“S” represents“ignorants” who have no idea about the rumor,“I” stands for“spreaders” who contact the rumor and spread it,and“R”represents “sti flers” who contact the rumor but do not propagate it.According to this SIR model,researches proposed many similar rumor-spread models.One of the most widely used SIR rumor model was presented by Nekoveeet al.[15]They considered the forgetting during the spreading process of rumors as an important mechanism,and constructed the mean- field equations in both homogeneous networks and heterogeneous networks.The critical thresholds and the final degree of rumor spreading on the two different types of networks were obtained.For more research on rumors,including Internet rumors,refer to Refs.[16–23].
In view of the spread of rumors can cause a lot of harm,and drawing experience from the immunization strategies for the prevention of epidemic transmission,[24]it has important realistic meaning to adopt immunization strategies to control rumor spreading,which has attracted attention of the relevant researchers.Huanget al.[25]ap-plied two major immunization strategies on the smallworld network.They found that the size of the average degree of the network determined the immune effect of the two immunization strategies.In addition,they also proposed a strategy to prevent the spread or rumors.In order to deal with rumor propagating on the mobile social networks,two kinds of methods have been designed,i.e.,blocking in fluential nodes to spread rumors and clarifying rumors by spreading truth.[26]Incorporating the two kinds of methods,Heet al.[27]presented two cost-efficient strategies to restrain rumor spreading on the mobile social networks.The efficiency of the two strategies had been extensively evaluated.Zhaoet al.[28]introduced the immunization strategy into the rumor spreading on the homogeneous networks.They obtained an immunization threshold value,above which rumor spreading can be stopped.They also found that the average degree of the network greatly in fluences the spread of rumors.In this paper,the immunization strategies all refer to make people know the truth of rumors.
In the above models,the immunization strategies are assumed to be implemented just when there are rumors spreading on the networks.In fact,the spread of rumors is always ahead of the implementation of immunization strategies,i.e.,there must be a delay time between them.How will the delay time together with the spread of the truth affect the immune effect? It is clear that the research of this problem will enable us to grasp more realistic dynamical behaviors of rumor spreading on the networks that are implemented immunization strategies,so that we can take measures to improve the immune effect of the corresponding immunization strategies.However,to the best of our knowledge,up to now,the above problem has not yet been explored.In order to efficiently prevent and control rumor propagation,considering the in fluence of the delay time before implementing immunization strategy and the spread of the truth of rumors,this paper proposes a novel SIR rumor model.The propagation characteristics of the model are fully examined by the detailed theory analysis and the sufficient numerical simulations.As will be shown in Sec.4,the delay time and the truth propagation have a great in fluence on the propagation dynamical behaviors of rumors.
2 SIR Rumor Spreading Model
Consistent with the previous research,[14]in our SIR rumor spreading model,the total population consisting ofNindividuals are also divided into ignorants(denoted asS),spreaders(denoted as I)and sti flers(denoted asR),which with respect to the epidemic correspond to susceptible individuals,infected individuals and removed individuals,respectively.In this paper,it is assumed that the population are closed and mixed,and can be described by using the homogeneous networks.In order to control the rumor spreading,the random immunization strategy that randomly chooses a part of ignorants and makes them know the truth of rumors is adopted.Considering the existence of delay time,thefpercent of ignorants in the entire network are randomly chosen to implement immunization afterTtime steps of rumor spreading.Different from the immune mechanism in the spread of epidemics,the immunized nodes here not only oppose the spread of rumors,but also can propagate the truth of rumors to spreaders.Of course,these immunized nodes will not accept rumors and thus can be classi fied as sti flers.So,stiflers in this paper consist of two different types of nodes,one is that who has accepted the rumor but has ceased to spread it(denoted asR1 sti flers),and the other is that who has known the truth of rumors and will propagate it to spreaders,i.e.,the immunized nodes(denoted asR2 sti flers).In the following process of rumor spreading,R1 sti flers will always remain silent state,and are no longer affected by rumors or the truth.So,there is no interaction betweenR1 sti flers andR2 sti flers.In addition,the mechanism that when contacting anR1 sti fler,a spreader will become anR1 sti fler is ignored.This is because thatR1 sti flers do not spread rumors and are likely to just keep silence,which cannot make a spreader into anR1 sti fler.Rumors spread among different nodes through direct contacts.The spreading process of our SIR rumor model is given as Fig.1.
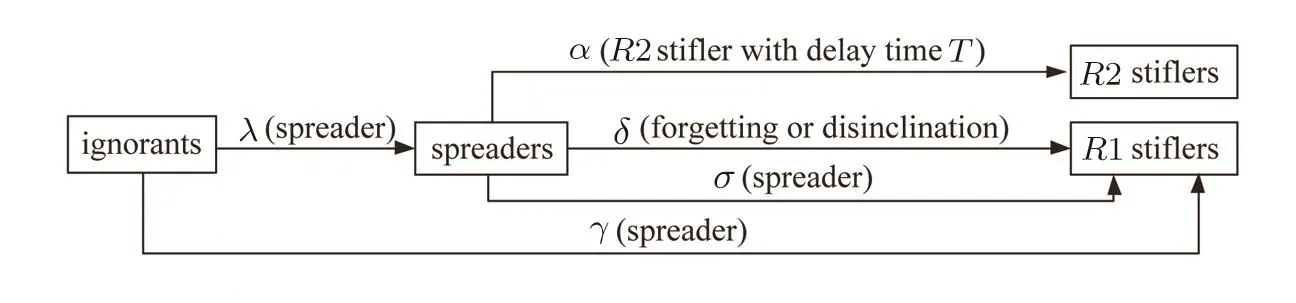
Fig.1 The rumor propagation process of our SIR model.
Combining with Fig.1,the transmission laws of our SIR model are as follows:
(i)When an ignorant contacts a spreader,the ignorant will become a spreader with probabilityλ(called the effective propagation rate),or anR1 sti fler at a rateγdue to he/she owning a strong identification force.
(ii)When a spreader contacts anR2 sti fler from theT+1 time step,the spreader will become anR2 sti fler with the probabilityα(called the reliability of the truth).
(iii)If a spreader contacts another spreader,the initiating one will become anR1 sti fler with probabilityσ.
(iv)Due to forgetting or disinclination to spread the rumor,a spreader will become anR1 sti fler with probabilityδ.
From the above description,it can be seen that in our SIR model,both the delay time before implementing immunization to ignorants andR2 sti flers spreading the truth of rumors to spreaders are taken into consideration.Obviously,our SIR model that is great different from the original models is more realistic.
3 SIR Model Analysis
For the homogeneous network,each node degree is approximately equal to the average degree of the network〈k〉.LetS(t),I(t),R1(t)andR2(t)denote the densities of ignorants,spreaders,R1 sti flers andR2 sti flers at timet,respectively.Obviously,these four variables meet the normalization conditionS(t)+I(t)+R1(t)+R2(t)=1.Then,according to the propagation rules of the proposed SIR model,we establish the mean- field equations as follows:
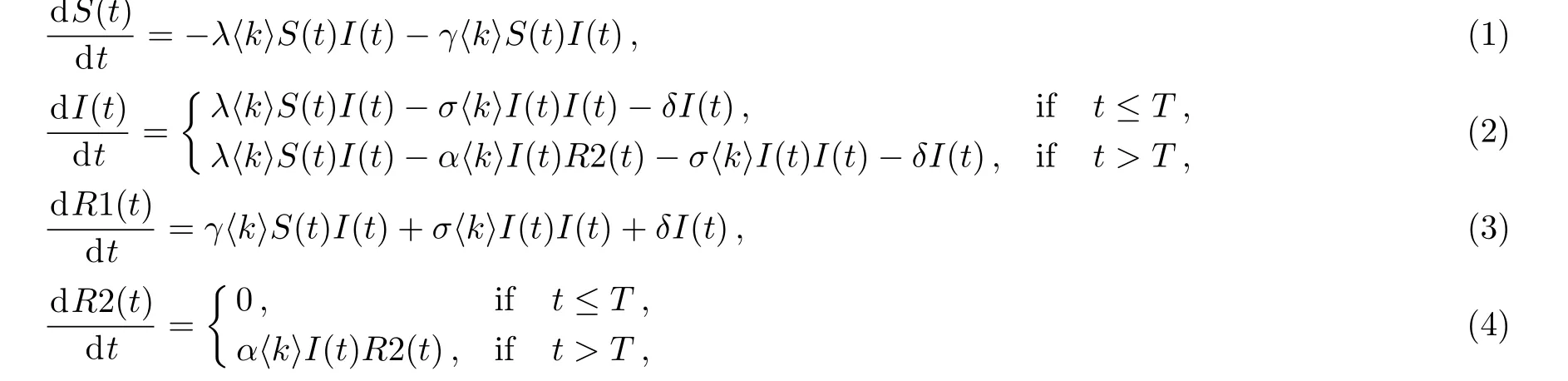
When a rumor begins spreading on the networks,it is assumed that there is only one node has accepted the rumor.So we can obtain the initial conditions for the above four variables as follows
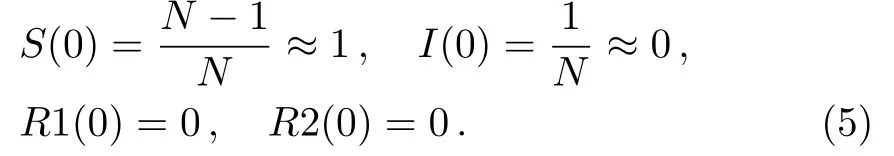
Since the existence of delay time,for the propagation timet<T,R2(t)=0.However,whent=T,the initial value ofR2(T)can be get thatR2(T)=f,and at this timeI(T)?f→I(T).
Considering the initial conditions as in Eq.(5),we can derive the density of ignorants from Eq.(1)as
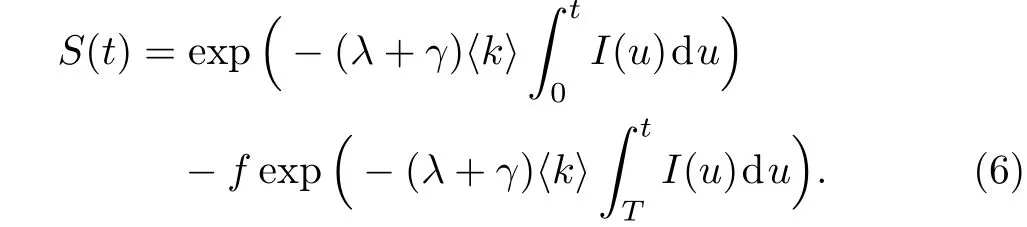
De fining an auxiliary function?(t)that is used to represent the value of integration in the exponent of the first term on the right-hand side in Eq.(6)
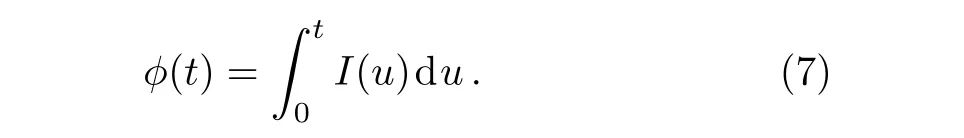
Substituting Eq.(7)back into Eq.(6)gives

When the value ofI(t)is smaller,its higher-order term can be neglected.Then,we can derive the density ofR1 sti flers from Eq.(3)as
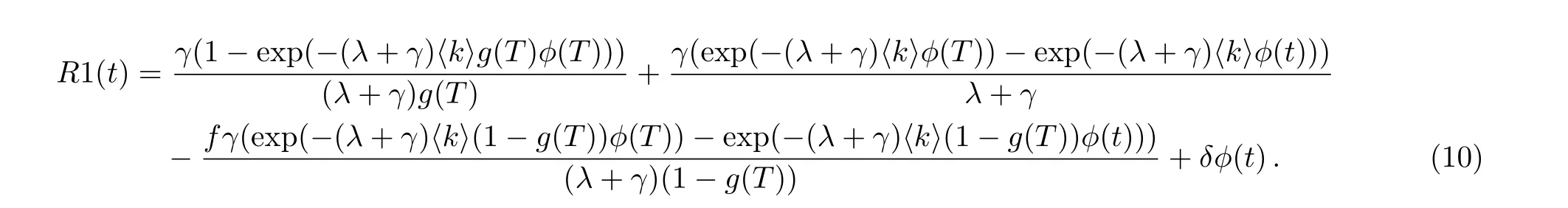
Imposing the initial conditionR2(T)=f,we can solve the differential equation Eq.(4)to obtain that

By differentiation of?(t),a self-consistent equation about?(t)can be expressed as
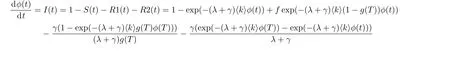

At the steady state of rumor spreading wheret→∞,there are no spreaders left in the networks,i.e.,I(∞)=0.So,we have that

Assume that the steady state value of?(t)is?∞,and then we can derive the expression of?∞from Eqs.(12)and(13)that

whereF(?∞)is an auxiliary function.Clearly,?∞=0 is a solution of Eq.(14),which corresponds to the situation where there is no rumor spreading outbreak.In order to have a rumor outbreak,?∞should have a non-zero solution satisfying

Eq.(15)de fines the critical thresholdλcas

In Eq.(16),λcobviously increases monotonically withf,and decreases monotonically withg(T).This means that the implementation of random immunization strategy can effectively depress the spread of rumors,but the existence of delay time reduces the immune effect of the immunization strategy.Equation(16)also shows that in the present model,nodes’identification force has no effect on the critical threshold.Considering thatf=α=γ=0,thenλc=1/〈k〉,which is consistent with the classical SIR rumor model.[15]
When rumor spreading reaches a steady state,We de-
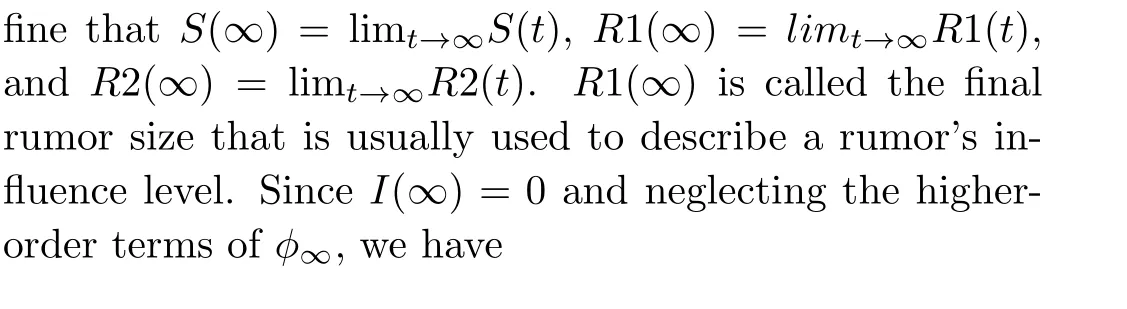
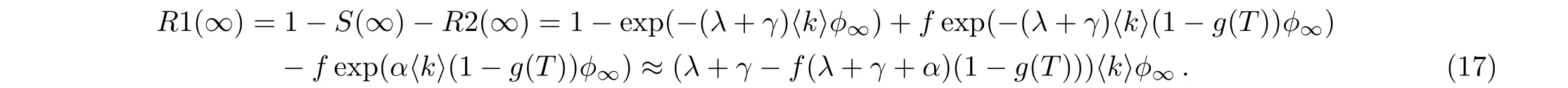
Whenλ→λc,it is reasonable to neglect the higher-order-term of?∞in Eq.(14).Applying the Maclaurin formula to Eq.(14),we have

By Eqs.(18)and(19),one can get

From Eq.(19),we can easily find that,R1(∞)∝f?1andR1(∞)∝g(T).Thus,it can be concluded that both the random immunization strategy and the delay time can bring an important in fluence on the rumor spreading.
4 Simulations Analysis
In this section,the propagation dynamical properties of the novel SIR rumor model proposed in this paper are investigated by using sufficient numerical simulations.The Monte Carlo method is adopted to describe the spreading process of rumors.As a more popular homogeneous network,the WS small-world model[29]is used to depict the population.AfterTtime step,thefpercent of nodes that have not contacted rumors are randomly selected as the immunized nodes.The network size isN=5000,the node average degree is〈k〉=6,and the rewiring probability isp=0.1;moreover,δ=1,σ=1,andγ=1.At the initial moment of rumor spreading,a single node is randomly selected as the propagation source.All the results are obtained by averaging each simulation at least 100 runs with different initial spreaders.
Figure 2 describes the variation of the critical threshold withλcthe immunization proportionf.From Fig.2,one can see that due to the in fluence of delay time,whenf=0,λc<1〈k〉of the classical SIR model on the homogeneous networks.[15]Figure 2 also shows that as the value offincreases,λcobviously increases.Figure 3 illustrates the variation of the value ofR1(∞)with the effective propagation rate.From this figure,we can see that when the rumor propagation comes to a steady state,the number of nodes that finally accept the rumor sharply decreases with the value offincreases.Thus,combining Figs.2 and 3,it can be inferred that even if considering the existence of the delay time,the random immunization strategy is still effective for preventing rumor spreading on the homogeneous networks,which also proves the correctness of the theory analysis in Sec.3.
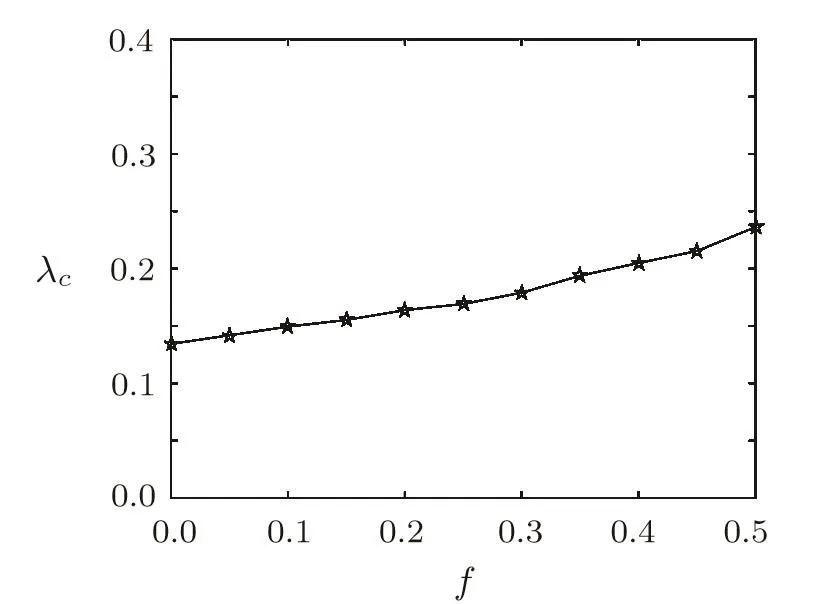
Fig.2 The relationship between the critical threshold λcand the immunization percentage f.The parameters are T=1 and α=0.1.

Fig.3 The relationship between the steady state value of R1 sti flers R1(∞)and the effective propagation rate λ when the value of f varies.The parameters are T=2 and α=0.1.
Figure 4 depicts the critical thresholdλcvaries with the delay timeT.From Fig.4,we find thatλcdecreases slowly with the increase ofT,which is consistent with the theory analysis in Sec.3.Compared with the larger value ofT,the smaller value ofTleads to more obviously decrease ofλc.Figure 5 shows the steady state value ofR1 sti flersR1(∞)depends on the delay timeT.From this figure one can see thatR1(∞)clearly increases as the value ofTincreases.From Figs.4 and 5,it can be followed that the sooner the immunization measure is implemented,the better the immune effect will be.Therefore,whether we can find rumors spreading on the network as soon as possible is essential for the control of rumor propagation.
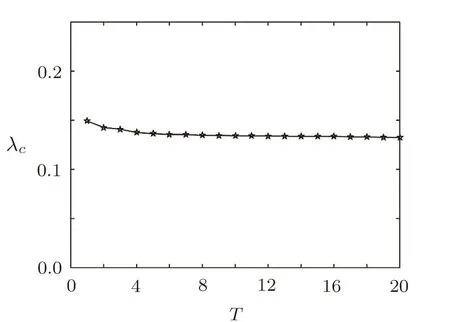
Fig.4 The relationship between the critical threshold λcand the delay time T.The parameters are f=0.05 and α=0.5.

Fig.5 The relationship between the steady state value of R1 sti flers R1(∞)and the the effective propagation rate λ when the value of T varies.The parameters are f=0.02 and α=0.1.
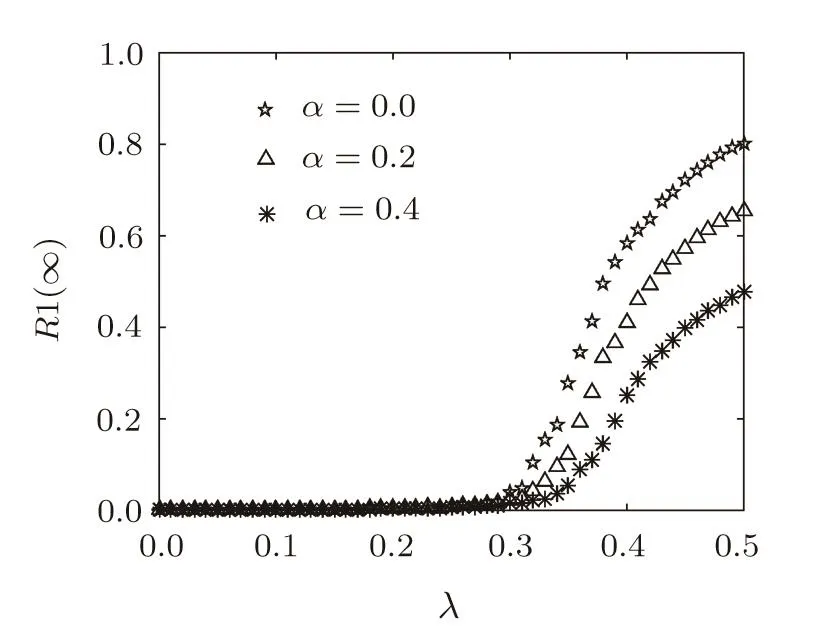
Fig.6 The relationship between the steady state value of R1 sti flers R1(∞)and the effective propagation rate λ when the value of α varies.The parameters are T=3 and f=0.1.
Figures 6 and 7 depict the relationship between the values ofR1(∞),R2(∞)and the critical thresholdλcwith the value ofαvarying.From these two figures,we can find that for a given value ofλ,as the value ofαincreases,the value ofR1(∞)evidently decreases,but the value ofR1(∞)distinctly increases.This implies that the stronger the reliability of the truth of rumors,the more spreaders becomeR2 sti flers,which accordingly reduces the number of nodes that finally accept the rumor.Thus,in order to better control the rumor spreading,the truth of rumors should be published by the more authoritative department,which helps to enhance the reliability of the truth of rumors accordingly.
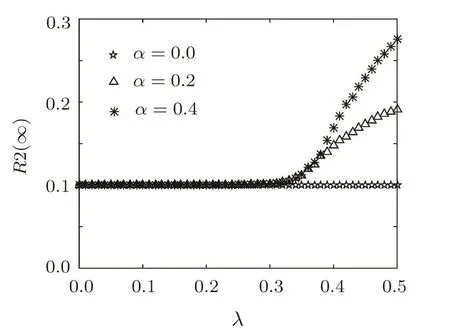
Fig.7 The relationship between the steady state value of R2 sti flers R2(∞)and the effective propagation rate λ when the value of α varies.The parameters are T=3 and f=0.1.
Figure 8 shows the relationship between the steady state value ofR1 sti flersR1(∞)and the parameterγ.From this figure we know that,for the given value ofλ,the value ofR1(∞)decreases slowly as the value ofγincreases.This is because that the stronger the identification force of ignorants,the less the number of spreaders,so that the value ofR1(∞)is decreased.Therefore,the propagation degree of rumors can be alleviated by enhancing the identification force of ignorants.Moreover,agreeably to theoretical analysis in Sec.3,we also find from the figure that the parameterγdoes not change the critical thresholdλc.
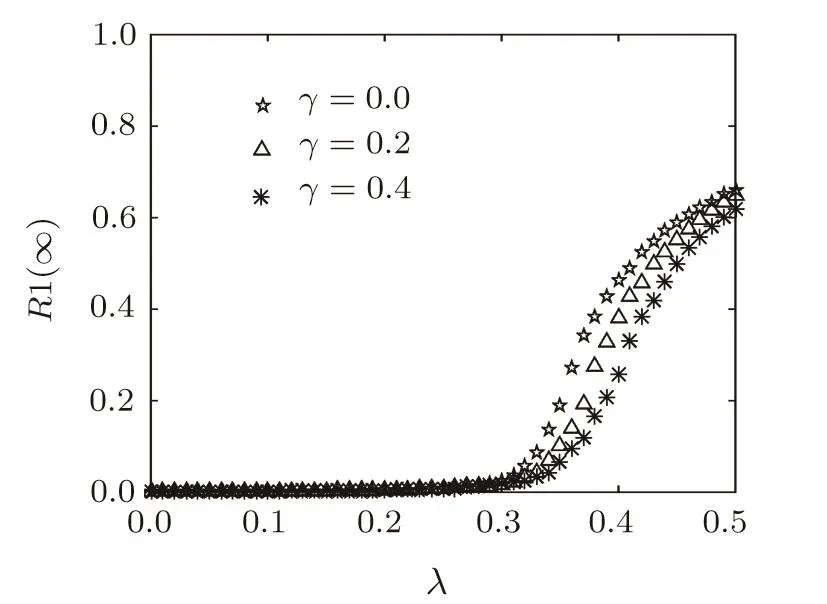
Fig.8 The relationship between the steady state value of R1 sti flers R1(∞)and the effective propagation rate λ when the value of γ varies.The parameters are T=3 and f=0.1.
Figure 9 gives the relationship between the density ofR1 sti flersR1(t)and the spreading timet.It has been seen that as the timetincreases,R1(t)increases exponentially.After some time the rumor spreading comes to a steady state which will remain constant,since there are not any more spreaders in the network at that time.It has also been observed that the larger the value off,the slower the spread of the rumor,and the less the number of nodes that accept the rumor.The density of spreadersI(t)is plotted against the propagation timetin Fig.10.It has observed thatI(t)increases exponentially with the timet,but after achieving a maximum it decays exponentially.Figure 10 also shows that as the value offincreases,the maximum ofI(t)decreases,but the time of rumor spreading is prolonged,which is conductive to taking measures to prevent and control the spread of rumors.

Fig.9 The relationship between the density of R1 stiflers R1(t)and the time t when the value of f varies.The parameters are T=5 and α=0.1.
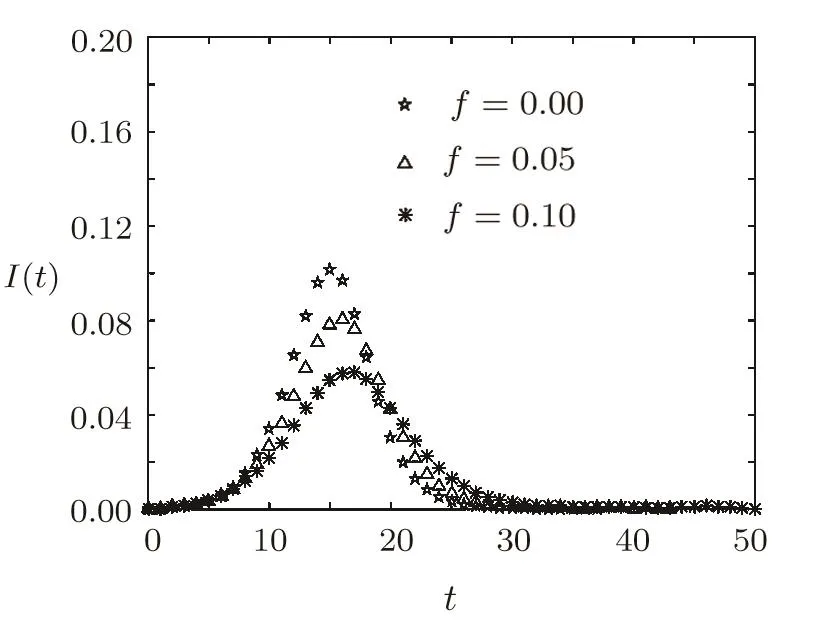
Fig.10 The relationship between the density of ignorants I(t)and the time t when the value of f varies.The parameters are T=5 and α=0.1.
In Fig.11,the density ofR1 sti flersR1(t)has been plotted against the transmission timet.One can clearly observe that when the value oftis small(e.g.,t<10),the values ofR1(t)are almost the same for different values ofα.However,when the value oftis large,R1(t)apparently decreases with the increase ofα.The main reason is that as the reliability of the truth increases,more and more spreaders becomeR2 sti flers,as a result abating the propagation degree of rumors.

Fig.11 The relationship between the density of R1 stiflers R1(t)and the time t when the value of α varies.The parameters are T=6 and f=0.05.
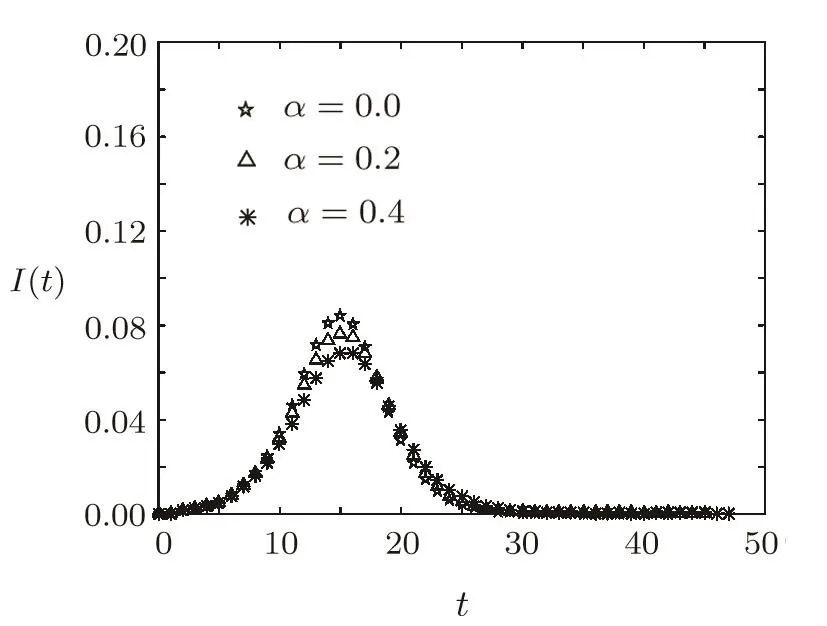
Fig.12 The relationship between the density of ignorants I(t)and the time t when the value of α varies.The parameters are T=6 and f=0.05.
Figures 12 and 13 illustrate the general trends for the density of spreadersI(t)over the spreading timetwith the values ofαandTvarying,respectively.Figure 12 shows that in the process of rumor spreading,as the value ofαincreases,the maximum number of spreaders slightly decreases.That is the propagation of the truth making part of spreaders intoR2 sti flers.Similar to the immune mechanism,the spread of the truth can also inhibit to some extent the spread of rumors.Figure 13 shows that the larger the value ofT,the greater the number of spreaders,and the longer the spread of rumors.Therefore,in order to better prevent and control the spread of rumors,we should not only enhance the reliability of the truth as much as possible,but also immunize the network as early as possible.
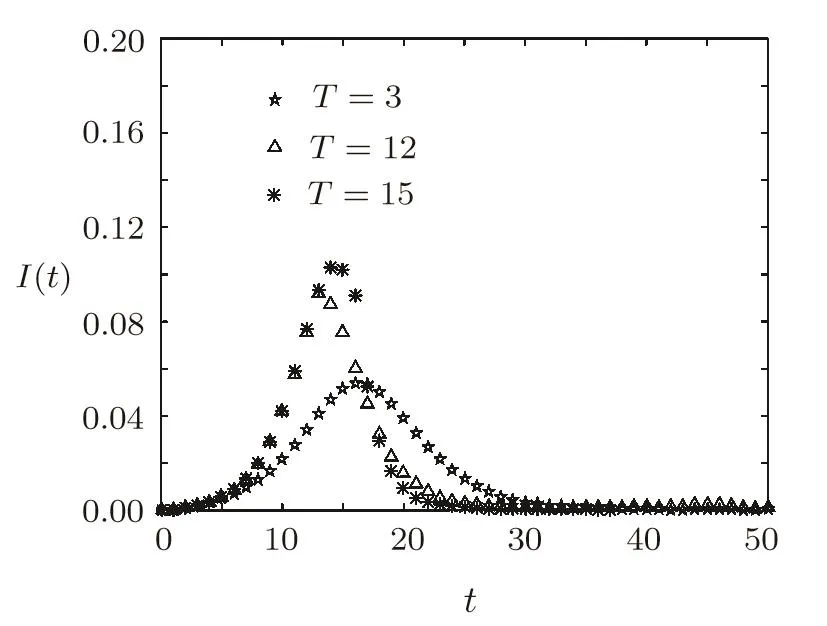
Fig.13 The relationship between the density of ignorants I(t)and the time t when the value of T varies.The parameters are α=0.1 and f=0.05.
5 Conclusions
The implementation of immunization strategy for the networks is an important way to prevent the spread of rumors.In this paper,considering the in fluence of delay time and the spread of truth,we have constructed a novel SIR rumor spreading model to investigate the effect of random immunization strategy on homogeneous networks.We found that the existence of delay time reduces the effect of immunization strategy,but the spread of truth can decrease the propagation degree of rumors on the networks.Therefore,it is very important to detect rumor spreading on the networks timely and publish the truth by the authoritative department.Moreover,we also found that in consideration of the effect of delay time,the network nodes’identification force can still exert an influence on the propagation behaviors of rumors,which is consistent with previous research conclusions.
The results obtained in the course of our study considered the case where each immune node has the same delay time.In reality,the time that each person contacts the truth of rumors is different,i.e.,there is a difference among the delay time of each person.Thus,it is very interesting to investigate the impact of the difference among each person’s delay time on the spread of rumors.In the future,we will focus on this research.
[1]R.Bhavnani,M.G.Findley,and J.H.Kuklinski,J.Politics 71(2009)876.
[2]C.R.Sunstein and A.Vermeule,J.Political Philos.17(2009)202.
[3]M.Del.Vicario,A.Bessi,and F.Zollo,Proc.Natl.Acad.Sci.U.S.A.113(2016)554.
[4]Y.Liu,S.M.Diao,and Y.X.Zhu,Physica A 461(2016)543.
[5]M.Kosfeld,J.Math.Econ.41(2005)646.
[6]S.A.Thomas,Sociological Spectrum 27(2007)679.
[7]Z.Zhang and Z.Zhang,Physica A 388(2009)4159.
[8]D.J.Daley and D.G.Kendall,IMA J.Appl.Math.1(1965)42.
[9]A.Sudbury,Journal of Applied Probability 22(1985)443.
[10]D.H.Zanette,Phys.Rev.E 65(2002)041908.
[11]R.Pastor-Satorras and A.Vespignani,Phys.Rev.Lett.86(2001)3200.
[12]C.Castellano and R.Pastor-Satorras,Phys.Rev.Lett.105(2010)218701.
[13]R.Yang,B.H.Wang,and J.Ren,Phys.Lett.A 364(2007)189.
[14]Y.Moreno,M.Nekovee,and A.F.Pacheco,Phys.Rev.E 69(2004)066130.
[15]M.Nekovee,Y.Moreno,and G.Bianconi,Physica A 374(2007)457.
[16]Y.Moreno,M.Nekovee,and A.Vespignani,Phys.Rev.E 69(2004)055101.
[17]D.Li and J.Ma,Physica A 469(2017)284.
[18]G.Cs′anyi and B.Szendroi,Phys.Rev.E 69(2004)036131.
[19]H.Ebel,L.I.Mielsch,and S.Bornholdt,Phys.Rev.E 66(2002)035103.
[20]L.Zhao,H.Cui,and X.Qiu,Physica A 392(2013)995.
[21]L.Zhao,Q.Wang,and J.Cheng,Physica A 390(2011)2619.
[22]X.Zhao and J.Wang,Discrete Dyn.Nat.Soc.2013(2013)1.
[23]S.Han,F.Zhuang,and Q.He,Physica A 394(2014)99.
[24]N.Madar,T.Kalisky,and R.Cohen,The European Physical Journal b-Condensed Matter and Complex Systems 38(2004)269.
[25]J.Huang and X.Jin,Journal of Systems Science and Complexity 24(2011)449.
[26]L.Sun,Y.Liu,and Q.A.Zeng,Int.J.Mod.Phys.C 26(2015)1550080.
[27]Z.He,Z.Cai,and J.Yu,IEEE Trans.Veh.Technol.99(2017)1.
[28]L.Zhao,J.Wang,and R.Huang,PloS One 10(2015)e0124978.
[29]D.J.Watts and S.H.Strogatz,Nature(London)393(1998)440.
 Communications in Theoretical Physics2017年12期
Communications in Theoretical Physics2017年12期
- Communications in Theoretical Physics的其它文章
- Damped Kadomtsev–Petviashvili Equation for Weakly Dissipative Solitons in Dense Relativistic Degenerate Plasmas
- In finite Conservation Laws,Continuous Symmetries and Invariant Solutions of Some Discrete Integrable Equations?
- New Exact Traveling Wave Solutions of the Unstable Nonlinear Schrodinger Equations
- General Solutions for Hydromagnetic Free Convection Flow over an In finite Plate with Newtonian Heating,Mass Diffusion and Chemical Reaction
- Anti-synchronization Between Two Coupled Networks with Unknown Parameters Using Adaptive and Pinning Controls?
- Linear Analysis of Obliquely Propagating Longitudinal Waves in Partially Spin Polarized Degenerate Magnetized Plasma
