Quantum Chemical Study on the Structural Characteristics and Stability of AlSn±Clusters①
ZHAO Guo-Xiang YANG Li-Jun WANG Yan-Fang MA Wen-Jin
?
Quantum Chemical Study on the Structural Characteristics and Stability of AlS±Clusters①
ZHAO Guo-Xiang YANG Li-Jun WANG Yan-Fang MA Wen-Jin②
(,041004,)
The geometric configurations and electronic structures of AlS±(= 1~10) clusters were studied by the B3LYP (DFT) method at the 6-311G** level. The changing rules of the ground state structure features, charge transfer and bonding characteristics of the aluminum-sulfur doped clusters were discussed in detail. The ground states of AlS±(> 2) are all AlcoreSshellplanar or solid double ring structures formed by inserting one Al atom to the Sand S-m(<) rings at the same time. Their molecular orbitals are mainly composed of Al- and-states mixed with S-states. Finally, the stabilities of AlS±clusters have been obtained by analyzing the energy of the ground state structures.
DFT, AlS±cluster, ground state structure, stability
1 INTRODUCTION
In recent years, the relevant theories and expe- riments about the metal-nonmetal binary clusters have captured great interest with the development of researches on binary clusters[1]. Since doping metal atoms into nonmetal clusters can greatly chan- ge their electronic structures and physicochemical properties[2-10], people would like to reveal the formation mechanism and stability rules of these kinds of binary clusters in theory. Sulfur owns many isomers[11-17], and sulfur clusters doped with metal aluminum have important application prospects in molecular catalysis, chemical nitrogen fixation, superconducting materials and so on[18], so the theoretical and experimental researches on sulfur binary clusters have drawn wide attention.
Guichemerre[19]studied the electronic states of AlS and AlS±by using the MRCI method, and obtained the spectral parameters of electronic states. Liu[20]got the time of flight mass spectrum of AlS–through the time of flight mass spectrometry measurement. Nakajima[21-23]obtained the ionization energy, affinity energy and the relevant photoelectron spectrum of AlS–clusters by using themethod and the photoelectron spec- troscopy measurements. Zhang[24]studied the geometric configurations and the vertical and adiabatic ionization energies of AlS–clustersusing the B3LYP method as well as mass spectrometry and X-ray photoelectron spectroscopy experiments. Jensen[25, 26]studied the geometric configura- tions and oscillation frequency of Al4S6and Al8S12clustersby using HF and B3LYP methods, respec- tively. Zhang[27]got the geometric configura- tions, the time of flight mass spectrum, the enthalpy and ionization energy of (Al2S3)clustersvia themethod and made the experiments of pho-toelectron spectroscopy. Ault[28]studied the geometric configurations and oscillation frequency of Al2S3using the LDF method, also obtaining the powder of Al2S3through the reaction of (CH3)3Al and H2S at high temperature. Zhong[29]studied the geometric configurations of AlSclus- ters and the electronic properties of oxide by using the B3PW91 method.Li[30]studied the geo- metric configurations of AlSclusters by using the B3LYP method, and compared the structure with other sulfur clusters.
In this article, the ground state structures and stability rules are prospected through theory study on the AlS±(= 1~10) clusters. The obtained results can provide some useful references for understanding the formation mechanism and the physical and chemical properties of AlS±clusters, also for the theoretical and experimental studies of finding larger clusters.
2 CALCULATION METHODS
The optimization process is carried out with the following three steps: firstly, the possible struc- tures considering all the designed configurations with different spin multiplicity were optimized at the B3LYP/3-21G[31, 32]level, and the optimization pro- cess does not consider the symmetry in order to verify the possibility of all calculated structures; secondly, the split basis sets with polarization and diffuse functions were adopted in the stable struc- tures which are obtained in the first step, and weuse the point group adjustment on the possible symmetry, re-optimize the structures at the B3LYP/6-31G* level and decide the stable structures with lower energy; in the end, the obtained structures with lower energy were optimized by using the B3LYP/6-311G** method and proceeded with the calculation of frequency, finally affording the ground state structures of AlS±(= 1~10) clusters. All the calculations were carried out with the Gaussian 03 program[33].
3 RESULTS AND DISCUSSION
3. 1 Geometricconfigurations of the ground state structures
3. 1. 1 Selection and confirmation of the ground state structures
In studying the properties of the clusters, the ground state structures including geometric confi- gurations and electronic structures should be firstly determined. Theoretically, various AlS±cluster topology structures with different sizes should be input as possible initial configurations. Therefore, all the possible geometric configurations of AlS±(≤4) were designed by the method of exhaustion. The calculated results show similarity on the changing rules of geometric configurations and stability of AlS[29, 30]. With increasing the geometric sizes of AlS±clusters, the quantity of the initially designed possible configurations increases greatly. The initial configurations of AlS±(>5) are obtained by two methods. One refers to the reported theory results of AlSclusters, and the other is to find certain rules in the features ofgeometric configurations of AlS±with lower energy during optimizing the AlS±clusters.
Fig. 1 shows the geometric configurations and symmetries of the ground state structures of AlS±(= 1~10) clusters by using the B3LYP/6-311G**theory. It can be seen that the ground state structures of AlS±(≤2) are all linear (the order is positive and then negative ion structure in the next discussion), among which the Al-S bonds are 0.221 and 0.212 nm, 0.004 and 0.002 nm longer than Al-S in 0.217 and 0.210 nm by using the MRCI[19]method, respectively. The bond lengths of Al-S in the AlS2±structures are 0.277 and 0.207 nm, respectively. The former is 0.069 nm longer than the bond length of Al-S in AlS2(0.208 nm)[30], and the latter is 0.001 nm shorter, which reveals that losing one electron could weaken the Al-S bond strength in a large degree.
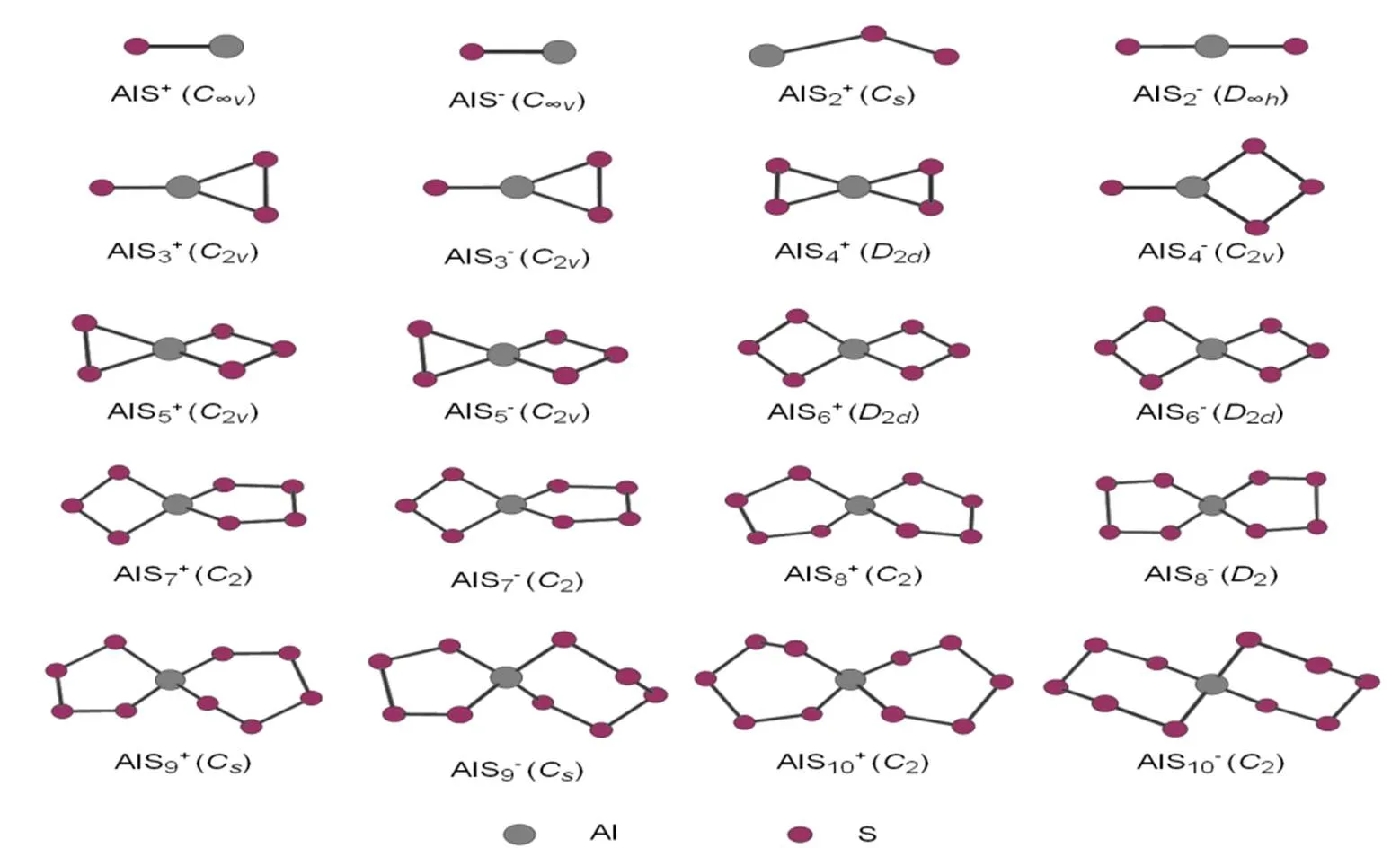
Fig. 1. Geometric configuration and symmetry of the ground state structures of AlS±clusters
The ground state structures of AlS±(> 2) clusters can be taken as planar or solid double ring structures formed by inserting one Al atom to the Sand S-m(<) rings at the same time. Most of the Al atoms are four-coordinated and most of the S atoms are two-coordinated in the structure. With increasing the cluster size, the ring structures of Sand S-min the ground state change from planar to solid staggered structure through sharing Al atoms. The ranges of the Al-S bond distances are 0.213~0.277 and 0.207~0.230 nm. The average bond distances are 0.231 and 0.222 nm, which are 0.010 and 0.001 longer than that of Al-S (0.221 nm) in a neutral structure[30], respectively. The ranges of S-S bond distances are 0.193~0.218 and 0.210~0.226 nm, with their averages to be 0.207 and 0.216 nm, respectively. Compared with the bond distance of S-S (0.211 nm) in a neutral structure, the former is 0.004 nm shorter, and the latter is 0.005 nm longer.
As seen from the changing rules of the above aluminum-sulfur binary clusters, the ground state structures of AlS±(= 1~10) clusters are linear or zigzag when≤ 2; the ground state structures of AlS±(>2) are planar or solid double ring. After analyzing the features of geometric configurations of the ground state structures, we can find that: the planar and solid double ringstructures of AlS±clusters can be taken as double-ring structure formed by inserting one Al atom to the Sand S-m(<) rings simultaneously and matching with four S atoms. The sizes of Sand S-mrings in the structure increase in an equal degree, and the structure of AlcoreSshellformed through sharing Al atoms is the most stable, while the stability declines when Al atoms appear in other places of the double-ring structure. After analyzing the symmetry of the ground state structure and the feature of chemical bonds, we can find that the symmetry of the structures of AlSand AlS-mdecreases owing to the distortion caused by Al atoms inserted to the Sand S-mrings[13]to form Al–S bonds. Most of the Al and S atoms are respectively four- and two-coordinated, and this is identical with the reported conclusion in literature[13], which suggests that most S atoms are two-coordinated except for some with 1,3-coordina- tion and the Al atoms are four-coordinated[34]in non-crystal clusters. The studies on the properties of structure and bond formation in this work present a shortcut to find and confirm the larger-sized AlS±clusters more quickly in the future.
3. 1. 2 Vibrational frequency
The vibrational frequencies of the structures in Fig. 1 can be calculated at the B3LYP/6-311G** level, and Table 1 shows the obtained values of minimum vibrational frequency () and the corres- ponding vibration frequency with maximum vibra- tional intensity (I) of the ground state structures of AlS±(= 1~10) clusters, and that in paren- thesis shows the symmetry of the vibrational mode. The minimum vibrational frequency () can reflect whether the obtained structures exist or not, while the vibrational frequency with maximum vibrational intensity (I) can reflect the location of the strongest absorption peaks in the infrared spectrum. As seen from Table 1, the minimum vibrational frequencies of the ground state structures of AlS±clusters concentrate in the ranges of 14~428 and 21~552 cm-1, and the vibrational mode is mainly bend vibration of the Al-S bond. The vibrational frequen- cies with the maximum vibrational intensity concen- trate in the 160~674 and 419~746 cm-1ranges, and the vibrational mode is mainly stretching vibration of the Al-S and S-S bonds. All the vibra- tional frequencies are positive, which means that all the optimized structures locate at stable positions on the potential energy surface.
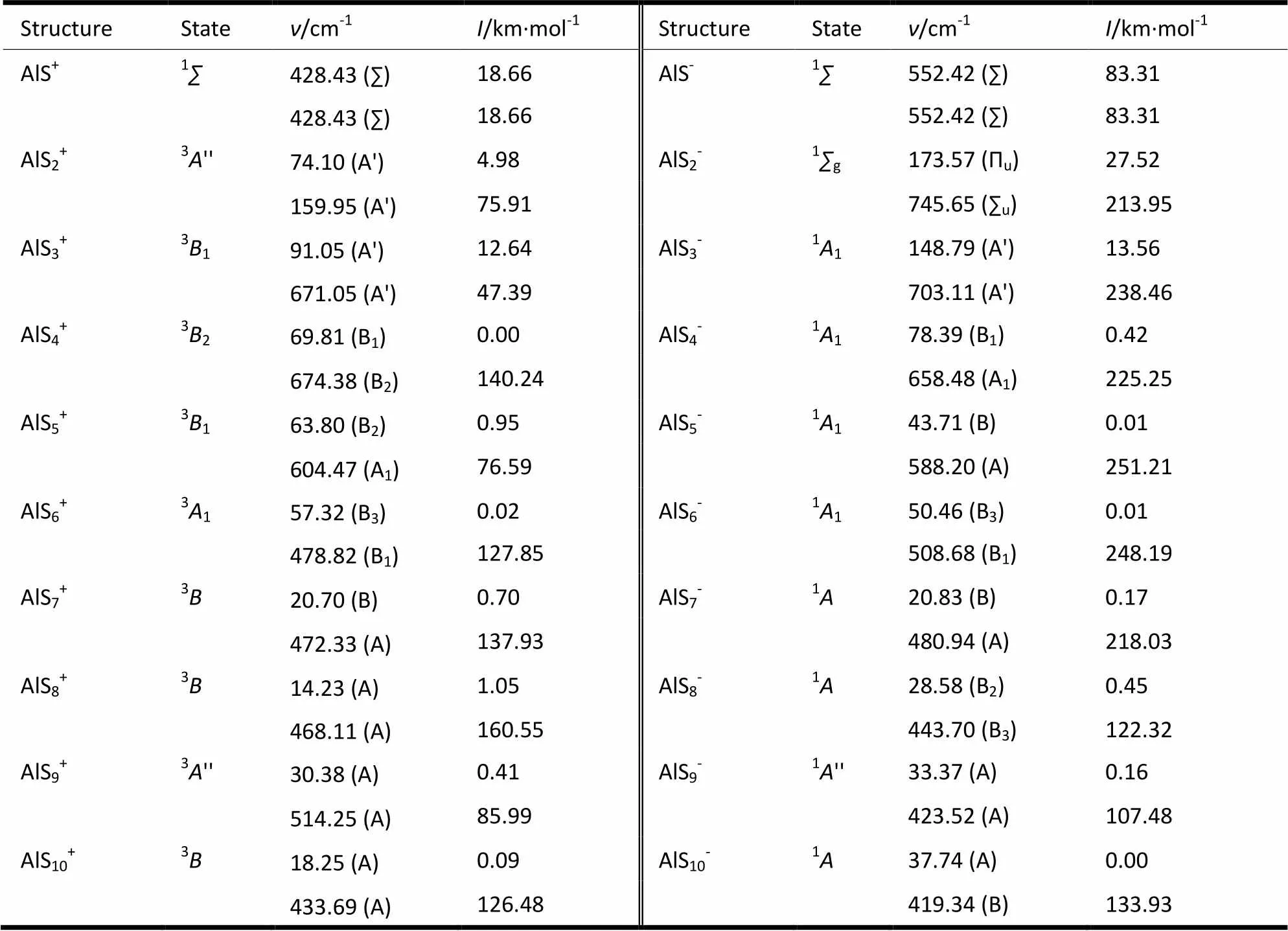
Table 1. Vibrational Frequencies of the Ground State Structures of AlSn± Clusters
: vibrational frequency;: vibrational intensity, the symmetry of the vibrational mode is in parenthesis.
3. 1. 3 Net charge distribution and bond formation of the atoms
After analyzing the net charge distribution of the atoms in NBO of the ground state structures of AlS±clusters, we can see that Al atoms locate at bridges of the Sand S-mrings, and this is beneficial to charge transfer between the Al and S atoms, and finally makes Al atoms show positive electricity, S atoms show negative electricity and Al–S bond ionize. Therefore, gaining or losing one electron would strengthen or weaken the intensity of Al–S bond, and the variation range means that the bond length is 0.009 nm.
The bond formation modes and shapes of HOMO (the highest occupied molecular orbital) and NHOMO (the next highest occupied molecular orbital) of the molecules reflect the features of the structures of the chemical bonds directly, and the changing rules of the geometric configurations and stability information of the molecules can be obtained through analyzing HOMO and NHOMO. Fig. 2 presents the molecular orbital figurations of 2D→3D structure. It can be found that a largebond is formed between Al and S atoms, and the S-Sbond in the HOMO structuresof planar AlS3±and AlS4-. It reveals that HOMO contributes to the formation of both Al-S and S-S bonds. The S chain of NHOMO also hasbond, which proves the contribution of NHOMO to the S-S bond formation. If we further investigate the electron cloud figures of the structures of AlS3±, we can find that the electron cloud density between Al–S and S–S bonds of the AlS3-structure is apparently bigger than that of AlS3+, although their configurations and point groups as well as the bond formation modes of HOMO and NHOMO are totally identical. Thebonds in the HOMO and NHOMO of AlS4+struc- tures stagger with each other, and concentrate be- tween the Al–S and S–S bonds, which means both HOMO and NHOMO contribute to the formation of ST(staggered) solid structure with2dsymmetry. The molecular orbitals of AlS±are mainly com- posed of Al- and-states mixed with S-states.-states from Al and S atoms form bigbond in the rings and S chain, and the electron delocalization effect makes the charge of different atoms well distribute, and also makes the bond lengths of Al-S and S-S become average and eventually enhan- ces the stability of the whole structure.
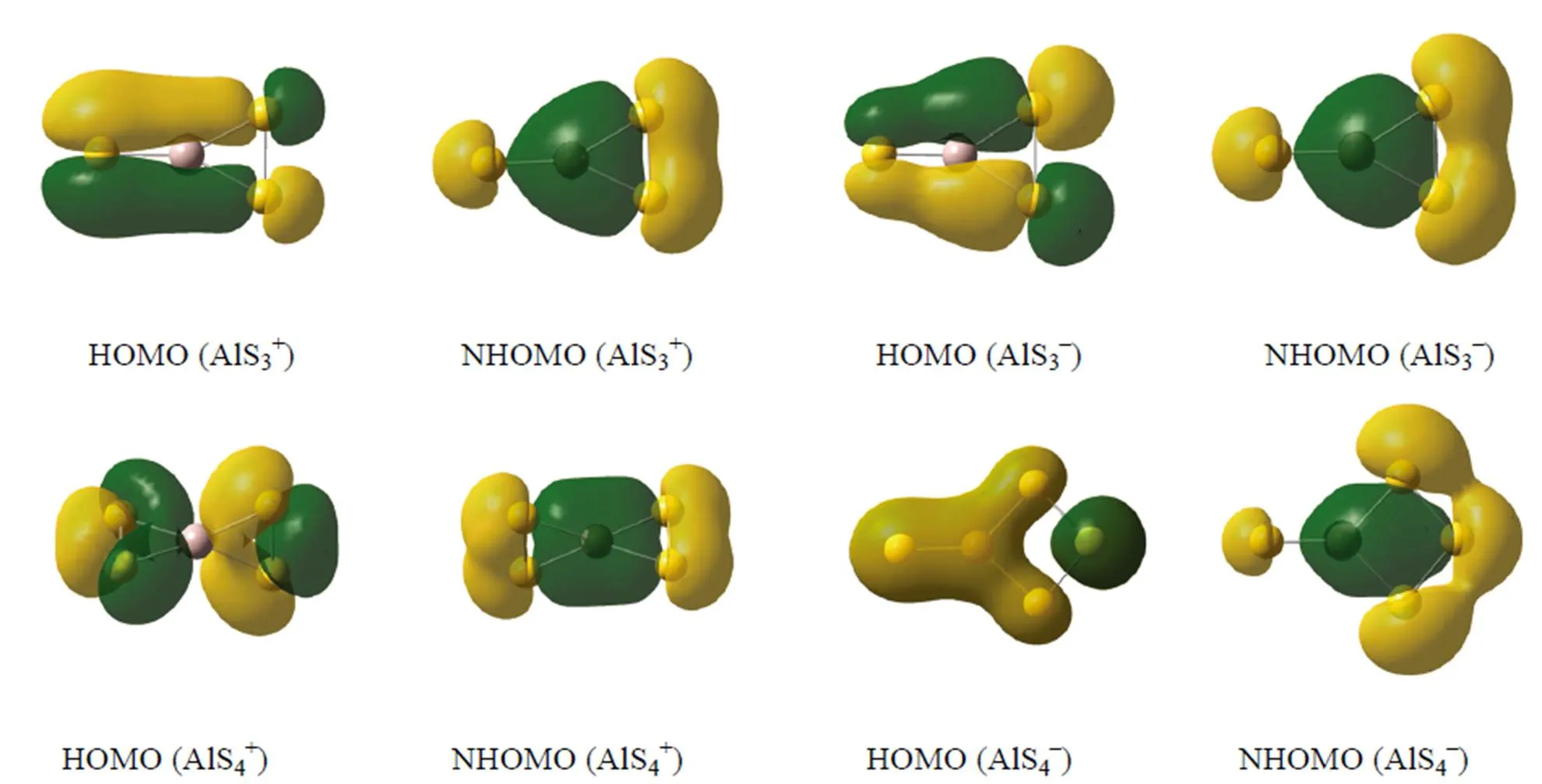
Fig. 2. HOMO and NHOMO of the ground state structures of AlS±clusters HOMO: the highest occupied molecular orbital NHOMO: the next highest occupied molecular orbital
3. 2 Stability of the ground state structure
3. 2. 1 Thermodynamical properties and energy
Table 2 presents the values of total energy (ET), zero-point energy (EZ), atom energy (ΔEn±), molar heat capacity (CP) and standard entropy (SΘ) of the ground state structures of AlS±clusters, in order to find the changing rules of the ground state structures of AlS±(= 1~10) clusters with size n. It can be concluded that: the values ofZ, ΔE±,PandΘof AlS±clusters increase with the increment of n in an equal degree. As seen from Table 2, the average increment ranges ofof AlS±clusters with positive and negative electrons are 4.53 and 4.37 kJ·mol-1, and those of ΔE±are 432.95 and 432.46 kJ·mol-1; while forPthey are 21.09 and 21.24 J·mol-1·K-1and forΘthey are 37.67 and 36.55 J·mol-1·K-1, respectively. The average increment rates of the values ofZ, ΔE±,PandΘof the ground state structures of AlS±clusters are basically identical.
3. 2. 2 Dissociation energy and second-order difference of energy
The relative stability of AlS±clusters was further investigated through analyzing the variation charac- teristics of the dissociation energy and second-order difference of energy versus the cluster sizes. Consi- dering the following chemical reactions
AlS±→ AlS-1±+ S (1)
2(AlS±) → AlS+1±+ AlS-1±(2)
the energy variation is defined as:
Δd±= (AlSn-1±+S) –AlSn±(3)
Δ2E±= (AlSn+1±+AlSn-1±) – 2AlSn±(4)
The energy variation Δd±in Eq. (3) is the disso- ciation energy of AlS±cluster. The value of Δd±can be used as a sign of the relative stability of the cluster[35]: the greater the numerical value, the better the stability of the corresponding structure. As seen from the relationship of Δd±of AlS±clusters versusin Fig. 3 as calculated according to Eq. (3), the dissociation energy (Δd±) of AlS±clusters shows an alternate oscillation downward trend with the increment of size, among which the Δd±values of AlS2-, AlS4-and AlS6-clusters are rela- tively higher. It concludes that they are more stable than other clusters; also it explains why the AlS-(≤6) clusters are easier to come into being in the experiment[20].
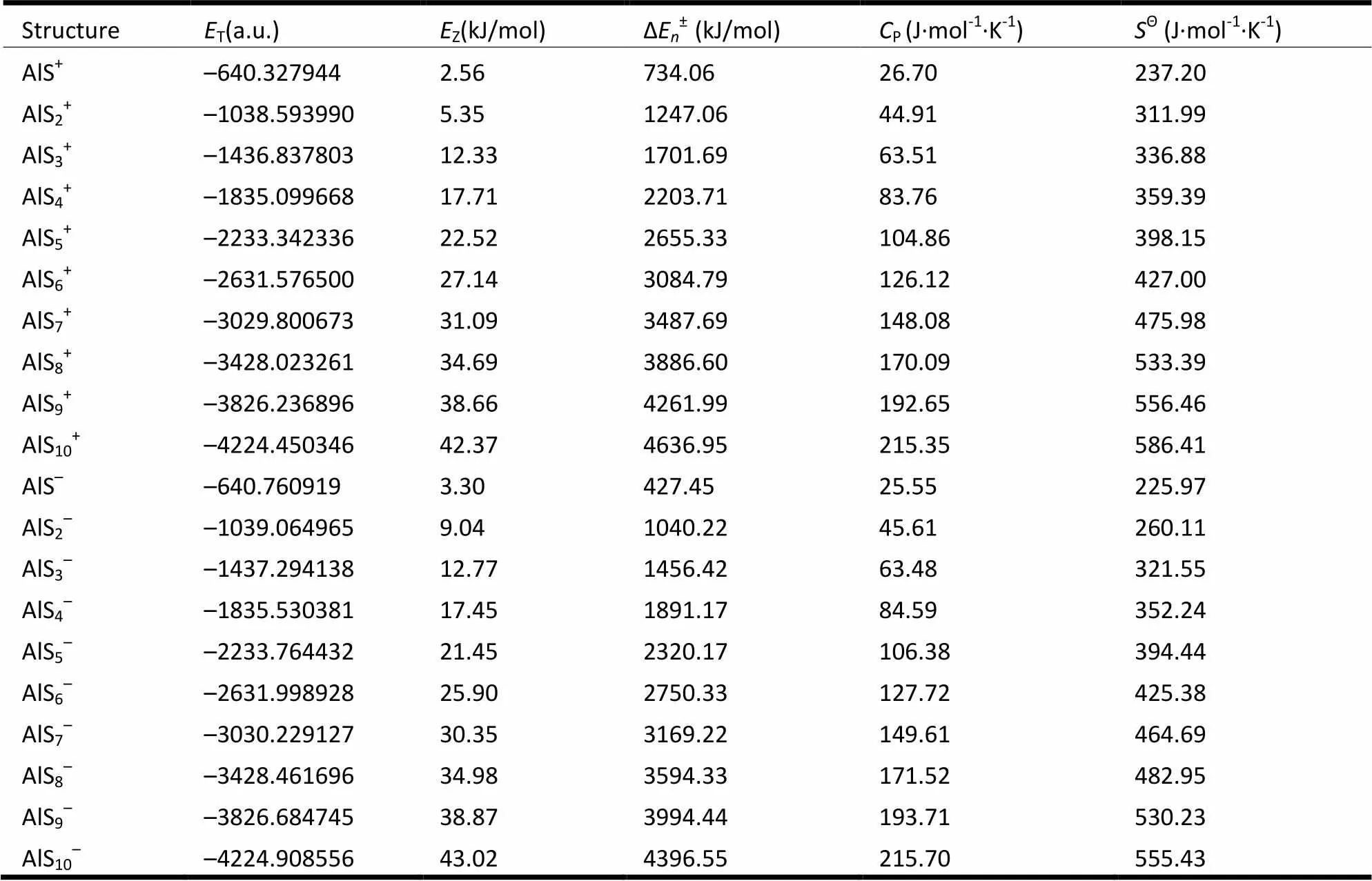
Table 2. Energy and Thermodynamic Parameters of the Ground State Structures of AlSn±Clusters
T: total energy;Z: zero-point energy; ΔE±: atom energy;P: molar heat capacity;Θ: standard entropy
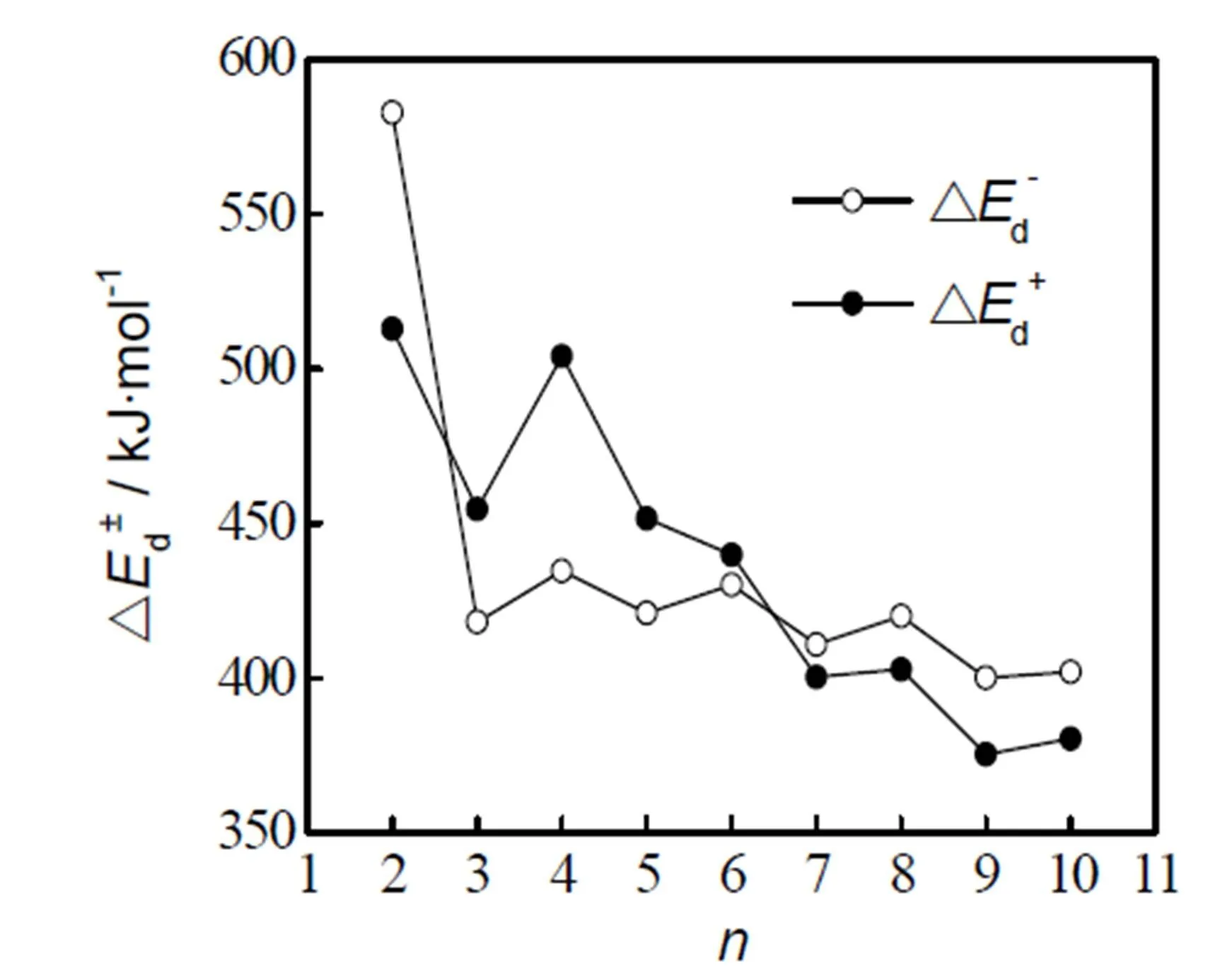
Fig. 3. Δd±of AlS±clusters versusΔd±: dissociation energy
Fig. 4. Δ2E±of AlS±clusters versusΔ2E±: the second-order difference of energy
The energy variation Δ2E±in Eq. (4) is the second-order difference of the energy for AlS±cluster. The value of Δ2E±can be used to judge the relative stability of the cluster with better sensi- tivity[36], and the bigger or smaller numerical value reflects the higher or lower relative stability of the structure. As seen from the relationship of Δ2d±of AlS±clusters versusin Fig. 4 as calculated according to Eq. (4), the values of Δ2d±decrease with the increment of n generally; the variation rule of Δ2d±is somewhatsimilar with that of Δd±.When= 3, 5, 7 and 9 as odd numbers, the values of Δ2E±are smaller or the decline is faster; and the values of Δ2E±decline more slowly or appear in peaks when= 2, 4, 6, 8 as even numbers. It con- cludes that the relative stability of the correspon- ding structures of AlS±clusters is worse when= 3, 5, 7, 9 as odd numbers; meanwhile, it is better when= 2, 4, 6 and 8 as even numbers.
4 CONCLUSION
In conclusion, the ground state structures of AlS±(= 1~10) clusters are linear or zigzag when≤2; the ground states of AlS±(>2) are AlcoreSshellplanar or solid double ring structures formed by inserting one Al atom into the Sand S-m(<) rings at the same time. The ground state structures of AlS±clusters can be found quickly when the Al atom is inserted into Sand S-m(<) rings simultaneously and matched with four S atoms to act as the matrix of designing the structures of AlS±clusters. The stability of the structures is better when Al atoms locate at the double ring bridge than at other places. The size of the ground state structure of Sand S-mcircles increases equally. Most of the Al atoms are four-coordinated and S atoms are mostly two-coordinated in the structure. The molecular orbitals are mainly composed of Al- and-states mixed with S-states. The stability of the ground state structures of AlS±(= 1~10) clusters decreases with the increment of n as a whole, and the stability of the structures of the corre- sponding clusters when n is an even number is better than that of n as an odd number.
(1) Zhai, H. J.; Ni, G. Q.; Zhou, R. F.; Wang, Y. Z. Research progress in mixed/doped clusters.1997, 17, 265–288.
(2) Zhao, Y. C.; Yuan, J. Y.; Zhang, Z. G.; Xu, H. G.; Zheng, W. J. Structures of manganese polysulfides: mass-selected photodissociation and density functional calculation.. 2011, 40, 2502–2508.
(3) Liang, B.; Wang, X.; Andrews, L. Infrared spectra and density functional theory calculations of group 8 transition metal sulfide molecules.2009,113, 5375–5384.
(4) Liang, B.; Wang, X.; Andrews, L. Infrared spectra and density functional theory calculations of group 10 transition metal sulfide molecules and complexes.2009, 113, 3336–3343.
(5) Wang, J. F.; Jia, J. F.; Ma, L. J.; Wu, H. S. Structure and stability of TiB(= 1~12) clusters: aninvestigation.2012,70,1643–1649.
(6) Guo, L. The structure and energetic of AlAs(= 1~15) clusters: a first-principles study.2010, 498, 121–129.
(7) Feng, X. J.; Luo, Y. H. Structure and stability of Al-doped boron clusters by the density-functional theory.2007, 111, 2420–2425.
(8) Li, X.; Wang, L. S.; Boldyrev, A. I.; Simons, J. Tetracoordinated planar carbon in the Al4C-anion. a combined photoelectron spectroscopy andstudy.1999,121, 6033–6038
(9) Averkiev, B. B.; Boldyrev, A. I.; Li, X.; Wang, L. S.Probing the structure and bonding in Al6N-and Al6N by photoelectron spectroscopy andcalculations.2007,111, 34–41.
(10) Averkiev, B. B.; Call, S.; Boldyrev, A. I.; Wang, L. M.; Huang, W.; Wang, L. S.Photoelectron spectroscopy andstudy of the structure and bonding of Al7N-and Al7N.2008,112, 1873–1879.
(11) Zakrzewski, V. G.; Niessen, W. V. Structures, stabilities and adiabatic ionization and electron affinity energies of small sulfur clusters S3-S5.1994, 88, 75–96.
(12) Suontamo, R. J.; Laitinen, R. S.; Pakkanen, T. A. Molecular valence calculations on small sulfur clusters S2-S5.() 1994, 313, 189–197.
(13) Chen, M. D.; Liu, M. L.; Luo, H. B.; Zhang, Q. E.; Au, C. T. Geometric structures and structural stabilities of neutral sulfur clusters.2001, 548, 133–141.
(14) Bai, Y. L.; Chen, X. R.; Yang, X. D.; Lu, P. F. Structures of small sulfures S(= 2~8) from langevin molecular dynamics methods.2003, 19, 1102–1107.
(15) Chen, M, D.; Liu, M, H.; Liu, J. W.; Jiao, Y. C.; Zhang, Q. E. Structural stabilities of small cationic sulfur clusters.2002, 15, 357–362.
(16) Chen, M. D.; Liu, M. H.; Liu, J. W.; Jiao, Y. C.; Zhang, Q. E. Structural stabilities of small cationic sulfur clusters.2002, 21, 557–561.
(17) Chen, M. D.; Liu, M. H.; Luo, H. B.; Qiu, Z. J.; Zhang, Q. E. Structural stabilities of small cationic sulfur clusters.2001,20, 399–405.
(18) Cui, M.; Feng, J. K.; Ge, M. F.; Wang, S. F.; Sun, J. Z.of lead-sulfur binary PbS-1+(= 2~4) clusters.1999, 57, 1062–1067.
(19) Guichemerre, M.; Chambaud, G. Theoretical study of the electronic states of AlS, AlS+, AlS-.2000, 104, 2105–2111.
(20) Liu, Z. Y.; Wang, C. R.; Huang, R. B.; Zheng, L. S. Mass distributions of binary aluminium cluster anions AlX-(X = O, S, P, As, C).1995, 141, 201–208.
(21) Nakajima, A.; Taguwa, T.; Nakao, K.; Hoshino, K.; Iwata, S.; Kaya, K. Photoelectron spectroscopy of AlS1–clusters (= 1~9).1995, 102, 660–665.
(22) Nakajima, A.; Zhang, N.; Kawamata, H.; Hayase, T.; Nakao, K.; Kaya, K. Photoelectron spectroscopy and mass distributions of aluminum-sulfur cluster anions (AlS-).1995, 241, 295–300.
(23) Nakajima, A.; Taguwa, T.; Nakao, K.; Hoshino, K.; Iwata, S.; Kaya, K. Photoelectron spectroscopy of binary-metal cluster anions containing sulfur atom.1996, 3, 417–421.
(24) Zhang, Z. G.; Xu, H. G.; Feng, Y.; Zheng, W. Communications: investigation of the superatomic character of Al13via its interaction with sulfur atoms.. 2010, 132, 161103.
(25) Jensen, J. O. Vibrational frequencies and structural determination of Al4S6.() 2003, 664, 37–45.
(26) Jensen, J, O. Vibrational frequencies and structural determination of Al8S12.2004, 60, 2547–2552.
(27) Zhang, N.; Shi, Y.; Gao, Z.; Kong, F.; Zhu, Q. Aluminum-sulfur cluster ions: formation and photolysis.. 1994, 101, 1219–1224.
(28) Ault, B. S. Matrix isolation study of the reaction of (CH3)3Al with hydrogen sulfide and mercaptans: synthesis and spectra of molecular Al2S3.1994, 98, 77–80.
(29) Zhong, M. M.; Kuang, X. Y.; Wang, Z. H.; Shao, P.; Ding, L. P. Probing the structural and electronic properties of aluminum-sulfur AlS(2 ≤+≤6) clusters and their oxides.. 2013,19, 263–274.
(30) Li, T. X.; Wang, L.; Wang, F.; Chen, J.; Jiang, Z. Y.; Li, L. S. Density functional theory study of neutral AlS(= 2~9) clusters.2011, 20, 033101.
(31) Becke, A. D. Density functional thermochemistry III. The role of exact exchange correlation functions.1993, 98, 5648–5652.
(32) Lee, C.; Yang, W.; Parr, R. G. Development of the Colle-Salvetti correlation energy formula into a functional of the electron density.1988, 37, 785–789.
(33) Frisch, M. J.; Trucks, G. W.; Schlegel, H. B.; Scuseria, G. E.; Robb, M. A.; Cheeseman, J. R.; Montgomery, J. A.; Vreven, T.; Kudin, K. N.; Burant, J. C.; Millam, J. M.; Iyengar, S. S.; Tomasi, J.; Barone, V.; Mennucci, B.; Cossi, M.; Scalmani, G.; Rega, N.; Petersson, G. A.; Nakatsuji, H.; Hada, M.; Ehara, M.; Toyota, K.; Fukuda, R.; Hasegawa, J.; Ishida, M.; Nakajima, T.; Honda, Y.; Kitao, O.; Nakai, H.; Klene, M.; Li, X.; Knox, J. E.; Hratchian, H. P.; Cross, J. B.; Bakken, V.; Adamo, C.; Jaramillo, J.; Gomperts, R.; Stratmann, R. E.; Yazyev, O.; Austin, A. J.; Cammi, R.; Pomelli, C.; Ochterski, J. W.; Ayala, P. Y.; Morokuma, K.; Voth, G. A.; Salvador, P.; Dannenberg, J. J.; Zakrzewski, V. G.; Dapprich, S.; Daniels, A. D.; Strain, M. C.; Farkas, O.; Malick, D. K.; Rabuck, A. D.; Raghavachari, K.; Foresman, J. B.; Ortiz, J. V.; Cui, Q.; Baboul, A. G.; Clifford, S.; Cioslowski, J.; Stefanov, B. B.; Liu, G.; Liashenko, A.; Piskorz, P.; Komaromi, I.; Martin, R. L.; Fox, D. J.; Keith, T.; Al-Laham, M. A.; Peng, C. Y.; Nanayakkara, A.; Challacombe, M.; Gill, P. M. W.; Johnson, B.; Chen, W.; Wong, M. W.; Gonzalez, C. J.; Pople, A.; Gaussian, Inc.; Wallingford CT 2004.
(34) Gutiérrez, G.; Johansson, B. Molecular dynamics study of structural properties of amorphous Al2S3.2002, 65, 104202.
(35) Bonacic, K. V.; Fantucci, P.; Koutecky, J. Quantum chemistry of small clusters of elements of groups 1a, 1b, and 11a: fundamental concepts, predictions, and interpretation of experiments.1991, 91, 1035–1042.
(36) Wang, J. F.; Wang, G. H.; Zhao, J. J. Structure electronic properties of Ge(= 2~25) clusters from density-functional theory..2001, 64, 205411.
7 January 2013;
11 March 2014
① The project was supported by the Natural Science Foundation of Shanxi Province (No. 2012011009-4)
. E-mail: ma_w_j@163.com
- 結(jié)構(gòu)化學(xué)的其它文章
- Synthesis and Crystal Structure of a New Complex [Cu(C14H9O3)2(C5H5N)2(C2H5OH)2]①
- Synthesis, Structure, and Luminescent Property of a 3-D Strontium Complex [Sr3(pda)2(Hpda)2(H2O)2]n·2nH2O①
- Syntheses, Crystal Structures and Catalytic Properties of Copper(II) and Cobalt(II) Complexes Containing 5,6-Dimethylbenzimidazole①
- Structure and Magnetic Properties of a Bipyridine-bridged One-dimensional Ni(II) Coordination Polymer①
- Tuning of NaTaO3 Band Structure through Mn2+ Ion Doping and the Enhanced Visible Light Response①
- Proton Transfer Mechanism of Alanine Induced by Zn2+: a Theoretical Study①

