Reliability Analysis of Hydraulic Transmission Oil Supply System of Power-Shift Steering Transmission with GO Methodology
YI Xiao-jian (伊梟劍), DONG Hai-ping (董海平)*, JIANG Ji-ping (姜基平), LAI Yue-hua (賴岳華), ZHANG Zhong (張 忠)
1 School of Mechatronical Engineering, Beijing Institute of Technology, Beijing 100081, China 2 China North Vehicle Research Institute, Beijing 100072, China
Reliability Analysis of Hydraulic Transmission Oil Supply System of Power-Shift Steering Transmission with GO Methodology
YI Xiao-jian (伊梟劍)1, DONG Hai-ping (董海平)1*, JIANG Ji-ping (姜基平)2, LAI Yue-hua (賴岳華)1, ZHANG Zhong (張 忠)2
1SchoolofMechatronicalEngineering,BeijingInstituteofTechnology,Beijing100081,China2ChinaNorthVehicleResearchInstitute,Beijing100072,China
GO methodology is a success-oriented method for system reliability analysis. There are components with multi-fault modes in repairable systems. It is a problem to use the existing GO method to make reliability analysis of such repairable systems. A new GO method for reliability analysis of such repairable systems with multi-fault modes was presented. Firstly, calculation equations of reliability parameters of operators which were used to describe components with multi-fault modes in reparable systems were derived based on Markov process theory. Then, this new GO method was applied in reliability analysis of a hydraulic transmission oil supply system (HTOSS) of a power-shift steering transmission at low and high speeds. Finally, Compared with fault tree analysis (FTA) and Monte Carlo simulation, the results show that this new GO method is correct and suitable for reliability analysis of repairable system with multi-fault modes.
multi-faultmodes;GOmethodology;reliabilityanalysis;hydraulictransmissionoilsupplysystem(HTOSS)ofpower-shiftsteeringtransmission
Introduction
Hydraulic oil in hydraulic control system is provided by hydraulic transmission oil supply system (HTOSS), which is a key subsystem of power-shift steering transmission in track-laying vehicle. Reliability of HTOSS directly affects reliability of power-shift steering transmission, so its reliability analysis research has been taken seriously. The components of HTOSS usually have multi-fault modes, and the reliability parameters on these fault modes can be got in practical engineering. Because of independence and two-state assumptions, traditional fault tree analysis (FTA) and failure mode and effect analysis (FMEA) are not very effective for the reliability analysis of HTOSS with multi-fault modes. GO methodology is a success-oriented method for system reliability analysis[1]. GO methodology considering multi-fault modes will bring a more exact result for reliability analysis of HTOSS. Moreover, the effect of fault modes on reliability of HTOSS attracts the attention of researchers. However, the above problem is not completely solved based on the existing GO methodology. So far, Li and Tan used GO method to make qualitative and quantitative analyses for hydraulic system of 25t crane type[2]. Taking certain hydraulic system as the subject, Dengetal. used type 14 operators to deal with the multi-state problem of system output[3]. They directly put success probability of units to conduct GO operation, and did not consider the multi-fault modes of components while making reliability analysis based on GO method. Zhengetal. used GO method to analyze the steady-state system reliability of ship anchor hydraulic system[4]. They calculated reliability parameter of structure correlation according to GO model and solved the correlation problem of standby parallel structure, but they only considered the signal fault mode of component. The components of hydraulic system usually have one to four kinds of fault modes[5]. Shenetal. proposed the formula of reliability parameters of GO operator which described the component with two fault modes[6], but the formulas of reliability parameters of GO operator with more than two fault modes were not studied.
In this paper, the formulas of steady-state reliability parameters of GO operator with multi-fault modes are deduced based on the Markov process theory. Then, GO method is applied in steady-state availability analysis and qualitative analysis for HTOSS with low and high speeds.
1 Formulas of Steady-State Reliability Parameters of GO Operator with Multi-fault Modes
Assume that distributions of mean time between failures and distributions of repair time of all components all follow exponential distributions; when the fault rate and maintenance rate of each fault mode are known, the steady-state reliability parameters can be obtained based on the Markov process theory.
All these involved random variables are mutually independent. There aren+1 states. State 0 means all fault modes do not occur. Stateimeans fault modeioccurs,i=1, 2, …,n. The derivation steps of formulas are as follows.
(1) The state transition diagram of repairable components with multi-fault modes is shown in Fig.1.

Fig.1 State transition diagram of repairable components with multi-fault modes
(2) The state transition matrixBcan be derived from the state transition diagram of repairable components with multi-fault modes.
(3) Calculate the steady-state reliability parameters of repairable components with multi-fault modes.
(4)P0is calculated by Eq. (1).
(1)
Then, the steady-state reliability parameters of repairable components with multi-fault modes are
(2)

There are not more than four kinds of fault modes in a component in hydraulic system[5], and the formulas of steady-state reliability parameters of component with one to four kinds of fault modes are respectively obtained from Eq. (2).
2 Reliability Analysis of HTOSS of Power-Shift Steering Transmission
2.1 System analysis of HTOSS of power-shift steering transmission
HTOSS consists of oil tank, pumps P1, P2, and P3, oil filters LF1, LF2, and LF3, pressure relay, by-pass valves LF2B and LF3B, one-way valves CV1 and CV2 and so on, as shown in Fig.2.
Oil is extracted by P1 from oil pan via LF1, and then oil is injected into oil tank via LF2 and case inner passage. When LF2
is obstructed and pressure between input and output becomes more than 0.5 MPa, oil will be injected into oil tank via LF2B.

Fig.2 Diagram of HTOSS
Oil is extracted by P2 from oil tank, then injected into CV2 via LF3, and then injected into hydraulic manifold block as the pressure oil provided for oil cylinder of transmission control system and torque converter clutch. P3 provides oil for P2 via CV1 to keep pressure of control oil at low speed under control of DRV. In addition, because pressure of control oil decreases a little at high speed, ingress oil of P2 can meet requirements of system. So success rule can be defined that system can provide oil to hydraulic transmission control system at low and high speeds at steering situation without considering overload protection.
2.2 Building GO model and data processing
According to analysis result of HTOSS, operator type corresponding to component is determined in Table 1. According to statistical results from engineering, fault modes and reliability parameters of operator are obtained, as shown in Table 1. Then, availability of components with one to four kinds of fault modes are respectively calculated by Eq. (2). The results of calculation are shown in Table 1.

Table 1 Operator type, serial number and reliability parameters of components in HTOSS
GO model of control signal of CV1 at low speed is shown in Fig.3, and GO model of HTOSS at low speed is shown in Fig.4. GO model of HTOSS at high speed is shown in Fig.5. Signal flow 17 is control signal of CV1, and signal flow 23 is system output.
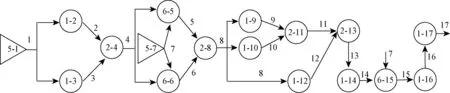
Fig.3 GO model of CV1 control signal at low speed

Fig.4 GO model of HTOSS at low speed

Fig.5 GO model of HTOSS at high speed
2.3 Quantitative analysis of HTOSS based on GO method
Based on direct probability formula algorithm[6], the success probability of CV1 control signal, HTOSS at low and high speeds can be obtained as 0.984610260, 0.998040860 and 0.999773290, respectively. Based on the modified algorithm with shared signal[7], the success probability of CV1 control signal, HTOSS at low high speeds are respectively 0.984364169, 0.986506702, and 0.986531380. Based on the exact algorithm with shared signal[8], the success probability of CV1 control signal, HTOSS at low and high speeds are respectively 0.9843284723, 0.981420933 and 0.985904145.
2.4 Qualitative analysis of HTOSS based on GO method
The results of qualitative analysis[6]of HTOSS based on GO method at low and high speeds are shown in Tables 2 and 3.

Table 2 Results of qualitative analysis of system at low speed based on GO method

Table 3 Results of qualitative analysis of system at high speed based on GO method
3 Results and Discussion
Analysis result based on above qualitative analysis can be verified by FTA[9]. When CV1 control signal at low speed is considered as a whole, we can obtain that minimum cut sets of HTOSS at low and high speeds with Go method are consistent with FTA. The sum of probability of all minimum cut sets of HTOSS at low and high speeds based on FTA are respectively 0.969036975 and 0.985892166.
The quantitative analysis results by GO method can also be verified by Monte-Carlo simulation[10]. Firstly, random numbers of success probability of operators in GO model are generated based on their availability in Table 1. Then, the simulation model is set up based on logical relationship among system and its components. At last, success probabilities of HTOSS at low and high speeds are obtained by simulation for one million times. And success probabilities of the system at low and high speeds are respectively 0.9829 and 0.9859.
Success probabilities of HTOSS of power-shift steering transmission at low and high speeds are calculated through different methods, as shown in Table 4.

Table 4 System success probability of different methods
Results in Table 4 show that as follows.
(1) Because qualitative analysis results by GO method and FTA can be thought as the lower limit of success probability of system, and results of direct algorithm, modified algorithm, and exact algorithm with shared signal by GO method are all larger than the lower limit, we can get that GO methodology is correct for reliability analysis of HTOSS at low and high speeds.
(2) The result by direct algorithm is obviously larger than results by modified algorithm and exact algorithm with shared signal. So, a large error will occur while GO operation is conducted without considering shared signal.
(3) The result by exact algorithm considering shared signal is closer to the result by Monte Carlo simulation, and it indicates that GO methodology is suitable for reliability analysis of HTOSS of at low and high speeds.
4 Conclusions
The formulas of steady-state reliability parameters of repairable component with multi-fault modes are deduced based on the Markov process theory. The reliability of HTOSS of power-shift steering transmission at low and high speeds is analyzed through direct algorithm, modified algorithm, and exact algorithm with shared signal by GO method. The result shows that a large error will occur while GO operation is conducted without considering shared signal. All minimum cut sets of HTOSS and their fault probability importance degree can be quickly got based on GO method. It can provide a theoretical basis for fault diagnosis of system. Compared with FTA and Monte Carlo, the results show that GO methodology is usable and correct. Moreover, this paper provides guidance for reliability analysis of repairable system with multi-fault modes.
[1] Gateley W Y, Williams R L. GO Methodology-Overview [R]. EPRI NP-765, Kaman Sciences Corporation, 1983.
[2] Li H Q, Tan Q. Reliability Analysis of Hydraulic System for Type Crane Based on Go Methodology [J].MachineTool&Hydraulics, 2008, 36(4): 213-215.
[3] Deng Y X, Xu F Y, Jia M P. Reliability Analysis of Hydraulic System Based on GO Methodology [J].ChineseHydraulics&Pneumatics, 2007(10): 3-6. (in Chinese)
[4] Zheng W J, Lin S F, Jiang X X,etal. A Reliability Analysis of the Repairable Hydraulic System Basing on GO Theory [J].Mechanical&ElectricalTechnology, 2009(Sl): 78-82. (in Chinese)
[5] Zhao J Y, Yao Y C. Hydraulic System Reliability Engineering [M]. Beijing: China Machine Press, 2011: 236-240. (in Chinese)
[6] Shen Z P, Huang X R. Principle and Application of GO Methodology [M]. Beijing: Tsinghua University Press, 2004: 50-100. (in Chinese)
[7] Shen Z P, Gao J. GO Methodology and Improved Quantification of System Reliability [J].JournalofTsinghuaUniversity, 1999, 39(6): 15-19.
[8] Shen Z P, GAO J, Huang X R. An Exact Algorithm Dealing with Shared Signals in the GO Methodology [J].ReliabilityEngineeringandSystemSafety, 2001, 73(2): 177-181.
[9] Zeng S K, Zhao T D, Zhang J G,etal. System Reliability Design and Analysis [M]. Beijing: University of Aeronautics and Astronautics Press, 2001. (in Chinese)
[10] Xiao G, Li T T. Monte Carlo Methods of the System Reliability Analysis [M]. Beijing: Science Press, 2003. (in Chinese)
Foundation item: Technical Basis Projects of China’s MIIT (No. 2012090003)
1672-5220(2014)06-0785-04
Received date: 2014-08-08
* Correspondence should be addressed to DONG Hai-ping, E-mail: donghaipingphd@126.com
CLC number: TB114.3 Document code: A
 Journal of Donghua University(English Edition)2014年6期
Journal of Donghua University(English Edition)2014年6期
- Journal of Donghua University(English Edition)的其它文章
- Optimization of Quality Consistency Problem of Electromechanical Component due to Manufacturing Uncertainties with a Novel Tolerance Design Method
- Effect of Starch Dodecenylsuccinylation on the Adhesion and Film Properties of Dodecenylsuccinylated Starch for Polyester Warp Sizing
- Interval Fault Tree Analysis of Excavator Variable-Frequency Speed Control System
- Combinatorial Optimization Based Analog Circuit Fault Diagnosis with Back Propagation Neural Network
- Reliability Allocation of Large Mining Excavator Electrical System Based on the Entropy Method with Failure and Maintenance Data
- Deployment Reliability Test and Assessment for Landing Gear of Chang’E-3 Probe
